2D Imaging

Many different gradient waveforms can be used to scan k-space and to obtain a desired image. The most common approach, called two-dimensional Fourier transform imaging (2D FT), is to scan through k-space along several horizontal lines covering a rectilinear grid in 2D k-space. See Figure 12.1 for a schematic of the k-space traversal. The horizontal grid lines are acquired using 128 to 256 excitations separated by a time TR, which is determined by the desired contrast, RF flip angle, and the T1 of the desired components of the image. The horizontal-line scans through k-space are offset in k_y by a variable area y-gradient pulse, which happens before data acquisition starts. These variable offsets in k_y are called phase encodes because they affect the phase of the signal rather than the frequency. Then for each k_y phase encode, signal is acquired while scanning horizontally with a constant x gradient.

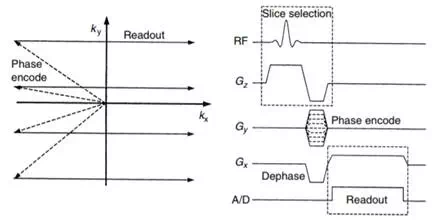
FIGURE 12.1 The drawing on the left illustrates the scanning pattern of the 2D Fourier transform imaging sequence. On the right is a plot of the gradient and RF waveforms that produce this pattern. Only four of the N_y horizontal k-space lines are shown. The phase-encode period initiates each acquisition at a different k_y and at −k_x (max). Data are collected during the horizontal traversals. After all N_y k-space lines have been acquired, a 2D FFT reconstructs the image. Usually 128 or 256 k_y lines are collected, each with 256 samples along k_x . The RF pulse and the z gradient waveform together restrict the image to a particular slice through the subject.