The Steadman Cold Comfort Studies

Because today we are more concerned about overall heat loss than frostbite -- although frostbite can still be a problem for outdoor workers and outdoor recreation -- Steadman and others looked into developing models of heat loss for long-term exposure to cold of clothed individuals. These studies looked to account for other factors such as solar heating, thermal radiation from the surroundings, clothing, physiological variables and level of activity. Clothing acts as insulation to heat loss and is accounted for using a resistance term to heat flow away from the body.

Steadman has devised an apparent temperature which accounts for these other factors through a series of complex calculations. We won't delve into the formulations here but will look at some of the implications. His research found that much of the heat loss effect of wind resulted by air movement through the clothing ensemble and the reduction of insulating air spaces within clothing by compression forces rather than by destroying the surface dead air layer. (Movement can also reduce clothing's effectiveness by about 20%.) This is why windbreakers of material highly impervious to wind penetration are so effective. It also accounts for the effectiveness of multi-layering of clothing rather than wearing only one thick layer.

Steadman also found that full sun on a standing person can warm the apparent temperature by 8 C^o under calm conditions and 4 C^o under strong winds during cold weather conditions.

Steadman's apparent temperature does not have many of the limitations of Siple's original windchill temperature equation (see below) because it stems from basic principles of the heat balance equations rather than simply fitting an empirical equation to measurements. Thus, it can be applied to higher temperatures. This allows calculations of windchill variations and corresponding clothing requirements applicable throughout the year.

In 2001, a new windchill index was introduced into North America using many of the same principles of Steadman's work. The section below looks at the original research of Paul Siple which lead to the first reported windchill temperatures and the new windchill index introduced in the Winter of 2001-2002.

WINDCHILL

The Siple Windchill Formulation

The original concern over the chilling effects of wind and temperature under extreme cold conditions was focused of frostbite on unprotected skin, particularly the face. At the time frostbite was felt to be the limiting factor in cold exposure. But the introduction of the protective face mask and other headwear later changed the focus to overall body heat loss.

The original windchill index was simply the product of air temperature (Celsius degrees below freezing) and wind speed (m/s). The term windchill was coined by Paul Siple as part of his doctoral thesis in 1939. But in the late 1930s, Paul Siple did research in the Antarctic (as part of the third Byrd expedition) on heat loss rates and freezing time of water-filled, plastic cylinders as a function of air temperature and wind speed. He believed that this experimental set-up best mimicked the heat loss from exposed human flesh. From his data, he produced an empirical formula for a heat loss rate:

h = a + bVⁿ +cV^m

where h is the heat transfer coefficient in kcal/(m² h C^o),

V is the wind speed in m/s

a, b and c are empirically derived constants

and n and m are derived exponents (usually n = 0.5 and m = 1)

From this expression, a dry heat loss expression can be derived:

Q_c = h (T_s - T)

where Q_c is the heat loss in kcal/(m² h)

T_s is the skin temperature in ^oC (usually taken as 33 ^oC)

and T is the air temperature in ^oC.

[Note that this equation is expressed similar to the basic "Ohm's Law" form (I = V/R) where the heat loss rate is the current (I), the temperature difference is the voltage potential difference (V), and h is the conductance which is the inverse of the resistance (R).]

Siple found that the heat loss from his cylinder peaked at 25 m/s (90 km/h) and then diminished. The data began at 2.25 m/s (5 mph) because this is the wind speed that removes the insulating layer of dead air from human skin. The experiment was only run for subfreezing temperatures, so strictly speaking, windchill should not be applied to conditions were temperatures are above freezing...which does not stop its application for these conditions by many.

Since Siple's original report in 1940 (and finally published in the open literature in 1945), the form of the equation has not changed but the coefficients have been recalculated by several investigators to provide a better fit to the data and to accommodate other units of measure. Siple was aided in his research by Charles Passel, so often the equation is referred to as the Siple-Passel equation.

From the heat loss, we can calculate an equivalent windchill temperature, or simply windchill temperature, by equating the heat loss equation for ambient conditions with one with V equal to 2.25 m/s (8 km/h), and then solve it for the unknown temperature.

In the 1960s, Siple's equation for windchill became a regular part of winter weather reports. In the US, only the windchill temperature is regularly reported. In Canada, the windchill temperature is reported in most areas outside the Prairies where the windchill factor (W/m²) is reported as a cooling rate. (Many believe this is the best means of reporting.)

Prior to 2001, Environment Canada used the following basic equations for calculating Wind Chill Temperature and Wind Chill Factor in metric units:

Wind Chill Temperature (ºC) = 33 - ((12.1 + 6.12 [Sqrt(V)] - 0.32V)(33 - T) / 27.8 )

where V is the wind speed in kilometers per hour, and T is the outside temperature in degrees Celsius.

Wind Chill Factor (W m^-2) = (33 - T) (12.1 + 6.12 [Sqrt(V)] - 0.32 V)

Similar equations can be defined for Imperial units: temperature in degrees Fahrenheit and wind speed in miles per hour. Doing so alters the value of the coefficents in the above equations.

The New Windchill Index

For many years, researchers have felt that, based on scientific arguments, the windchill concept needed to be altered. They argued that a beaker of water is hardly a good model for heat loss from the heat-generating human body. Thus, intensive research over the past decade or two led to a new model and windchill formula based on modern heat-transfer theory instead of Siple's experiments. The current model is based on heat loss from an exposed human face, chosen because it is the part of the body most often exposed directly to severe winter weather. The model assumes the rest of the body to be clothed appropriately for the weather conditions.

The task of developing a new windchill index was lead by researchers from Indiana's Purdue University and Canada's Defence Civil Institute of Environmental Medicine in Toronto. The new formula was tested on human subjects in a chilled wind tunnel at Canada's Defence Civil Institute of Environmental Medicine in Toronto during the Summer of 2000. In the wind tunnel, the faces of six men and six women were exposed to various combinations of temperatures and wind speed, and the rate of temperature drop of the exposed skin was measured and their assessment of the "feel" of the cold recorded.

The resulting equation for an equivalent-temperature windchill index was accepted by Environment Canada and the US National Weather Service in 2001. It is also important to note that the windchill is not a temperature in the strict sense, but a temperature-like number that quantifies the sensation of cold. Thus, in Canada, it will be reported without the degree designation. (I will use it here, however, to distinguish between the metric and imperial windchill numbers.) The former practice of issuing a windchill factor expressed in watts per square metre used in parts of Canada will be dropped with the introduction of the new index.

Specifically, the new Windchill Temperature Index:

• uses calculated wind speed at an average height of 1.5 m (5 ft), the typical height of an adult human face determined from readings at the standard anemometer height of 10m (33 ft);
• is based on a human face model;
• incorporates modern heat transfer theory to determine heat loss from the face to its surroundings, during cold and breezy/windy conditions;
• lowers the "calm" wind threshold to 4.8 km/h (3 mph), the normal walking speed of a pedestrian across an intersection;
• uses a consistent value for skin tissue resistance; and
• assumes no impact from the sun (i.e. clear night sky).

The metric formula for windchill is:

T_wc = 13.112 + 0.6215 T_a -11.37 V^0.16 + 0.3965 T_a V^0.16

where T_wc is the windchill, V is in the wind speed in kilometres per hour, and T_a is the ambient air temperature in degrees Celsius.

The equivalent formula for Fahrenheit temperatures and wind speed in mph is:

T_wc = 35.74 + 0.6215 T_a -35.75 V^0.16 + 0.4275 T_a V^0.16

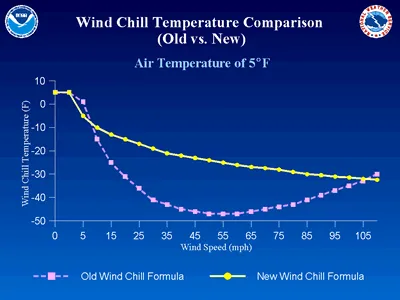
The new windchill values won't sound as scary as the old ones since the drop in windchill temperature at higher wind speeds is not as great under the new equations. For example, at minus 15^oC (5^oF) with a 50-km/h (30-mph) wind, the old formula would produce a wind chill of minus 40 (C or F), but the new formula reports the chill at minus 28 C (minus 19 F).

Under the new windchill formulation, windchills of minus 28 C (minus 19 F) and colder can cause frostbite on exposed skin in 15 minutes or less.

On October 1, 2001, Canada officially began reporting windchill using the new formulation. The Americans followed suit on November 1, using the Imperial unit version of the same formula.

Research on windchill is continuing to expand the concept for use under other potential frostbite conditions, such as including the effect of solar radiation into the windchill index and windchill under wet conditions. A "wet windchill index" could be useful, for example to mariners exposed to freezing spray conditions.