FOUR BAR MECHANISM ANALYSIS BY RELATIVE VELOCITY METHOD

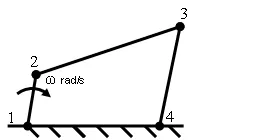
Four bar mechanism is given below in the diagram having angular velocity ω rad/s in the clockwise direction

Fig. 3.2 four bar mechanism

Velocity of the point 3 relative to 1= velocity of point 3 relative to velocity of point 2+ velocity of point 2 relative to velocity of point 1.                            

\[{{\text{V}}_{{\text{31}}}} = {{\text{V}}_{{\text{32}}}} + {{\text{V}}_{{\text{21}}}}\]                            

The velocity of point 3 relative to 1 will be equal to velocity of point 3 relative to 4 as point 1 & point 4 both are fixed point, so we can write

\[{{\text{V}}_{{\text{34}}}} = {{\text{V}}_{{\text{32}}}}{\text{ + }}{{\text{V}}_{{\text{21}}}}\]

Here

\[{{\text{V}}_{{\text{21}}}} = \omega\times {\text{12}}\], which is perpendicular to 12

Similarly,

V₃₂ is unknown in magnitude but it will be perpendicular to link 23

V₃₄ is unknown in magnitude but it will be perpendicular to link 43

Fig. 3.3

Angular velocity of links

a. Velocity of 3 relative to 2, V₃₂ link 3 moves in anti-clockwise about 2.

\[{{\text{V}}_{{\text{32}}}}{\text{ = }}{\omega _{{\text{32}}}} \times {\text{23}}\]

\[{\omega _{{\text{32}}}} = {\text{ }}\frac{{{{\text{V}}_{{\text{32}}}}}}{{{\text{23}}}}\]

b. Velocity of link 34

Angular velocity of link 3 relative to 4 is in clockwise direction & is given by

\[{\omega _{{\text{34}}}}{\text{ = }}\frac{{{{\text{V}}_{{\text{34}}}}}}{{{\text{43}}}}\]