Locating instant centres  of velocity

In the last two sections we have considered several methods of locating instant centers of velocity. They can often be located by inspecting the figure of a mechanism and visually seeking out a point that fits the definition, such as a pin-joint center. Also, after some instant centres are found, others can be found from them by using the theorem of three centers. Section 3.13 demonstrated that an instant center between a moving body and the fixed link can be found if the directions of the absolute velocities of two points of the body are known or if the absolute velocity of one point and the angular velocity of the body are known. The purpose of this section is to expand this list of techniques and to present examples.

Consider the cam-follower system of Fig. 3.25. The instant centers P12 and Pl3 can be located, by inspection, at the two pin centers. However, the remaining instant center, P23, is not as obvious. According to the Aronhold-Kennedy theorem, it must lie on the straight line connecting P12 and Pl3, but where on this line? After some reflection we see that the direction of the apparent velocity VAd3 must be along the common tangent to the two moving links at the point of contact; and, as seen by an observer on link 3, this velocity must appear as a result of the apparent rotation of body 2 about the instant center P23. Therefore, P23 must lie on the line that is perpendicular to VA2/3. This line now locates P23 as shown. The concept illustrated in this example should be remembered because it is often useful in locating the instant centers of mechanisms involving direct contact.

A special case of direct contact, as we have seen before, is rolling contact with no slip. Considering the mechanism of Fig. 3.26, we can immediately locate the instant centers P12, P23, and P34. If the contact between links 1 and 4 involves any slippage, we can only say that instant center P14 is located on the vertical line through the point of contact. However, if we also know that there is no slippage-that is, if there is rolling contact-then the instant center is located at the point of contact. This is also a general principle, as can be seen by comparing the definition of rolling contact, Eq. (3.14), and the definition of an instant center; they are equivalent.

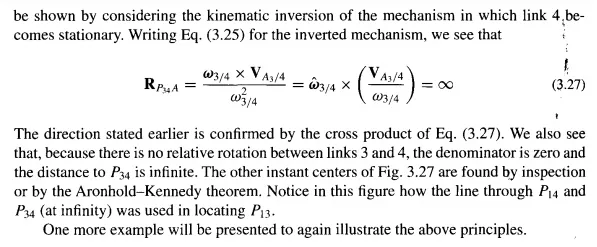
Another special case of direct contact is evident between links 3 and 4 in Fig 3.27. In this case there is an apparent (slip) velocity VA3/4 between points A of links 3 and 4, but there is no apparent rotation between the links. Here, as in Fig. 3.25, the instant center P34 lies along a common perpendicular to the known line of sliding, but now it is located infinitely far away, in the direction defined by this perpendicular line. This infinite distance can