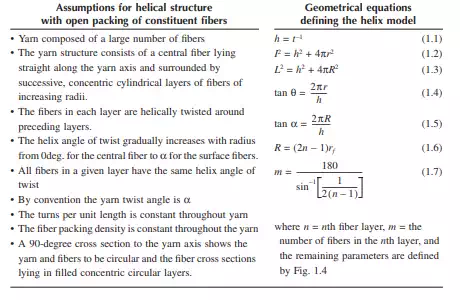
Assumptions and Geometrical Relations for Helix Yarn Model

We must consider several important limitations to the basic model.

• Many fibers do not have circular cross sections. Furthermore, when fibers of circular cross sections are inclined at a helix angle of twist, they appear elliptical in the yarn cross section 90° to the yarn axis. Thus, only the circular fiber on the yarn axis strictly meets this assumption. Nevertheless, fiber diameters are sufficiently small, and generally tend sufficiently toward circular, for the model to remain useful.

• In the yarn cross section, the concentric circular layers are filled with fibers in contact with each other. Therefore, if there are N layers comprising the yarn, then the arithmetic sum of the number, m, of fibers in each layer should equal the total amount of fibers in the yarn cross section. This, however, is not always so, and the outer layer then becomes partially filled. The result is that the yarn radius, R, is ill defined. In practice, there are many fibers in the cross sections of yarns and correspondingly many circular layers, each only the thickness of one fiber — a few microns in diameter. Thus, a partially filled outer layer may not give too great an error.

• The model does not take into account the projection of fiber ends from the yarn surface (termed yarn hairiness) or the relative positions of fiber ends within the body of a spun yarn. The projection of fiber ends from the yarn surface suggests that fiber lengths must move across layers for their ends to become hairs. Fibers at the yarn surface must have part of their lengths within the body of the yarn; otherwise, the yarn would not hold together.

The fibers of a yarn are therefore interlaced. The interlacing of fibers is called migration and is further described in Chapter 6. Migration enables the frictional contact between fibers to resist fiber ends slipping past each other. When compared with Figure 1.3, the model is clearly more appropriate for continuous filament yarns. It can be assumed, however, that, under applied axial loads, where overlapping fiber ends have sufficient frictional contact because of migration and twist, such sections of a staple yarn will approximate the behavior of a continuous filament yarn, and, where insufficient, the ends will slip past each other. Hence, by introducing the idea of slippage of overlapping fiber ends, the model can be used to interpret the effect on yarn properties of important geometrical parameters such as twist.