Sampling Theorem for Low Pass Signals

The low pass signals having the low range frequency and whenever this type of low-frequency signals need to convert to discrete then the sampling frequency should be double than these low-frequency signals to avoid the distortion in the output discrete signal. By following this condition, the sampling signal does not overlap and this sampled signal can be reconstructed to its original form.

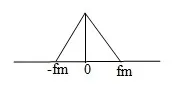
·         Bandlimited signal xa(t)

·         Fourier signal representation of xa(t) for reconstruction Xa(F)

Proof of Sampling Theorem

The sampling theorem states that the representation of an analog signal in a discrete version can be possible with the help of samples. The input signals which are participating in this process are analog signal and sample pulse train sequence.

Input analog signal is s(t) 1

The sample pulse train is

sample-pulse-train

The spectrum of an input analog signal is,

Input signal spectrum

Fourier series representation of the sample pulse train is

Fourier-series-representation-of-sample-pulse

The spectrum of the sample output signal is,

                                         spectrum-of-the-sample-output-signal

When these pulse train sequences are multiples with the analog signal we will get the sampled output signal which is indicated by here as g(t).

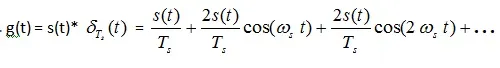
                                      sampled-output-signal

When the signal related to equation 3 passes from the LPF, only Fm to –Fm signal only passed to the output side and the remaining signal is going to be eliminated. Because LPF is assigned to the cut off frequency which is equal to the input analog signal frequency value. In this way at one side analog signal going to converted to discrete and recovered to its original position simply passing from a low pass filter.