Defining Nonlinear Load Cases In Safe To Check Uplift In Foundation

Before we get to define nonlinear load cases in SAFE, for the sake of those who don’t have any idea on the difference of nonlinear and linear analysis, let me give you an equivalent political example:

In a country ravaged by slavery, political unrest and drugs, you would expect more decadence and decay that’s deeply ingrained as a result of its sustained and cumulative damage on its victims which is the general populace.

The effect would be different if say we have peace and then invasion. And then peace again, and then the political unrest. And then peace again and the drugs infestation. If we combine the individual effects of which you won’t get the same screwed state of the people like the first one.

Now, before Zack de la Rocha comes screaming in the background let me rephrase the examples:

The last example is called the method of superposition where you combine the individual effects of several loading conditions which all started from an underformed condition. In ETABS and SAFE, if you don’t specify a nonlinear analysis, you will be defining by default a superpositioning method which is called the linear analysis.

The first example however is the nonlinear case where you add a certain loading to an already-deformed body, which has a different stiffness (taking into account the presence of cracks) compared to an underformed condition. This is a more realistic approach to analysis which is technically more correct than its linear counterpart.

After a long detour, let’s get back to our original topic.

Nonlinear analysis as defined is the accumulation of the results of a step-by-step analysis. For example, we want to check the uplift (or the presence of which) in SAFE considering the load combination 0.60 DL + 0.70 EQ which was stipulated in ASCE 7-10 (clause 2.4.1 equation 8).

First and foremost, we need to establish which loads precede what. In this case, it has to be the dead load first before applying the lateral loads. Lateral loads cannot come first before the gravity loads, right?

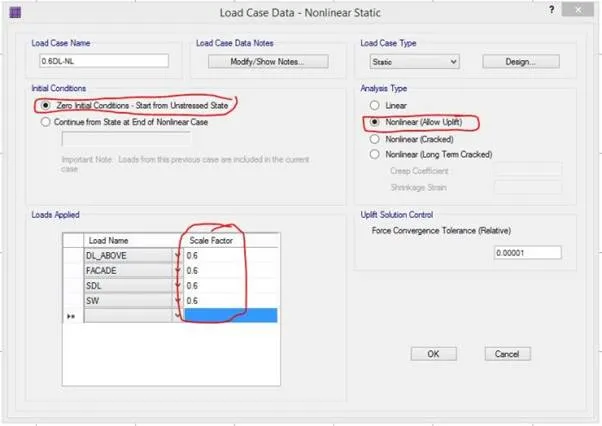
So we will define a nonlinear load case called 0.6DL-NL where NL stands for nonlinear. This is the initial condition, i.e., from an unstressed state, which we will apply the dead loads. And what comes after this condition will be the starting point of the succeeding loading condition. Just don’t forget the scale factors and to allow uplift.

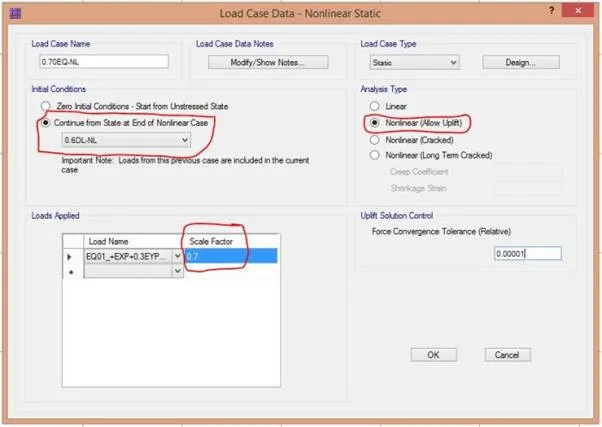
Continuing from the previous nonlinear dead load case, we define now the nonlinear seismic load case. Again, please be mindful of the scale factor and to allow uplift.

In order to confirm that the results make sense after running the analysis, the bearing contour should show zero values of the bearing pressures that were once in tension using the linear load case/load combination.

And to add to the chaos, please keep in mind the following bits and pieces of my gleaned realizations and conclusions:

A nonlinear load case involving spectral seismic loads is not possible in SAFE, hence only static load cases can be used in nonlinear analysis.

The load combination with the highest tensile bearing pressure in linear analysis does not ALWAYS produce the maximum equivalent compressive bearing stresses on the other end of the footing. Hence, we cannot immediately conclude that that nonlinear load case will always produce the greatest compressive stresses on the footings.

Having said that, we must trace one by one, out of the 100+ or so seismic and wind load combinations which produces uplift. And again, it is another matter of finding the maximum bearing pressure and finding the maximum ultimate load combination for design.

It is by far the best solution, if possible to totally eliminate if not significantly reduce to a negligible amount the uplift in order not to go to this troublesome nonlinear analysis.