Computation of Deflections

Introduction

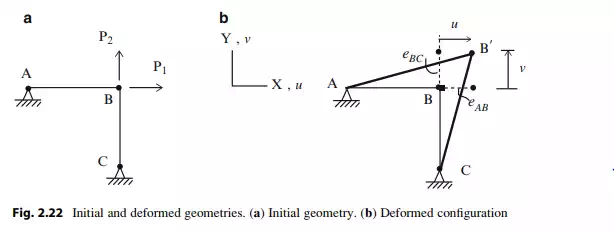
The deflections of the joints are due to the change in length of the members that make up the truss. Each member is subjected to an axial force which produces, depending on the sense, either an extension or a contraction along the member. We call these movements “axial deformation.” The study of deflection involves two steps. Firstly, we determine the axial deformation due to the applied loading. This step involves introducing the material properties for the members. Secondly, we need to relate the deflections to the axial deformations. This step is purely geometric. In what follows, we develop procedures for determining the axial deformation due to an axial force, and the deflections resulting from a set of axial deformations. The latter procedure is carried out here using a manual computation scheme.

Force–Deformation Relationship

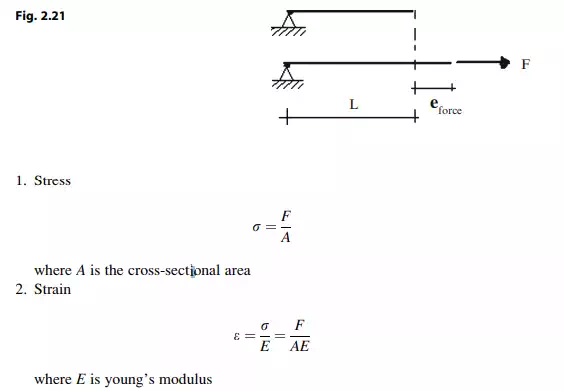
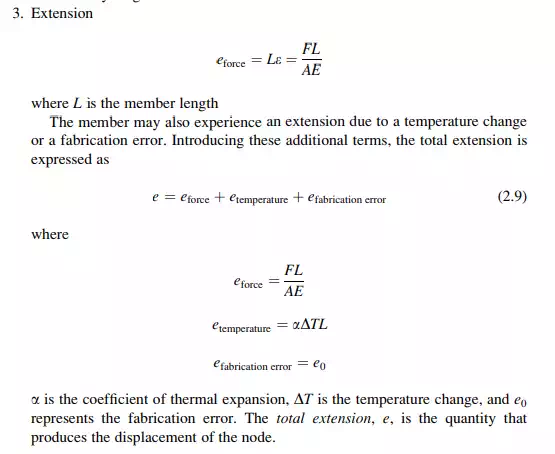
Consider the axially loaded member shown in Fig. 2.21. We suppose an axial force, F, is applied, and the member extends an amount e. Assuming the material is linear elastic, e is a linear function of F. We estimate the proportionality factor by first determining the stress, then the strain, and lastly the extension.

The steps are briefly reviewed here.