POLYMER PHASE EQUILIBRIA 

SPINODAL AND BINODAL

From the thermodynamic models for polymer blends and dissolved polymers, it is possible to calculate the thermodynamic conditions (temperature, pressure, and composition) under which phase separation occurs.

Whether two polymers are mutually miscible or a polymer is soluble in a solvent, depends on the sign of the Gibbs free energy, Δg_m, which is related to the energy and entropy of mixing:

Δg_mix = Δh_mix - T Δs_mix = Δh_mix - T ∂Δg_mix / ∂T |_p,φ < 0

However, this criterion alone is not sufficient to determine if a mixture is truly stable against phasing into two polymer mixtures of different composition. It only states that the mixture will not separate into two phases of pure components, like pure solvent and polymer. As will be shown below, phasing can occur even for negative Gibbs free energies of mixing,  Δg_mix < 0, whereas a mixture is stable if the free energy curve does not show local minima.

Δg_mix < 0  and  ∂²Δg_mix / ∂φ_p²

The dependency of Δg_mix on composition (i.e. volume fraction) at constant temperature is illustrated in the figure below. If Δg_mix is positive over the entire range of composition, as illustrated by curve I, then the two components are completely immiscible, or in other words, the solvent and the polymer form two separate phases. The other extreme, is total miscibiltiy, which is illustrated by curve III. This is the case when Δg_mix is positive and the second derivative of Δg_mix is negative over the entire range of composition:

Δg_mix < 0    and   (∂²Δg_mix / ∂φ_P²)_p,T > 0

     GIBBS FREE ENERGY OF MIXING AS A FUNCTION OF COMPOSITION

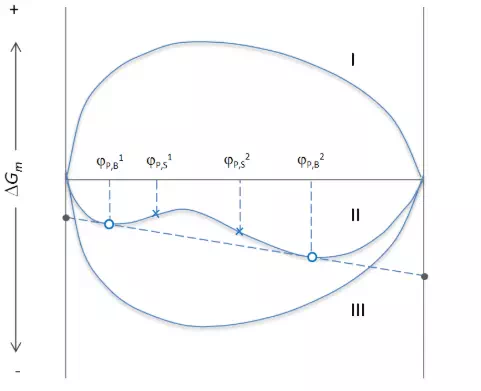
The third case describes partial miscibility. This curve has two minima at φ_P,B¹ and φ_P,B². Any mixture with a composition between these two concentrations will spontaneously phase separate into a solvent-rich and polymer-rich phase of composition φ_P,B¹ and φ_P,B² which are the points of the common tangent as illustrated by curve II. For a given polymer fraction φ_P, the volume fractions φ_P,L and φ_P,H of the two coexisting mixed phases can be calculated from

φ_P = φ_P,L φ_P,B¹ + φ_P,H φ_P,B²
           = φ_P,L φ_P,B¹ + (1 - φ_P,L) φ_P,B²

Solving this equation for φ_P,l gives

φ_P,L = (φ_P,B² - φ_P) / (φ_P,B² - φ_P,B¹)

φ_P,H = (φ_P - φ_P,B¹) / (φ_P,B² - φ_P,B¹)

The two minima φ_P,B¹ and φ_P,B² are the so called binodal points, which can be found by differentiating the Gibbs free energy of mixing, Δg_mix, with respect to the composition, φ_P. At constant pressure (p) and temperature (T), the binodal condition reads

∂Δg_mix / ∂φ_P |φ_P,B¹ = ∂Δg_mix / ∂φ_P |φ_P,B^2-

the condition for equilibrium between the two phases in a binary mixture can be also expressed by equality of the chemical potentials in the two phases:

μ_P1¹ = μ_P1²,  and μ_P2¹ = μ_P2²

μ_Pi = ∂Δg_mix / ∂n_i |_p,T, nj

where the subscripts P₁ and P₂ are the designations for the two compounds and the superscripts 1 and 2 for the two phases.

For a symmetrical polymer blend, i.e. for a blend of two polymers with equal chain length, r_P1 = r_P2 = r, the expression for the binodal reads:

χ_1,2 · r = ln[φ_P2 / φ_P1 ] / (1 - 2φ_P1)

where φ_P1 and φ_P2 are the volume fractions of the two polymers and χ_1,2 is the polymer-polymer interaction parameter.

For the general case r_P1 ≠ r_P2, no simple analytical solution exists, thus, the binodal points are usually estimated using numerical methods. This is often done with the aid of a computer. The binodal points can be also predicted by constructing the common tangent, as indicated in the figure above.

A very different situation is encountered when the composition lies between φ_P,B¹and φ_P,S¹ or between φ_P,B² and φ_P,S² (see curve II), where φ_P,S¹ and φ_P,S² are the points of inflection. We already stated that points on a concave curve, (∂²Δg_mix /∂φ_P²)_p,T > 0, are stable against phase separation, simply because on a concave curve, the total free energy of neighboring compositions is greater than that of the mixture in question, i.e., the free energy will always increase when the mixture separates into any two phases. Similarly, a mixture on a concave part of a curve with a convex portions is stable against phase separation into compositions on the concave part, that is, all points that lie between the minimum and the point of inflection of the concave portion are stable in regard to neighboring compositions. However, these compositions are not stable against separation into phases of composition φ_P,B¹ and φ_P,B². Thus, the mixture is only stable against small concentration fluctuations, but not stable against large fluctuations. This is why this region is called meta-stable. The point that separates the unstable region from the meta-stable is called a spinodal point. It is the point of inflection at constant T and p:

(∂²Δg_mix / ∂φ_P²)_p,T = 0

Another important point is the point at which the two coexisting phases coincide. This point is called the critical point. In a binary phase diagram, these are the lower (LCST) and upper critical solution temperature (UCST) of the phase curves. It is also the point where the binodal and spinodal coincide. These points are easy to calculate; if the temperature increases, the local maxima diminish and eventually vanish, thus the points of inflection and the contact points on the double tangent approach each other until they coincide. At this particular point, both the second and the third derivative of Δg_mix vanish:

(∂²Δg_mix / ∂φ_P²)_p,T = (∂³Δg_mix / ∂φ_P³)_p,T = 0

The critical value of χ where the miscibility gap begins is given by^1,2

χ_c = ½ · (r_P1^-½ + r_P2^-½)²

And the critical concentration is located at

φ_P1,c = r_P1^½ / (r_P1^½ + r_P2^½)