
STEADY STATE SOLUTION
The steady state solution of the diffusitivy equation can be derived using precisely the same mathematical steps as for the semi-steady state solution only, in this case, since ∂p/∂t = 0, the diffusivity equation is reduced to

which is the radial form of the Laplace equation. Because of the simple form of equ. (6.13) the mathematics involved in obtaining inflow equations expressed in terms of pe and p is somewhat easier than in the previous section. The derivation of these equations will therefore be left as an exercise for the reader. The solutions of the radial diffusivity equation for both steady state and semi-steady state flow conditions are summarised in table 6.1.
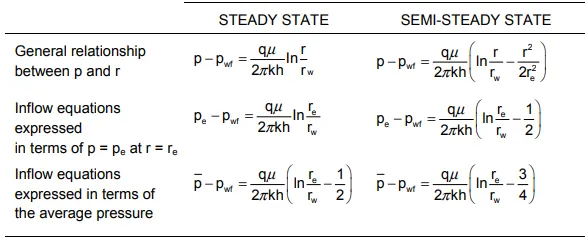
N.B. To express in field units (stb/d, psi, mD, ft.) the term q 2 kh µ π should be replaced by 141.2q Bo kh µ , in each of the equations in table 6.1 In addition the mechanical skin factor can be included in the equations as shown in equs. (4.27) and (6.7). As an alternative, the skin factor can be accounted for in the inflow equations by artificially changing the wellbore radius. For example, including the skin factor, equ. (6.12) can be expressed as

is the effective wellbore radius due to the presence of skin. If the formation is damaged, so that the permeability close to the well is reduced, the skin factor is positive. If, however, the well has been stimulated, for instance by acidising, then the permeability close to the well can exceed the average formation permeability and the skin factor is then negative.