FLUID MOVEMENT IN WATERFLOODED RESERVOIRS

Many of the principles discussed in this section also apply to immiscible gas injection, primary recovery by gravity drainage, and natural bottom-water drive. However, because of the importance of waterflooding in the United States, the emphasis is placed on fluid movement in waterflooded reservoirs. The importance of various factors that affect displacement of oil by water were discussed in the first section. In particular, the discussion on the effect of wettability on relative permeability characteristics is important in the understanding of oil displacement during waterflooding. Several textbooks on waterflooding are available [ 133,254,276-2781. The source most often referred to in this section is the excellent SPE monograph by Craig [133]; many of the principles in this monograph are summarized in the Interstate Oil Compact Commission text [277] and in an SPE paper [279]. The text by Smith [254] contains many useful aspects of waterflooding, and the recent SPE text 12781 contains a more thorough and mathematical treatment of the subject.

Displacement Mechanisms

Under ideal conditions, water would displace oil from pores in a rock in a piston-like manner or at least in a manner representing a leaky piston. However, because of various wetting conditions, relative permeabilities of water and oil are important in determining where flow of each fluid occurs, and the manner in which oil is displaced by water. In addition, the higher viscosity of crude oil in comparison to water will contribute to nonideal displacement behavior. Several concepts will be defined in order that an understanding of displacement efficiencies can be achieved.

Buckley-Leverett Frontal Advance.

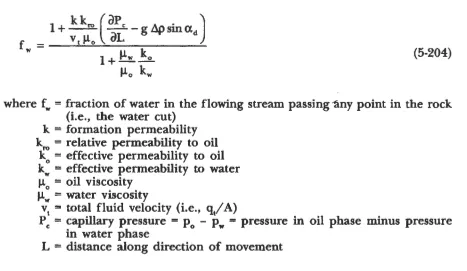
By combining the Darcy equations for the flow of oil and water with the expression for capillary pressure, Leverett [loo] provided an equation for the fractional flow of water, fw,at any point in the flow stream:

Equation 5-209 can be used to calculate the saturation distribution in a linear waterflood as a function of time. According to Equation 5-209, the distance moved by a given saturation in a given time interval is proportional to the slope of the fractional flow curve at the saturation of interest. If the slope of the fractional flow curve is graphically obtained at a number of saturations, the saturation distribution in the reservoir can be calculated as a function of time. The saturation distribution can then be used to predict oil recovery and required water injection on a time basis. A typical plot of dfw/dSw vs. S, will have a maximum as shown in Figure 5-154. However, a problem is that equal values of the slope, dfw/dSw, can occur at two different saturations which is not possible. To overcome this difficulty, Buckley and Leverett [ 1521 suggested that a portion of the saturation distribution curve is imaginary, and that the real curve contains a saturation discontinuity at the front. Since the Buckley-Leverett procedure neglects capillary pressure, the flood front in a practical situation will not exist as a discontinuity, but will exist as a stabilized zone of finite length with a large saturation gradient.