Rotational motion, vorticity field
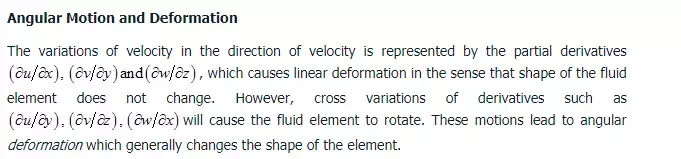
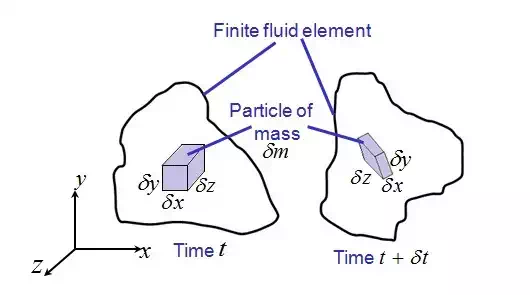
In order to illustrate this concept, we consider a typical fluid
element of certain volume at any arbitrary time as shown in Fig. 3.2.1. After
certain time interval, it has moved and changed its shape as well as
orientation drastically. However, when we limit our attention to an
infinitesimal particle of volume 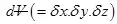 at time t and
at time t and 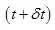 within the fluid element, it may be observed that the change of
its shape is limited to only stretching/shrinking and rotation with its sides
remaining straight even though there is a drastic change in the finite fluid
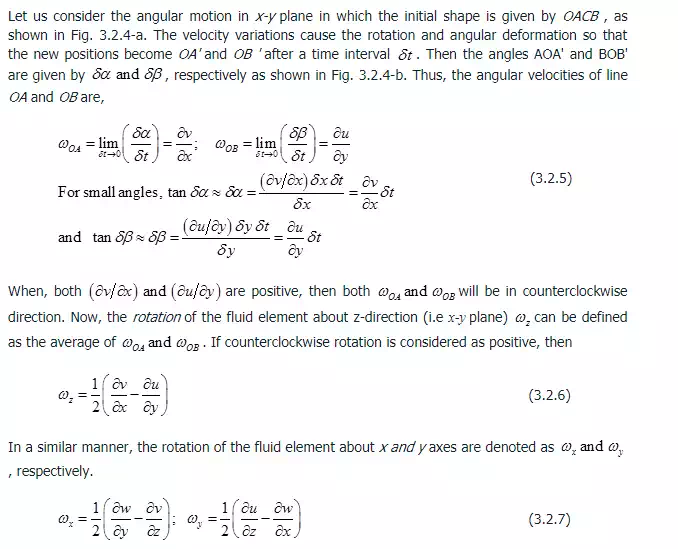
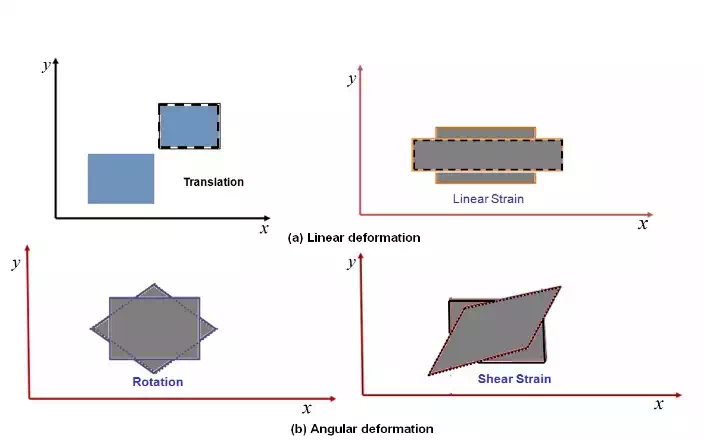
element. Thus, the particle motion in a fluid flow can be decomposed into four
fundamental components i.e. translation,
rotation, linear strain and shear strain as shown in Fig. 3.2.2. When
the fluid particle moves in space from one point to another, it is referred
as translation. Rotation of
the fluid particle can occur in any of the orthogonal axis. In the case
of linear strain , the
particle's side can stretch or shrink. When the angle between the sides of the
particle changes, it is called as shear
strain .
within the fluid element, it may be observed that the change of
its shape is limited to only stretching/shrinking and rotation with its sides
remaining straight even though there is a drastic change in the finite fluid
element. Thus, the particle motion in a fluid flow can be decomposed into four
fundamental components i.e. translation,
rotation, linear strain and shear strain as shown in Fig. 3.2.2. When
the fluid particle moves in space from one point to another, it is referred
as translation. Rotation of
the fluid particle can occur in any of the orthogonal axis. In the case
of linear strain , the
particle's side can stretch or shrink. When the angle between the sides of the
particle changes, it is called as shear
strain .
Schematic representation of motion of finite fluid element and infinitesimal particle mass at two different time steps.
Basic deformations of fluid mass: (a) Linear deformation; (b) Angular deformation.
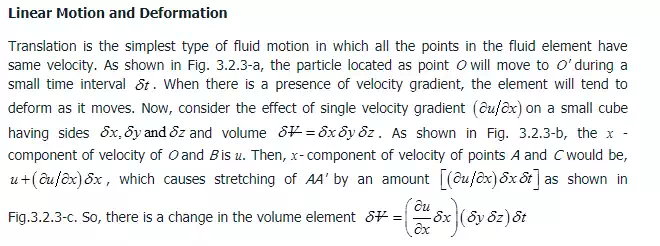
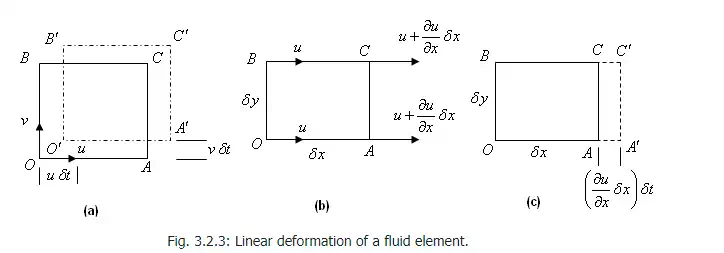
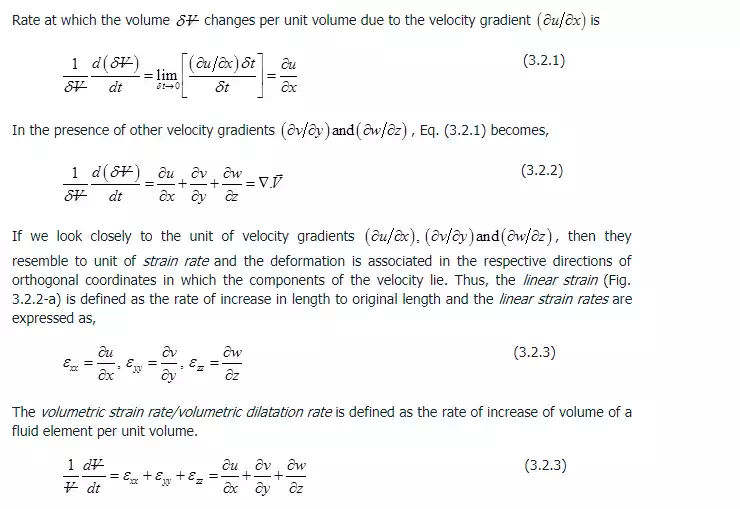
In an incompressible fluid, the volumetric dilatation rate is zero because the fluid element volume cannot change without change in fluid density.