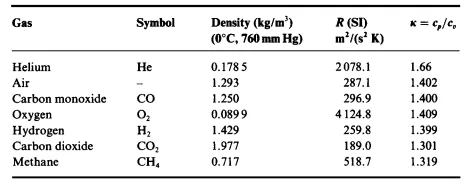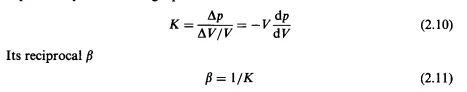
Compressibility
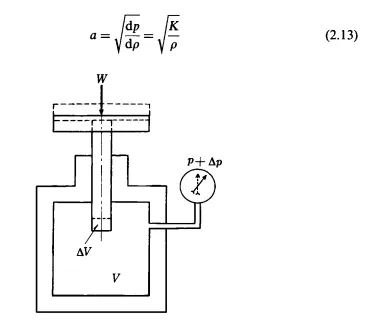
As shown in Fig. 2.9, assume that fluid of volume V at pressure p decreased its volume by AV due to the further increase in pressure by Ap. In this case, since the cubic dilatation of the fluid is AV/V, the bulk modulus K is expressed by the following equation:
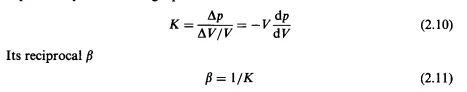
is called the compressibility, whose value directly indicates how compressible the fluid is. For water of normal temperature/pressure K = 2.06 x lo9 Pa, and for air K = 1.4 x los Pa assuming adiabatic change. In the case of water, B = 4.85 x lo-'' l/Pa, and shrinks only by approximately 0.005% even if the atmospheric pressure is increased by 1 atm. Putting p as the fluid density and M as the mass, since pV = M = constant, assume an increase in density Ap whenever the volume has decreased by AV, and

The bulk modulus K is closely related to the velocity a of a pressure wave propagating in a liquid, which is given by the following equation (see Section 13.2):