Basic Properties Of Fluids

Fluids are not strictly continuous media in the way that all the successors of Euler and Bernoulli have assumed, for they are composed of discrete molecules. The molecules, however, are so small and, except in gases at very low pressures, the number of molecules per millilitre is so enormous that they need not be viewed as individual entities. There are a few liquids, known as liquid crystals, in which the molecules are packed together in such a way as to make the properties of the medium locally anisotropic, but the vast majority of fluids (including air and water) are isotropic. In fluid mechanics, the state of an isotropic fluid may be completely described by defining its mean mass per unit volume, or density (ρ), its temperature (T), and its velocity (v) at every point in space, and just what the connection is between these macroscopic properties and the positions and velocities of individual molecules is of no direct relevance.

A word perhaps is needed about the difference between gases and liquids, though the difference is easier to perceive than to describe. In gases the molecules are sufficiently far apart to move almost independently of one another, and gases tend to expand to fill any volume available to them. In liquids the molecules are more or less in contact, and the short-range attractive forces between them make them cohere; the molecules are moving too fast to settle down into the ordered arrays that are characteristic of solids, but not so fast that they can fly apart. Thus, samples of liquid can exist as drops or as jets with free surfaces, or they can sit in beakers constrained only by gravity, in a way that samples of gas cannot. Such samples may evaporate in time, as molecules one by one pick up enough speed to escape across the free surface and are not replaced. The lifetime of liquid drops and jets, however, is normally long enough for evaporation to be ignored.

There are two sorts of stress that may exist in any solid or fluid medium, and the difference between them may be illustrated by reference to a brick held between two hands. If the holder moves his hands toward each other, he exerts pressure on the brick; if he moves one hand toward his body and the other away from it, then he exerts what is called a shear stress. A solid substance such as a brick can withstand stresses of both types, but fluids, by definition, yield to shear stresses no matter how small these stresses may be. They do so at a rate determined by the fluid’s viscosity. This property, about which more will be said later, is a measure of the friction that arises when adjacent layers of fluid slip over one another. It follows that the shear stresses are everywhere zero in a fluid at rest and in equilibrium, and from this it follows that the pressure (that is, force per unit area) acting perpendicular to all planes in the fluid is the same irrespective of their orientation (Pascal’s law). For an isotropic fluid in equilibrium there is only one value of the local pressure (p) consistent with the stated values for ρ and T. These three quantities are linked together by what is called the equation of state for the fluid.

For gases at low pressures the equation of state is simple and well known. It is

where R is the universal gas constant (8.3 joules per degree Celsius per mole) and M is the molar mass, or an average molar mass if the gas is a mixture; for air, the appropriate average is about 29 × 10−3 kilogram per mole. For other fluids knowledge of the equation of state is often incomplete. Except under very extreme conditions, however, all one needs to know is how the density changes when the pressure is changed by a small amount, and this is described by the compressibility of the fluid—either the isothermal compressibility, βT, or the adiabatic compressibility, βS, according to circumstance. When an element of fluid is compressed, the work done on it tends to heat it up. If the heat has time to drain away to the surroundings and the temperature of the fluid remains essentially unchanged throughout, then βT is the relevant quantity. If virtually none of the heat escapes, as is more commonly the case in flow problems because the thermal conductivity of most fluids is poor, then the flow is said to be adiabatic, and βS is needed instead. (The S refers to entropy, which remains constant in an adiabatic process provided that it takes place slowly enough to be treated as “reversible” in the thermodynamic sense.) For gases that obey equation (118), it is evident that p and ρ are proportional to one another in an isothermal process, and

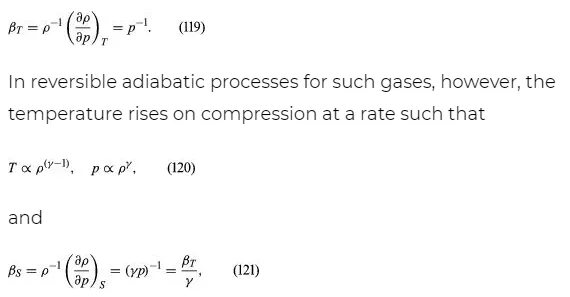
where γ is about 1.4 for air and takes similar values for other common gases. For liquids the ratio between the isothermal and adiabatic compressibilities is much closer to unity. For liquids, however, both compressibilities are normally much less than p−1, and the simplifying assumption that they are zero is often justified.

The factor γ is not only the ratio between two compressibilities; it is also the ratio between two principal specific heats. The molar specific heat is the amount of heat required to raise the temperature of one mole through one degree. This is greater if the substance is allowed to expand as it is heated, and therefore to do work, than if its volume is fixed. The principal molar specific heats, CP and CV, refer to heating at constant pressure and constant volume, respectively, and

Solids can be stretched without breaking, and liquids, though not gases, can withstand stretching, too. Thus, if the pressure is steadily reduced in a specimen of very pure water, bubbles will ultimately appear, but they may not do so until the pressure is negative and well below -107 newton per square metre; this is 100 times greater in magnitude than the (positive) pressure exerted by the Earth’s atmosphere. Water owes its high ideal strength to the fact that rupture involves breaking links of attraction between molecules on either side of the plane on which rupture occurs; work must be done to break these links. However, its strength is drastically reduced by anything that provides a nucleus at which the process known as cavitation (formation of vapour- or gas-filled cavities) can begin, and a liquid containing suspended dust particles or dissolved gases is liable to cavitate quite easily.

Work also must be done if a free liquid drop of spherical shape is to be drawn out into a long thin cylinder or deformed in any other way that increases its surface area. Here again work is needed to break intermolecular links. The surface of a liquid behaves, in fact, as if it were an elastic membrane under tension, except that the tension exerted by an elastic membrane increases when the membrane is stretched in a way that the tension exerted by a liquid surface does not. Surface tension is what causes liquids to rise up capillary tubes, what supports hanging liquid drops, what limits the formation of ripples on the surface of liquids, and so on.