Hydrostatic force on submerged surfaces

Designing of any hydraulic structure, which retains a significant amount of liquid, needs to calculate the total force caused by the retaining liquid on the surface of the structure. Other critical components of the force such as the direction and the line of action need to be addressed. In this module the resultant force acting on a submerged surface is derived.

Hydrostatic force on a plane submerged surface              

Shown in Fig.L-9.1 is a plane surface of arbitrary shape fully submerged in a uniform liquid. Since there can be no shear force in a static liquid, the hydrostatic force must act normal to the surface.

Consider an element of area  on the upper surface. The pressure force acting on the element is

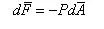
Note that the direction of  is normal to the surface area and the negative sign shows that the pressure force acts against the surface. The total hydrostatic force on the surface can be computed by integrating the infinitesimal forces over the entire surface area.

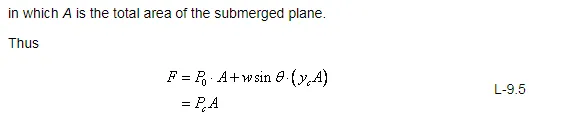
This equation says that the total hydrostatic force on a submerged plane surface equals to the pressure at the centroid of the area times the submerged area of the surface and acts normal to it.

Centre of Pressure (CP)

The point of action of total hydrostatic force on the submerged surface is called the Centre of Pressure (CP). To find the co-ordinates of CP, we know that the moment of the resultant force about any axis must be equal to the moment of distributed force about the same axis. Referring to Fig. L-9.2, we can equate the moments about the x-axis.

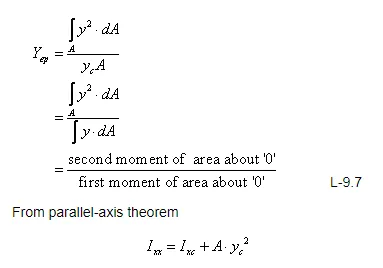
This equation indicates that the centre of the pressure is always below the centroid of the submerged plane. Similarly, the derivation of xcp can be carried out.