CLAUSIUS-CLAPEYRON-EQUATION

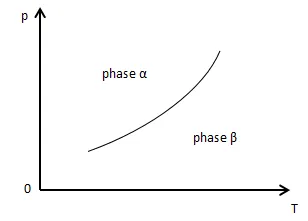
During the phase transition of pure substance, its temperature remains constant and the process is also isobaric. This phenomenon indicates that under the equilibrium of phase changing (two coexisting phases), pressure and temperature are dependent properties and have some kind of relationship with each other.

We can draw the phase transition in a p-T phase diagram as following:

The Clausius-Clapeyron equation represents the relation between pressure and temperature in a two-phase equilibrium and the slope of dp/dT of the curve as well.

We have several methods to derive this equation. And here one way will be showed.

* α and β in the following derivation stand for phase A and phase B during the phase transition

We know from the Maxwell Relation that: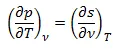

As mentioned above, pressure depends only on the temperature and has nothing to do with the specific volume during the phase-change process, therefore:

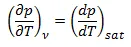here the subscript “sat” means saturation state.

Since this slope dp/dT is independent of the specific volume, it can be treated as a constant:

ν_α=constant       and       ν_β=constant

and then :(*Note that phase transition is also an isothermal process)

Hence the Maxwell Relation is now:

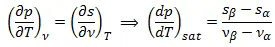
Also it is held that:

T∙ds=dh – ν∙dp

and p=constant during phase-change process (dp=0)

Then we obtain:

T∙ds=dh  →  h_β – h_α=T∙( s_β – s_α)

So the slope of dp/dT can be written now in:

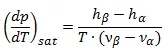
This is the well-known Clausius-Clapeyron equation which plays a crucial role in the thermodynamics if we want to solve the problems of phase transition.