LUMPED SYSTEM ANALYSIS

1)

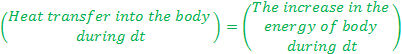
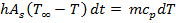
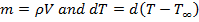
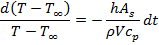
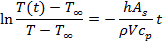
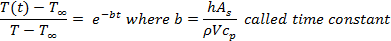
3) Criteria for Lumped System Analysis

a) Characteristic length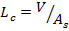 

b) Biot Number Bi

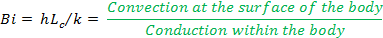
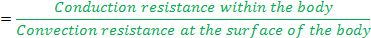
c) When a solid body is being heated by the hotter fluid surrounding it, heat is first convected to the body and subsequently conducted within the body. a small Biot number represents small resistance to heat conduction, and thus small temperature gradients within the body

d) Lumped system analysis assumes a uniform temperature distribution throughout the body, which will be the case only when the thermal resistance of the body to heat conduction (the conduction resistance) is zero. Thus, lumped system analysis is exact when Bi=0 and approximate when Bi>0. Of course, the smaller the Bi number, the more accurate the lumped system analysis. Then the question we must answer is, how much accuracy are we willing to sacrifice for the convenience of the lumped system analysis?

e) It is generally accepted that lumped system analysis is applicable if Bi ≤0.1

f) When this criterion is satisfied, the temperatures within the body relative to the surroundings (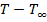) remain within 5 % of each other even for well-rounded geometries such as a spherical ball. Thus, when Bi ≤0.1, the variation of temperature with location within the body will be slight and can reasonably be approximated as being uniform.

g) Small bodies with high thermal conductivity are good candidates for lumped system analysis, especially when they are in a medium that is a poor conductor of heat (such as air or another gas) and motionless. Thus, the hot small copper ball placed in quiescent air, discussed earlier, is most likely to satisfy the criterion for lumped system analysis