Length of a Cross Belt Drive

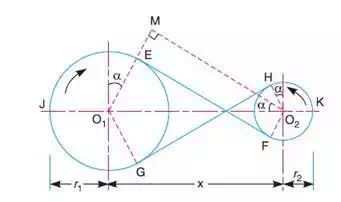
We have already discussed that in a cross belt drive, both the pulleys rotate in opposite directions as shown in Fig.

Let  r1 and r2 = Radii of the larger and smaller pulleys,

x = Distance between the centres of two pulleys (i.e. O1 O2), and L = Total length of the belt.

Let the belt leaves the larger pulley at E and G and the smaller pulley at F and H, as shown in Fig. Through O2, draw O2M parallel to FE.

From the geometry of the figure, we find that O2M will be perpendicular to O1E. Let the angle MO2 O1 = α radians

We know that the length of the belt,

L = Arc GJE + EF + Arc FKH + HG

= 2 (Arc JE + EF + Arc FK)

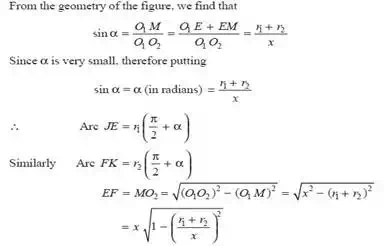
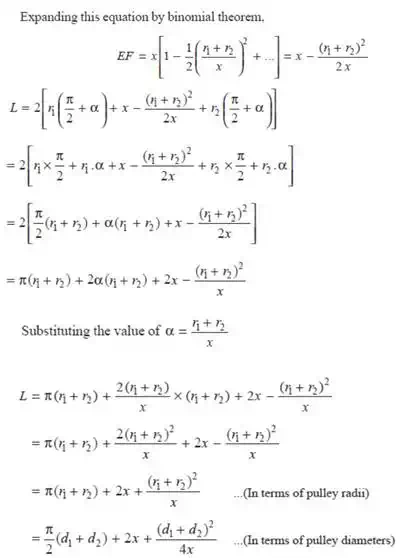
It may be noted that the above expression is a function of (r1 + r2). It is thus obvious that if sum of the radii of the two pulleys be constant, then length of the belt required will also remain constant, provided the distance between centres of the pulleys remain unchanged.