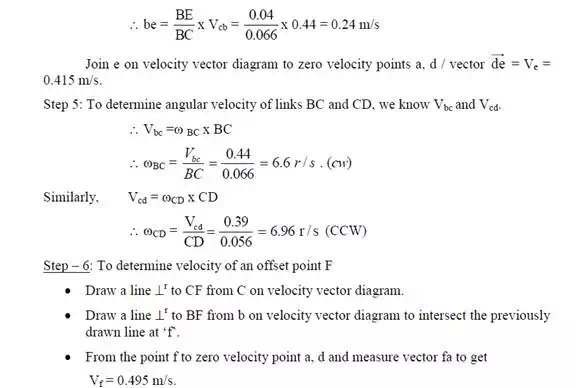
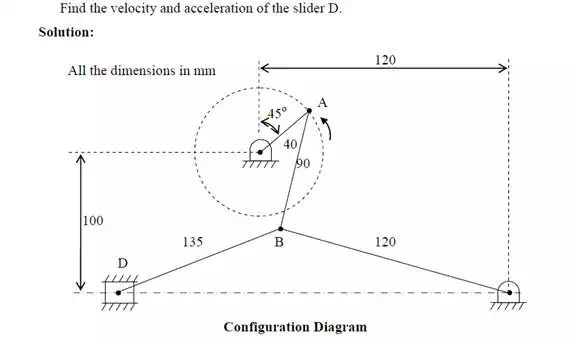
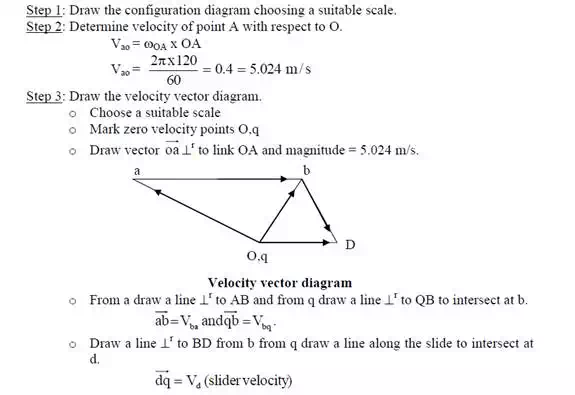
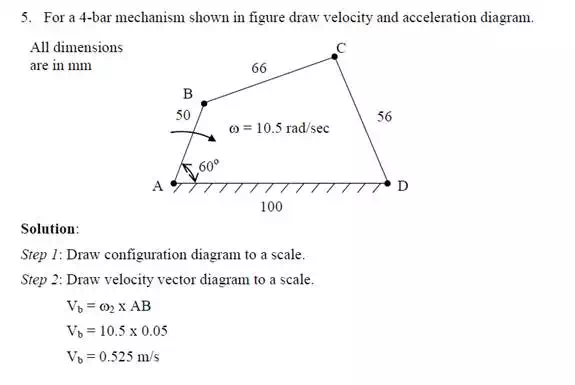
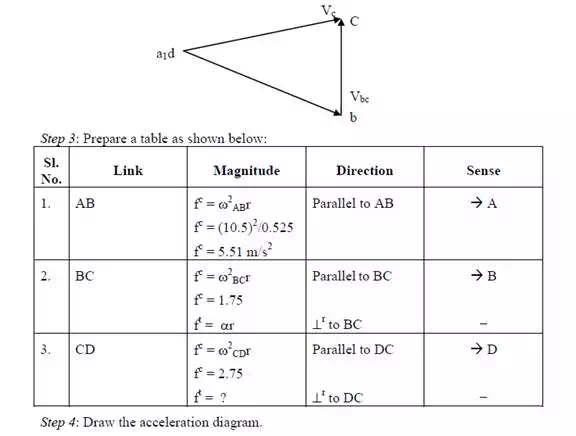
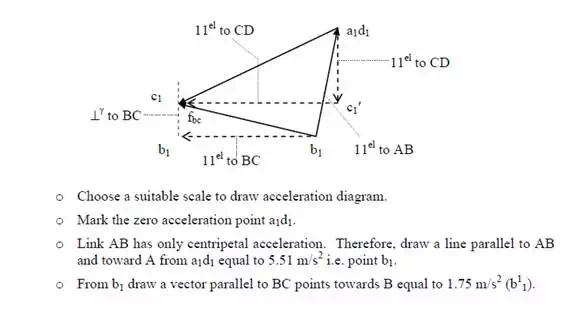
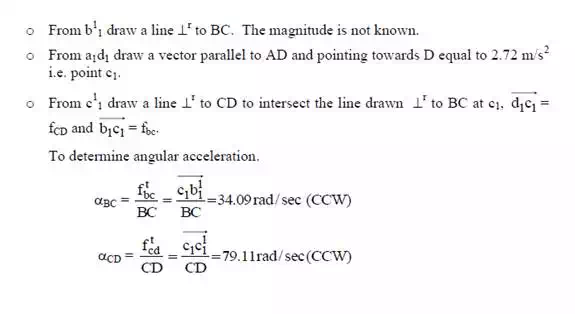
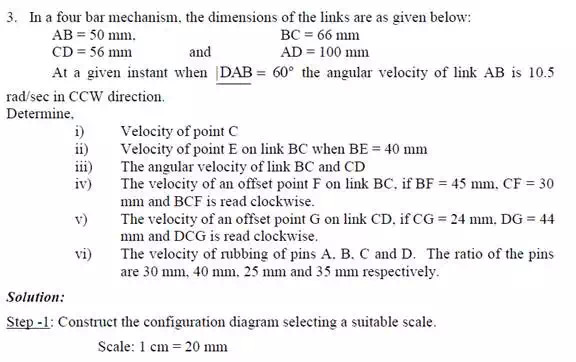
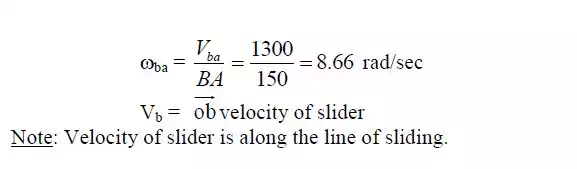Acceleration in mechanisms
We have discussed in the previous topics the velocities of various points in the mechanisms. Now we shall discuss the acceleration of points in the mechanisms. The acceleration analysis plays a very important role in the development of machines and mechanisms.
Acceleration diagram for a link
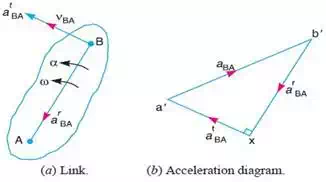
Consider two points A and B on a rigid link as shown in Fig. (a). Let the point B moves with respect to A, with an angular velocity of ω rad/s and let α rad/s2 be the angular acceleration of the link AB.
We have already discussed that acceleration of a particle whose velocity changes both in magnitude and direction at any instant has the following two components:
1. The centripetal or radial component, which is perpendicular to the velocity of the particle at the given instant.
2. The tangential component, which is parallel to the velocity of the particle at the given instant. Thus for a link AB, the velocity of point B with respect to A (i.e. vBA) is perpendicular to the link AB as shown in Fig.(a). Since the point B moves with respect to A with an angular velocity of ω rad/s, therefore centripetal or radial component of the acceleration of B with respect to A,
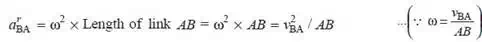
This radial component of acceleration acts perpendicular to the velocity vBA, In other words, it acts parallel to the link AB.
We know that tangential component of the acceleration of B with respect to A,
This tangential component of acceleration acts parallel to the velocity vBA. In other words, it acts perpendicular to the link AB.
In order to draw the acceleration diagram for a link AB, as shown in Fig. (b), from any point b', draw vector b'x parallel to BA to represent the radial component of acceleration of B with respect
to A i.e.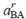 
from point x draw vector xa' perpendicular to B A to represent the tangential component of acceleration of B with respect to A i.e. 
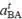. Join b' a'. The vector b' a' (known as acceleration image of the link AB) represents the total acceleration of B with respect to A (i.e. aBA) and it is the vector sum of radial component 
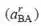and tangential component 
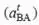of acceleration.
Exercise Problems:
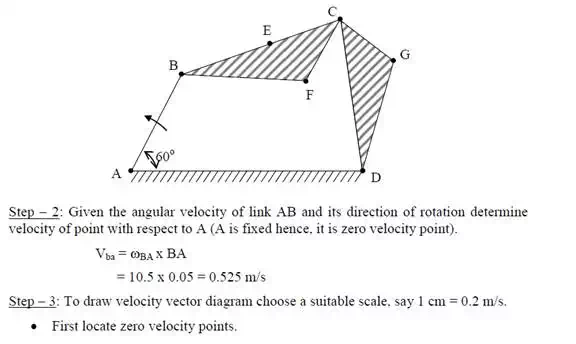
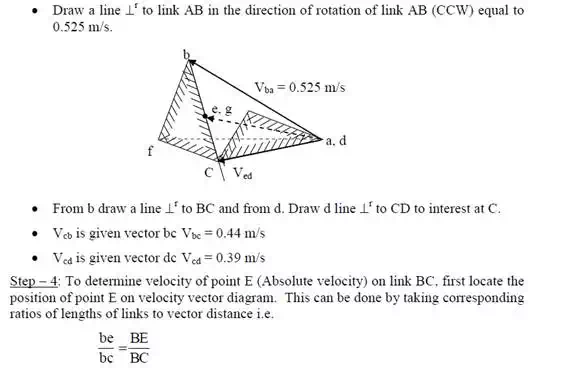
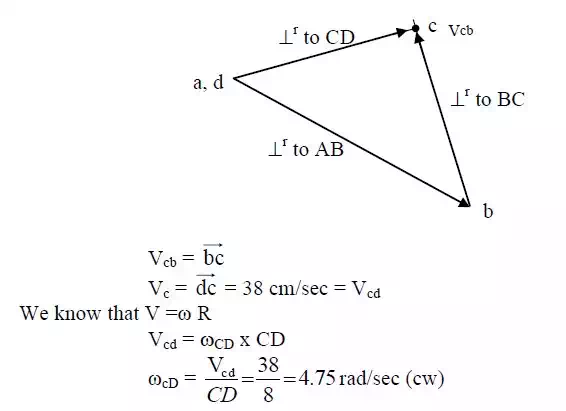
1. In a four bar chain ABCD link AD is fixed and in 15 cm long. The crank AB is 4 cm long rotates at 180 rpm (cw) while link CD rotates about D is 8 cm long BC = AD and | BAD = 60o. Find angular velocity of link CD.
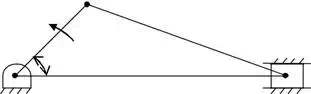
2. In a crank and slotted lever mechanism crank rotates of 300 rpm in a counter clockwise direction. Find
(i) Angular velocity of connecting rod and
(ii) Velocity of slider.
A
60 mm 150 mm
45o
B
Configuration diagram
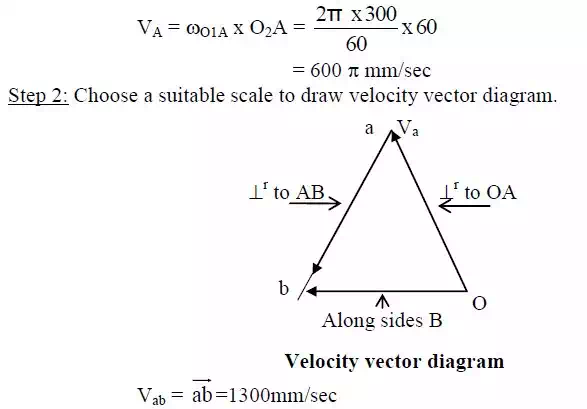
Step 1: Determine the magnitude and velocity of point A with respect to 0,
