The Frictional Force Formula

Last time we discussed the force of friction, another force in our ongoing discussion about changing forms of energy, and we learned that it’s often a counterproductive force which design engineers and engineering experts must work to minimize in order to optimize functionality of devices we’re designing.   Today we’ll introduce the frictional force formula, which computes the amount of friction present when two surfaces meet.

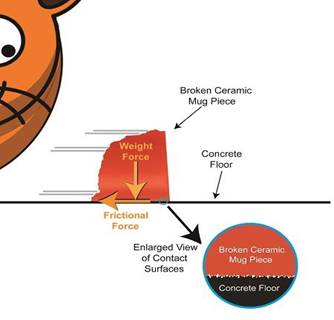
To demonstrate frictional force, we’ve been working with the example of a shattered mug’s broken ceramic pieces and watching their progress as they slide across a concrete floor.   They eventually come to a stop not too far from the point where the mug shattered, because friction causes them to stop.   The mass of the ceramic pieces in combination with the downward pull of gravity causes the broken bits to “bear down” on the floor, thereby maximizing contact and creating friction.

At first glance the floor and mugs’ surfaces may appear slippery smooth, but when viewed under magnification we see that both actually contain many peaks and valleys.   The peaks of one surface project into the valleys of the other and it’s fight, fight, fight for the ceramic pieces to continue their progress across the floor.   The strength of the frictional force acting upon the pieces is a factor of their individual weights coupled with the roughness of the two surfaces coming into contact — the shattered pieces and the floor.   If friction didn’t exist and no other impediments were in the way, the pieces might travel to the next state before stopping!

 Frictional Force Resists Motion

Last time we discussed about Charles-Augustin de Coulomb, a scientist whose work with friction led to the later development of a formula to calculate it.   It’s presented here, and frictional force is denoted as F_F,

F_F = μ × m × g

where, m is the mass of an object making contact with another surface and g is the gravitational acceleration constant, which is due to the force of Earth’s gravity.   The Greek letter μ, pronounced “mew,” represents the coefficient of friction, a number.   Numerical values for μ were determined by laboratory testing and are recorded in engineering books for many combinations of materials, including rubber on concrete, leather on steel, wood on aluminum, and our own example of ceramic on concrete.