Density and atom packing

Definition and measurement.
Density is mass per unit volume. It is measured in kg/m3. The density of a sample with a regular shape can be determined using precision mass balances and accurate measurements of the dimensions (to give the volume), but this is not the best way. Better is the “double weighing” method: the sample is weighed in air and when fully immersed in a liquid of known density. When immersed, it feels an upwards force equal to the weight of liquid it displaces (Archimedes’ principle); the density is then calculated as shown in Figure 1.
Drilling down: what determines the density?
Atoms differ greatly in weight but little in size. Among solids, the heaviest stable atom, uranium (atomic weight 238), is about 35 times heavier than the lightest, lithium (atomic weight 6.9), yet when packed to form solids their diameters are almost exactly the same (0.32 nm). The largest atom, cesium, is only 2.5 times larger than the smallest, beryllium. Thus the density is mainly determined by the atomic weight, not the atom size, and is influenced to a lesser degree by the way in which atoms are packed. Metals are dense because they are made of heavy atoms, packed densely together. Polymers have low densities because they are largely made of light carbon (atomic weight: 12) and hydrogen (atomic weight: 1) atoms in low-density amorphous or semi-crystalline packings. Ceramics, for the most part, have lower densities than metals because they contain light O, N, C, or Si atoms. Even the lightest atoms, packed in the most open way, give solids with a density of around 1000 kg/m3 - the same as that of water. Materials with lower densities than this are foams – materials made up of cells containing a large fraction of pore space. Atom packing, then, is important.
Atom packing.
The balls on a billiard table, when set, are arranged as a close-packed layer, as in Figure 2(a). The atoms of many metals pack in this way, forming layers that are far more extensive. There is no way to pack atoms more closely than this, so this particular arrangement is called “close packed”. Atomic structures are close packed not just in 2 dimensions but in 3. Surprisingly, there are two ways to do this. The depressions where three atoms meet in the first layer, layer A, allow the closest nesting for a second layer, B. A third layer can be added such that its atoms are exactly above those in the first layer, so that it, too, is in the A orientation, and the sequence repeated to give a crystal with ABABAB…stacking, as in Figure 2 (b); it is called close packed hexagonal, or cph (or sometimes hcp) for short.
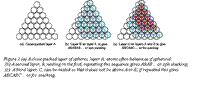
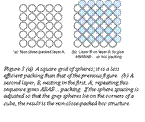
There is also an alternative. In placing the second layer, layer B, there are two choices of position. If the third layer, C, is nested onto B so that it lies in the alternative position, the stacking becomes (on repeating) ABCABCABC….as shown at (c) in the figure; it is called face-centered cubicor fcc for short. Many metals, such as copper, silver, aluminum, and nickel, have the fcc structure; many others, such as magnesium, zinc, and titanium have the cph structure. The two alternative structures have exactly the same packing fraction, 0.74, meaning that the spheres occupy 74% of the available space.
Not all structures are close packed. Figure 3 shows one of these, made by stacking square-packed layers with a lower packing density than the hexagonal layers of the fcc and hcp structures. An ABABAB…. stacking of these layers builds the body-centered cubic structure, bcc for short, with a packing fraction of 0.68. Iron and most steels have this structure. There are many other crystal structures, each with their arrangement of atoms in the single layers and stacking sequence.
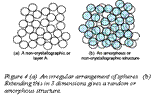
Any regular packing of atoms that repeats itself is called a crystal. It is possible to pack atoms in a non-crystallographic way to give what is called an amorphous structure, sketched in Figure 4. As you might guess, this is not an efficient way to fill space with spheres: the packing fraction at best is 0.64.
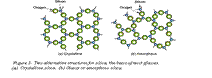
The crystalline state is the lowest energy state for elements and compounds. Melting disrupts the crystallinity, scrambling the atoms and destroying the regular order. On cooling through the melting point most metals crystallize, though by cooling them exceedingly fast it is sometimes possible to trap the molten structure to give an amorphous metallic “glass”. With compounds it is easier to do this, and with one in particular, silica – SiO2 – crystallization is so sluggish that its usual state is the amorphous one. Figure 5 shows, on the left, the atom arrangement in crystalline silica: identical hexagonal Si-O rings, regularly arranged. On the right is the more usual amorphous state. Now some rings have 7 sides, some have 6, some 5, and there is no order – the next ring could be any one of these.
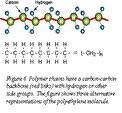
Polymer structures are quite different. The backbone of a “high” polymer (“high” means high molecular weight) is a long chain of carbon atoms, to which side groups are attached. Figure 6 shows a segment of the simplest: polyethylene, PE, (- CH2 -)n. The chains bond together to form solids. The resulting structure is sketched in Figure 7(a): a dense spaghetti-like tangle of molecules with no order or crystallinity; it is amorphous. This is the structure of thermoplastics; the weak bonds melt easily, allowing the polymer to be molded, retaining its new shape on cooling. Elastomers (rubbery polymers) have chains that have occasional cross-links to other chains, as in Figure 7(b). Thermosets like epoxies and phenolics have many cross-links, as at (c), making them stiffer and stronger than thermoplastics. The cross-links are not broken by heating, so once the links have formed thermosets cannot be thermally molded or (for that reason) recycled. All these structures are amorphous. The weak bonds of thermoplastics do, however, try to line molecules up giving ordered regions called crystallites; as shown at (d).
