Although you already have some intuition as to the behavior and properties of fluids in everyday situations, we begin this article with a seemingly simple question: From an engineering standpoint, what exactly is a fluid? Scientists categorize compositions of matter in different ways. A chemist classifies materials according to their atomic and chemical structures in the context of the periodic table of elements. An electrical engineer might group materials according to the manner in which they respond to electricity—as conductors, insulators, or semiconductors. Mechanical engineers often categorize substances as being either solids or fluids.
The technical distinction between the two centers on how they behave when forces are applied to them. We know that how the behavior of a solid material is described by a stress–strain curve. A rod that is made of an elastic solid will satisfy Hooke’s law , and its elongation will be proportional to the force acting on it. When a tension, compression, or shear force is applied to a solid object, it usually deforms by a small amount. As long as the yield stress has not been reached, a solid material will spring back to its original shape once the force has been removed.
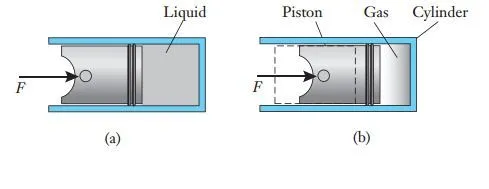
A fluid, on the other hand, is a substance that is unable to resist a shear force without continuously moving. N0 matter how small, any amount of shear stress applied to a fluid will cause it to move, and it will continue to flow until the force is removed. Fluid substances are further categorized as being either liquids or gases, and the distinction here depends on whether the fluid easily can be compressed Figure 1 . When forces are applied to a liquid, the volume does not change appreciably, even though the liquid may move and change its shape. For the purposes of most engineering applications, a liquid is an incompressible fluid. The hydraulic systems that control flight surfaces in aircraft, power off-road construction equipment, and control automotive brakes develop their large forces by transmitting pressure from the liquid hydraulic fluid to pistons and other actuators. Gases, the second category of fluids, have molecules that separate from one another widely in order to expand and fill an enclosure. A gas can be easily compressed, and, when it is compressed, its density and pressure increase accordingly.
(a) For most practical purposes in engineering, liquids are incompressible and retain their original volume when forces act on them. (b) The gas within the cylinder is compressed by the piston and force F
The primary difference between a solid and a fluid is the manner in which each behaves when subjected to a shear force. Figure depicts a thin layer of fluid that is being sheared between a stationary surface and a flat plate that is moving horizontally. The plate is separated from the surface by a small distance, and the fluid between them might be a thin layer of machine oil. When a force is applied to the upper plate, it will begin to slide over and shear the oil layer. A fluid responds to shear stress by a continuous motion that is called a flow. As an analogy, place a deck of playing cards on a tabletop, and as you press your hand against the top of the deck, also slide your hand horizontally Figure. The uppermost card moves with your hand, and the lowermost card sticks to the table. The playing cards in between are sheared, with each one slipping slightly relative to its neighbors. The oil layer in Figure behaves in a similar manner.
(a) A layer of oil is sheared between a moving plate and a stationary surface.
(b) The shearing motion of the fluid is conceptually similar to a deck of cards that is pressed and slid between one’s hand and a tabletop
A fluid layer is also sheared between two surfaces when a puck slides over an air-hockey table, an automobile tire hydroplanes over water on a road’s surface, and a person takes a plunge down a water slide. In the field of computer data storage, the read/write head in a hard disk drive (Figure) floats above the surface of the rotating disk on a thin film of air and liquid lubricant. In fact, the air layer between the read/write head and the disk is an important part of the hard disk drive’s design, and without it, rapid wear and heating of the recording head and the magnetic medium would prevent the product from functioning reliably.
Figure : The read/write heads in this computer hard disk drive slide above the surface of the rotating disk on an exceptionally thin film of air and lubricant
Experimental evidence shows that, for the majority of engineering applications, a condition called no-slip occurs at the microscopic level between a solid surface and any fluid that is in contact with it. A fluid film, which might be only several molecules thick, adheres to the solid’s surface, and the remaining fluid moves relative to it. In the case of the oil film of Figure, the no-slip condition implies that the lowermost bit of fluid will be stationary, and the uppermost element of fluid will move at the same speed as the adjacent plate. As we look across the thickness of the oil film, each layer of fluid moves at a different speed, with the velocity of the oil changing gradually across its thickness. When the upper plate in Figure slides over the fluid layer at constant speed, it is in equilibrium in the context of Newton’s second law of motion. The applied force F is balanced by the cumulative effect of the shear stress exerted by the fluid on the plate.
(a) A fluid layer is sheared between a stationary surface and a moving plate. (b) The velocity of the fluid changes across its thickness. (c) The applied force is balanced by the shear stress exerted on the plate by the fluid.
The property of a fluid that enables it to resist a shear force by developing steady motion is called viscosity. This parameter is a physical property of all gases and liquids, and it measures the stickiness, friction, or resistance of a fluid. When compared to water, honey and maple syrup, for instance, have relatively high viscosity values. All fluids have some internal friction, and experiments show that, in many cases, the magnitude of the shear stress is directly proportional to the plate’s sliding velocity. Those substances are called Newtonian fluids, and they satisfy the relation
The parameter μ (the lowercase Greek character mu, ) is called the fluid’s viscosity, and it relates the fluid’s shear stress to the plate’s speed. For Equation ( 1 ) to be dimensionally consistent, we can see that viscosity has the units of mass/(length-time).
Unit of viscosity
Viscosity values are listed in Table below for several common fluids. In both the SI and USCS, the numerical values for are generally small. Because the viscosity property arises frequently in fluids engineering, a special unit called the poise (P) was created and named in recognition of the French physician and scientist Jean Poiseuille (1797-1869), who studied the fl ow of blood through capillaries in the human body. The poise is defined as
1 P = 0.1 kg/(m.s)
Table : Density and Viscosity Values for Several Gases and Liquids at Room Temperature and Pressure
The units of kg/(m . s), slug/(ft .s), and P can each be used for viscosity. In additionto the poise, because the numerical values for μ often involve a power of -ten exponent, the smaller dimension called the centipoise (cP) is sometimes used. Centipoise is defined as 1 cP = 0.01 P. The centipoise is a relatively convenient dimension to remember, since the viscosity of freshwater at room temperature is about 1 cP.
Other Properties of fluid
Other fluid properties include the mass density, specific volume and specific weight.
· Mass density , ρ: It is denoted as mass per unit volume of the fluid.
· Specific volume, Vs : It is the reciprocal of the mass density.
· Specific weight, ɣ : It is known as weight of unit volume of fluid. ɣ = ρg