Calculate Area Moment of Inertia of Irregular Sections in Five Steps

This article will discuss the procedure to calculate area moment of inertia of different irregular cross sections.

The area moment of inertia calculation formulas are readily available for regular cross sections. But, occasionally you may come across many mechanical design problemswhere you need to find out the area moment of inertia for irregular cross sections.

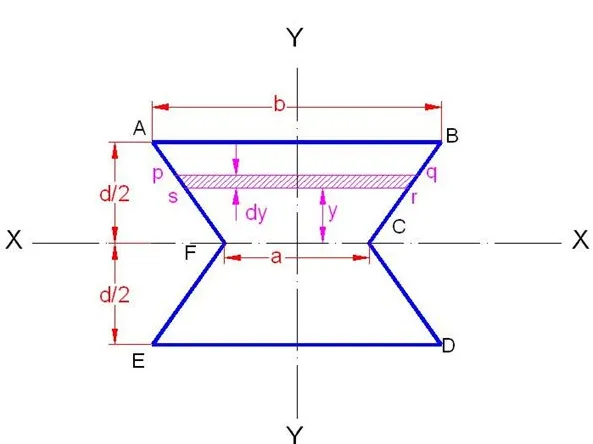
Say, you need to find the area moment of inertia of the following irregular section (ABCDEF) with respect to axis X-X. In the following five steps you will see how to proceed with such problems:

Step-1

Take a small area (pqrs) of depth dy at a distance y from the XX axis. Shown in pink color hatched area in the figure above.

Step-2

The small area pqrs can be expressed as:

Area = Width x Depth

dA = [y*{(b-a)/ (d/2)} + a]*dy……….eqn1.1

Step-3

The general equation for calculating area moment of inertia about XX axis is:

Ixx =2*[0d/2 ∫ y²dA]……………...……eqn1.2

Step-4

Using eqn1.1 & eqn1.2 we can write:

Ixx =2*[0d/2 ∫ y²[y*{(b-a)/ (d/2)} + a] dy]

= (d^3/48)*(3b-7a)

Step-5

You just derived the formula for calculating area moment of inertia for the section ABCDEF. You can obtain the area moment of inertia value by putting the value of a, band d.

Conclusion

The area moment of inertia is used in beam theory and its application. Area moment of inertia calculation formulas for the regular cross section are readily available in design data handbooks. The procedure described in this article will be useful for deriving the area moment of inertia formula for any irregular sections. Similarly, you can calculate the area moment of inertia about the axis