A Brief Discussion of Ratcheting

A review of available plasticity models in general finite element analysis (FEA) codes like ANSYS shows that there are a number of different plasticity models, including various types of hardening. Linear, multi-linear, and nonlinear hardening models are available; what is the difference and does it really matter? And, what is a Chaboche and how do you pronounce it?

For structures subjected to cyclic loading where the stress state exceeds the elastic limit of the material, low cycle fatigue response occurs, which can lead to catastrophic failure. Therefore, accurate prediction of the structural response, particularly the accumulation of plastic strain over each cycle - which is called ratcheting, is critical.

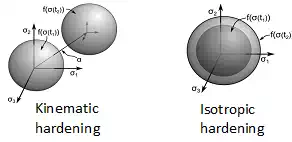
The hardening of the material during plastic deformation is the driver behind ratcheting.  Recall from basic plasticity theory that a yield surface is defined such that the stress state cannot exist outside of the yield surface.When the stress state reaches the yield surface, the surface must either translate, called kinematic hardening, or grow uniformly, called isotropic hardening, or do a combination of both.

Kinematic hardening is considered the main reason for ratcheting in metals.  Most finite element analysis codes have the ability to define bilinear, multi-linear and nonlinear kinematic hardening slopes. Ratcheting typically requires a nonlinear kinematic hardening slope for accurate modeling.

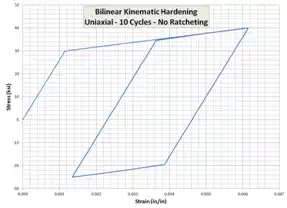
Consider the case of a uniaxial stress cycle with a non-zero mean stress. Using a bilinear kinematic hardening model for 10 cycles, no ratcheting is predicted, as shown in Figure 1 below.  If a biaxial stress cycle is used, ratcheting will be predicted, but it typically will result in a poor representation of this phenomenon. This approximation will tend to reach a stabilized state where the plastic strain accumulation is the same for each cycle, called shakedown, much sooner than is seen in experiment.

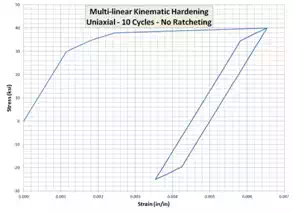
The multi-linear kinematic model is an improvement to the linear model and can predict a more realistic hysteresis loop, but the loop is closed for the uniaxial case and thus no ratcheting is produced, as shown in Figure 2.  Ratcheting will be predicted in a biaxial cycle, but poor ratcheting representation is generally found, similar to the bilinear case.

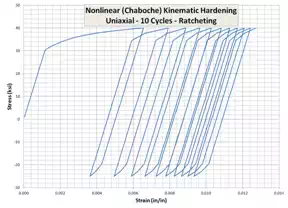
There are several nonlinear kinematic hardening models that have been developed. A common one is the Chaboche model which uses a decomposed nonlinear kinematic hardening rule which divides the hysteresis curve into segments that can describe the onset of yielding; the more constant segment at a higher strain range, and the transient nonlinear segments at the transition regions. Figure 3, below shows the uniaxial cyclic loading causing ratcheting over the 10 cycles.

Although the Chaboche model is found in many FEA codes such as ANSYS, it is not necessarily accurate for all cases and may over-predict ratcheting. Other models have been developed, including Ohno-Wang and Guionnet, that can provide a more accurate ratcheting solution.  These models are typically not available in most standard finite element codes, but they can be added with a user-defined material model.