PARABOLA and its CONSTRUCTION by ECCENTRICITY METHOD

The parabola  is a conic section, the intersection of a right circular conical surface and a plane to a generating straight line of that surface. Given a point (the focus) and a corresponding line (the directrix) on the plane, the locus of points in that plane that are equidistant from them is a parabola

OR SIMPLY

A parabola is a curve traced by a point, moving such that, at any position ,its distance from the fixed point  (focus) is always equal to its distance from a fixed straight line (directrix)

Construction

Draw the axis AB and the directrix CD, at right to each other.

Mark the focus F on the axis with given length for suppose AF=50 or 40 etc..

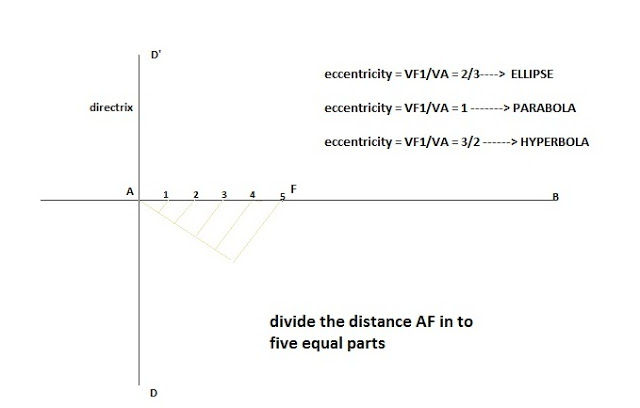
Locate the vertex V on AB such that AV=VF= ½(AF)

Draw a line VE, perpendicular to AB such that VE=VF

Join A,E and extend , by construction VE/VA=VF/VA=1, the eccentricity.

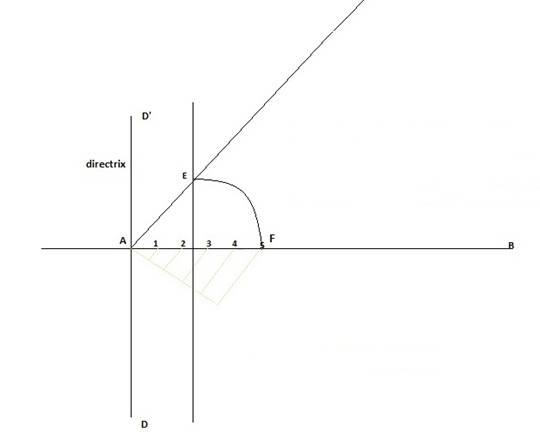
Locate a number of points 1,2,3, etc . to the right of V on the axis, which need not be equidistant.

Through the points 1,2,3 etc, draw  lines perpendicular to the axis and to meet the line AE extended at 1’,2’,3’, etc.

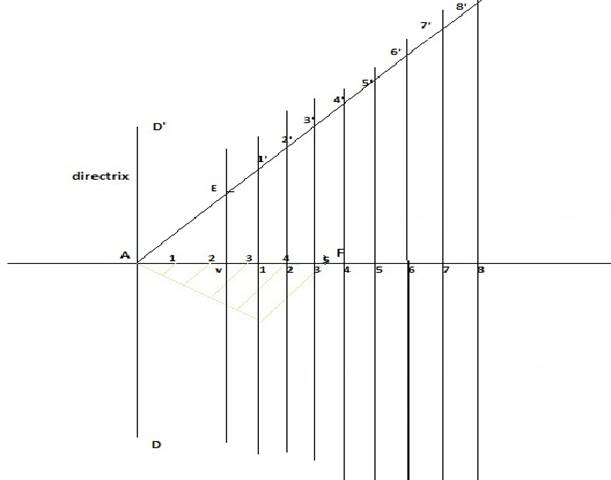
With the center F and radius 1-1’, draw arcs intersecting the line through 1 at P1 and P1’. P1 and P1’ are the points on the parabola, because, the distance of P1(P1’) from Fis 1-1’and from CD. It is A-1 and

1-1’/A-1=VE/VA=VF/VA=1

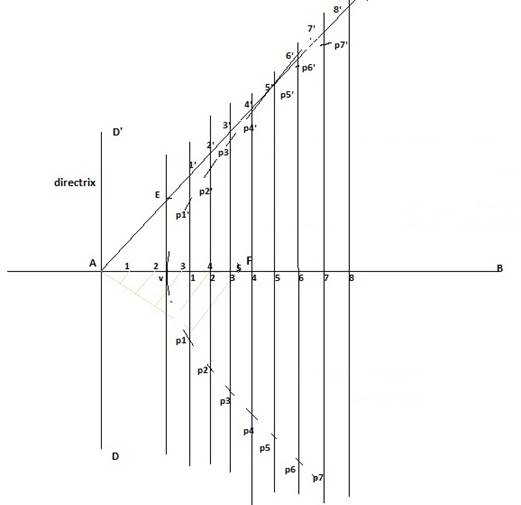
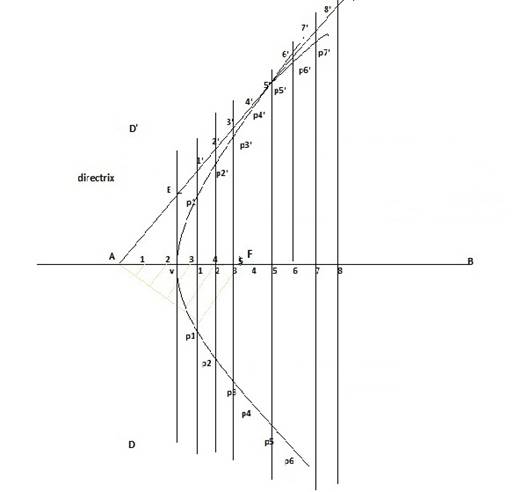
Similarly locate the points P2,P2’;P3,P3’; etc.. on either side of the axis.

Join the points by a smooth curve, forming the required parabola

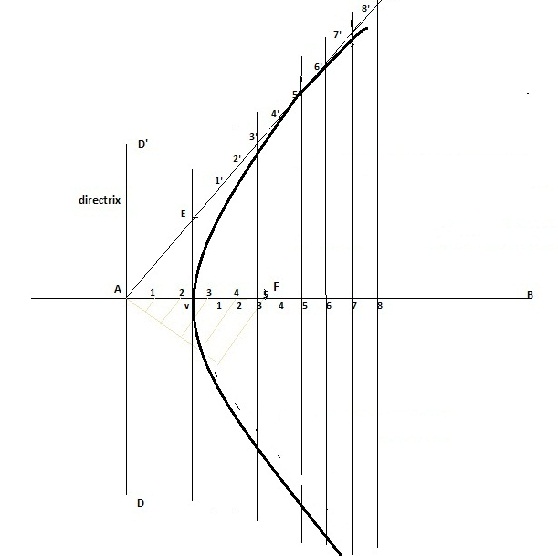
NOTE

OUR REQUIRED ONE ( PARABOLA) SHOULD BE DARK

hence our final figure is

To draw the tangent and normal to the parabola , locate the point M. which at a given distance from directrix

Then join M and  F and draw a line through F, perpendicular to MF, meeting the directrix at T.

The line joining T and M and extended (T-T) is the tangent and line N-N , through M and perpendicular to TM is the normal to the curve

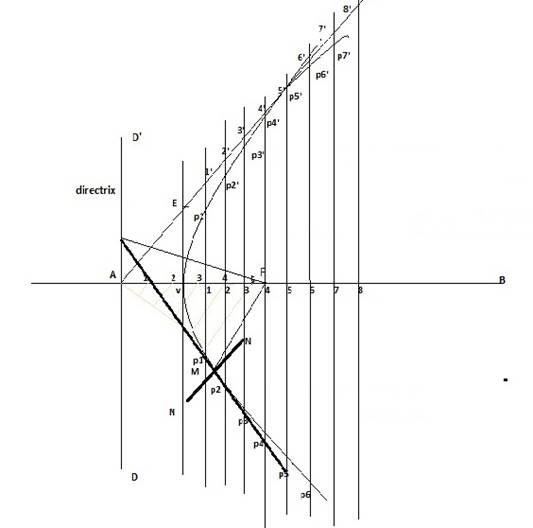
this is the construction of parabola by eccentricity method