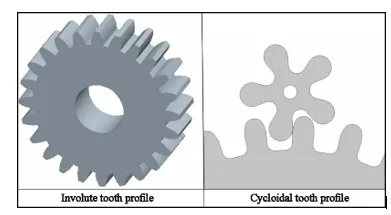
Comparison between Involute and Cycloidal Gear Tooth Profiles
Involute tooth profile is almost used generally everywhere and given preference over cycloidal tooth profile or any other gear tooth form. This doesn’t mean that involute tooth profile form is the best from every aspect.

Below the table, comparison between involute gear tooth and cycloidal gear tooth profiles provides a realistic assessment of the positive and negative points of both involute and cycloidal tooth profiles.
S.No | Involute Tooth Profile | Cycloidal Tooth Profile |
1 | Being the angle made by the common tangent of base circles with a common tangent to pitch circles at pitch point, the pressure angle remains constant throughout the engagement. This ensures smooth running of the gears. | Pressure angle varies continuously; being zero at the pitch point and maximum at the point of engagement and disengagement. This causes continuous variation in power component and also in bearing load. The running is less smooth. |
2 | Involute tooth profile consists of a single (involute) curve and the track cutter used for regenerating the profile has straight teeth. The rack cutter is cheaper and the method of manufacture is simpler. This leads to reduction in the cost of manufacture of involute teeth. | The cycloidal tooth profile consists of two curves (epicycloid and hypocycloid). The method of manufacture is more involved and leads to costlier gear tooth. |
3 | Perhaps the most desirable feature of involute teeth is that a small variation in centre distance does not change the velocity ratio. Thus distance between shafts need not necessarily be maintained exact as per design specifications. This gives great flexibility during assembly and larger tolerances may be permitted. | Exact centre distance is necessary to transmit constant velocity ratio. |
4 | Since involute curve doesn’t exist within base circle, interference is always possible if base circle radius is larger than dedendum circle radius. | Since outside the pitch (directing) circle epicycloidal curve exists and inside it the hypocycloidal curve exists, cycloidal curve can exist everywhere on tooth profile and no interference exists. |
5 | The radius of curvature of involute curve, near the base circle, is quite small and contact stresses are likely to be very high. The tooth profile in flank portion is almost radial. Both the factors together produce a tooth weaker in flank region compared to cycloidal tooth. | Cycloidal curve (hypocycloidal in particular) produces a spreading flank and, for this reason, cycloidal tooth is stronger . |
6 | In involute tooth profile gears, convex surface of pinion tooth comes in contact with convex portion of gear tooth and this leads to more wear. | In cycloidal tooth profile epicycloidal shape of face of gear tooth comes in contact with hypocloidal flank portion of pinion tooth. Thus a convex flank has a contact with concave face which results in lesser wear. |