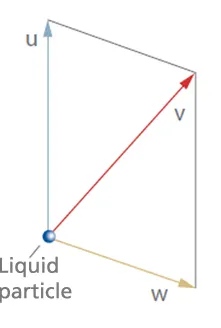
Velocity triangle

The velocity triangle is the vectorial representation of kinematic movement. A vector is a directional magnitude. This relationship is illustrated in the velocity parallelogram of a liquid particle. siehe Fig. 1 Velocity triangle

Fig. 1 Velocity triangle: Parallelogram of velocities u, v, w

The illustration of the relationships in the form of the velocity triangle, which is often also called a velocity diagram (not to be confused with forces diagram or vane profile) also shows the flow angles. See Fig. 2 Velocity triangle

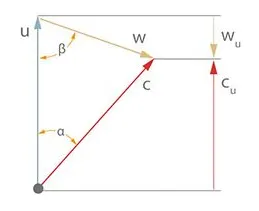
Fig. 2 Velocity triangle: With flow angle

Flow angles in the velocity triangle

§  Angle α between v and positive u direction ("absolute angle")

§  Angle β between w and negative u direction ("relative angle")

The position of one velocity triangle in relation to another velocity triangle does not indicate whether the flow velocities plotted on these triangles are in fact situated in the same positions in relation to each other in the actual flow. See Figs. 3 and 4 Velocity triangle

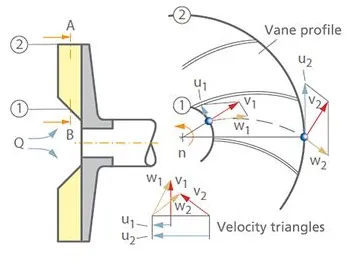
Fig. 3 Velocity triangle: Vane profile and velocity triangles for meridional section A-B through the radial impeller (longitudinal section through pump shaft)

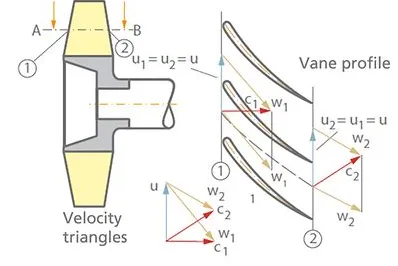
Fig. 4 Velocity triangle: Vane profile and velocity triangles for cylindrical section A-B through the axial impeller shown in meridional section

The absolute velocity of a liquid particle may be divided into axial, radial and peripheral components. See Fig. 5 Velocity triangle

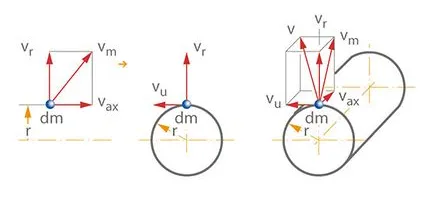
Fig. 5 Velocity triangle: Subdivision of a velocity into an axial, radial and circumferential component (example: absolute velocity v of a liquid particle dm)