Series Resonance

Resonance occurs in electric circuits due to the presence of energy storing elements like inductor and capacitor. It is the fundamental concept based on which, the radio and TV receivers are designed in such a way that they should be able to select only the desired station frequency. There are two types of resonances, namely series resonance and parallel resonance. These are classified based on the network elements that are connected in series or parallel. In this chapter, let us discuss about series resonance.

Series Resonance Circuit Diagram

If the resonance occurs in series RLC circuit, then it is called as Series Resonance. Consider the following series RLC circuit, which is represented in phasor domain.

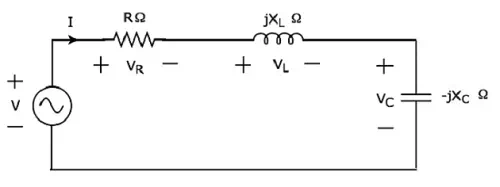
Here, the passive elements such as resistor, inductor and capacitor are connected in series. This entire combination is in series with the input sinusoidal voltage source.

Apply KVL around the loop.

V−VR−VL−VC=0V−VR−VL−VC=0

⇒V−IR−I(jXL)−I(−jXC)=0⇒V−IR−I(jXL)−I(−jXC)=0

⇒V=IR+I(jXL)+I(−jXC)⇒V=IR+I(jXL)+I(−jXC)

⇒V=I[R+j(XL−XC)]⇒V=I[R+j(XL−XC)]Equation 1

The above equation is in the form of V = IZ.

Therefore, the impedance Z of series RLC circuit will be

Z=R+j(XL−XC)Z=R+j(XL−XC)

Parameters & Electrical Quantities at Resonance

Now, let us derive the values of parameters and electrical quantities at resonance of series RLC circuit one by one.

Resonant Frequency

The frequency at which resonance occurs is called as resonant frequency f_r. In series RLC circuit resonance occurs, when the imaginary term of impedance Z is zero, i.e., the value of XL−XC$X_L-X_C$ should be equal to zero.

⇒XL=XC⇒XL=XC

Substitute XL=2πfLXL=2πfL and XC=12πfCXC=12πfC in the above equation.

2πfL=12πfC2πfL=12πfC

⇒f2=1(2π)2LC⇒f2=1(2π)2LC

⇒f=1(2π)LC−−−√⇒f=1(2π)LC

Therefore, the resonant frequency fr of series RLC circuit is

fr=1(2π)LC−−−√fr=1(2π)LC

Where, L is the inductance of an inductor and C is the capacitance of a capacitor.

The resonant frequency f_r of series RLC circuit depends only on the inductance L and capacitance C. But, it is independent of resistance R.

Impedance

We got the impedance Z of series RLC circuit as

Z=R+j(XL−XC)Z=R+j(XL−XC)

Substitute XL=XCXL=XC in the above equation.

Z=R+j(XC−XC)Z=R+j(XC−XC)

⇒Z=R+j(0)⇒Z=R+j(0)

⇒Z=R⇒Z=R

At resonance, the impedance Z of series RLC circuit is equal to the value of resistance R, i.e., Z = R.

Current flowing through the Circuit

Substitute XL−XC=0XL−XC=0 in Equation 1.

V=I[R+j(0)]V=I[R+j(0)]

⇒V=IR⇒V=IR

⇒I=VR⇒I=VR

Therefore, current flowing through series RLC circuit at resonance is I=VR$\mathit{I}\mathit{=}\frac{\mathit{V}}{\mathit{R}}$.

At resonance, the impedance of series RLC circuit reaches to minimum value. Hence, the maximum current flows through this circuit at resonance.

Voltage across Resistor

The voltage across resistor is

VR=IRVR=IR

Substitute the value of I in the above equation.

VR=⟮VR⟯RVR=⟮VR⟯R

⇒VR=V⇒VR=V

Therefore, the voltage across resistor at resonance is VR = V.

Voltage across Inductor

The voltage across inductor is

VL=I(jXL)VL=I(jXL)

Substitute the value of I in the above equation.

VL=⟮VR⟯(jXL)VL=⟮VR⟯(jXL)

⇒VL=j⟮XLR⟯V⇒VL=j⟮XLR⟯V

⇒VL=jQV⇒VL=jQV

Therefore, the voltage across inductor at resonance is VL=jQVVL=jQV.

So, the magnitude of voltage across inductor at resonance will be

|VL|=QV|VL|=QV

Where Q is the Quality factor and its value is equal to XLRXLR

Voltage across Capacitor

The voltage across capacitor is

VC=I(−jXC)VC=I(−jXC)

Substitute the value of I in the above equation.

VC=⟮VR⟯(−jXC)VC=⟮VR⟯(−jXC)

⇒VC=−j⟮XCR⟯V⇒VC=−j⟮XCR⟯V

⇒VC=−jQV⇒VC=−jQV

Therefore, the voltage across capacitor at resonance is VC=−jQVVC=−jQV.

So, the magnitude of voltage across capacitor at resonance will be

|VC|=QV|VC|=QV

Where Q is the Quality factor and its value is equal to XCRXCR

Note − Series resonance RLC circuit is called as voltage magnificationcircuit, because the magnitude of voltage across the inductor and the capacitor is equal to Q times the input sinusoidal voltage V.