Standing Wave Patterns
As mentioned earlier, all objects
have a frequency or set of frequencies with which they naturally vibrate when
struck, plucked, strummed or somehow disturbed. Each of the natural frequencies
at which an object vibrates is associated with a standing wave pattern. When an
object is forced into resonance vibrations at one of
its natural frequencies, it vibrates in a manner such that a standing wave is
formed within the object. The topic of standing wave patterns was introduced in Unit 10 of
The Physics Classroom. In that unit, a standing wave
pattern was described as a vibrational pattern created within a medium when the
vibrational frequency of a source causes reflected waves from one end of the
medium to interfere with incident waves from the source, The result
of the interference is that specific points along the medium appear to be
standing still while other points vibrated back and forth. Such patterns are
only created within the medium at specific frequencies of vibration. These
frequencies are known as harmonic frequencies or merely harmonics. At any frequency other than a harmonic frequency, the interference of
reflected and incident waves results in a disturbance of the medium that is
irregular and non-repeating.
Natural
Frequencies and Vibrational Patterns
So the natural frequencies of an object are merely the
harmonic frequencies at which standing wave patterns are established within the
object. These standing wave patterns represent the lowest energy vibrational
modes of the object. While there are countless ways by which an object can
vibrate (each associated with a specific frequency), objects favor only a few specific modes or
patterns of vibrating. The favored modes
(patterns) of vibration are those that result in the highest amplitude
vibrations with the least input of energy. Objects favor these
natural modes of vibration because they are representative of the patterns that
require the least amount of energy. Objects are most easily forced into
resonance vibrations when disturbed at frequencies associated with these
natural frequencies.
The wave pattern associated with the natural frequencies of
an object is characterized by points that appear to be standing still. For this
reason, the pattern is often called a "standing wave pattern." The points in the pattern that are standing still are referred
to as nodal points or nodal positions. These positions occur as
the result of the destructive interference of incident and reflected waves.
Each nodal point is surrounded by antinodal points, creating an alternating pattern of nodal
and antinodal points. Such patterns were
introduced in Unit 10 of The Physics Classroom Tutorial. In this
unit, we will elaborate on the essential characteristics and the causes of
standing wave patterns and relate these patterns to the vibrations of musical
instruments.
Chladni Plates
A common Physics demonstration utilizes a square metal plate
(known as a Chladni plate), a violin bow
and salt. The plate is securely fastened to a table using a nut and bolt. The
nut and bolt 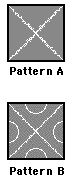 are clamped to the center of the
square plate, preventing that section from vibrating. Salt (or sand) is
sprinkled upon the plate in an irregular pattern. Then the violin bow is used
to induce vibrations within the plate; the plate is strummed and begins
vibrating. And then the magic occurs. A high-pitched pure tone is sounded out
as the plate vibrates. And, remarkably (as is often the case in a physics
class), the salt upon the plate begins vibrating and forms a pattern upon the
plate. As we know, all objects (even a silly little metal plate) have a set of
natural frequencies at which they vibrate; and each frequency is associated
with a standing wave pattern. The pattern formed by the salt on the plate is
the standing wave pattern associated with one of the natural frequencies of
the Chladni plate. As the plate vibrates,
the salt begins to vibrate and tumble about the plate until it reaches points
along the plate that are not vibrating. Subsequently, the salt finally comes to
rest along the nodal positions. The diagrams at the right show two of the most
common standing wave patterns for the Chladni plates.
The white lines represent the salt locations (nodal positions). Observe in the
diagram that each pattern is characterized by nodal positions in the corners of
the square plate and in the center of the
plate. For these two particular vibrational modes, those positions are unable
to move. Being unable to move, they become nodal points - points of no
displacement.
are clamped to the center of the
square plate, preventing that section from vibrating. Salt (or sand) is
sprinkled upon the plate in an irregular pattern. Then the violin bow is used
to induce vibrations within the plate; the plate is strummed and begins
vibrating. And then the magic occurs. A high-pitched pure tone is sounded out
as the plate vibrates. And, remarkably (as is often the case in a physics
class), the salt upon the plate begins vibrating and forms a pattern upon the
plate. As we know, all objects (even a silly little metal plate) have a set of
natural frequencies at which they vibrate; and each frequency is associated
with a standing wave pattern. The pattern formed by the salt on the plate is
the standing wave pattern associated with one of the natural frequencies of
the Chladni plate. As the plate vibrates,
the salt begins to vibrate and tumble about the plate until it reaches points
along the plate that are not vibrating. Subsequently, the salt finally comes to
rest along the nodal positions. The diagrams at the right show two of the most
common standing wave patterns for the Chladni plates.
The white lines represent the salt locations (nodal positions). Observe in the
diagram that each pattern is characterized by nodal positions in the corners of
the square plate and in the center of the
plate. For these two particular vibrational modes, those positions are unable
to move. Being unable to move, they become nodal points - points of no
displacement.
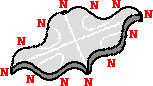
Flickr Physics Photo
Salt is sprinkled onto a metal plate. The plate
is strummed with a violin bow and set into vibration. The salt crystals vibrate
about the plate until they settle onto positions of nodes (points of no despacement). Several patterns can be obtained, each associated with a
unique frequency of vibrations. These standing wave patterns are known as Chladni patterns,
named in honor of a
19th century German physicist who advanced our understanding of acoustics and
the physics of music.
Standing Wave Patterns for Vibrating Strings
The diagram below depicts one of the natural patterns of
vibrations for a guitar string. In the pattern, you will note that there are
certain positions along the string (the medium) that appear to be standing still.
These positions are referred to as nodes and are labeled on
the diagram. In between each nodal position, there are other positions that
appear to be vibrating back and forth between a large upward displacement to a large downward displacement. These points
are referred to as antinodes and are also labeled on
the diagram. There is an alternating pattern of nodal and antinodal positions in a standing wave pattern.
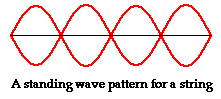
Because the antinodal positions
along the guitar string are vibrating back and forth from a large upward
displacement to a large downward displacement, the standing wave pattern is
often depicted by a diagram such as that shown below.
 The pattern above is not the only pattern of vibration for a guitar
string. There are a variety of patterns by which the guitar string could
naturally vibrate. Each pattern is associated with one of the natural
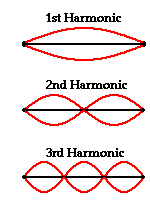
frequencies of the guitar strings. Three other patterns are shown in the
diagrams at the right. Each standing wave pattern is referred to as a harmonic
of the instrument (in this case, the guitar string). The three diagrams at the
right represent the standing wave patterns for the first, second, and third
harmonics of a guitar string. (Harmonics will be discussed in more detail in the next
section of this lesson.)
The pattern above is not the only pattern of vibration for a guitar
string. There are a variety of patterns by which the guitar string could
naturally vibrate. Each pattern is associated with one of the natural
frequencies of the guitar strings. Three other patterns are shown in the
diagrams at the right. Each standing wave pattern is referred to as a harmonic
of the instrument (in this case, the guitar string). The three diagrams at the
right represent the standing wave patterns for the first, second, and third
harmonics of a guitar string. (Harmonics will be discussed in more detail in the next
section of this lesson.)
There are a variety of other low energy vibrational patterns
that could be established in the string. For guitar strings, each pattern is
characterized by some basic traits:
· There is
an alternating pattern of nodes and antinodes.
· There are
either a half-number or a whole number of waves within the pattern established
on the string.
· Nodal
positions (points of no displacement) are established at the ends of the string
where the string is clamped down in a fixed position.
· One
pattern is related to the next pattern by the addition (or subtraction) of one
or more nodes (and antinodes).
