Mathematics of Standing Waves
As discussed in Lesson 4, standing
wave patterns are wave patterns produced in a medium when two waves of identical
frequencies interfere in such a manner to produce points along the medium that
always appear to be standing still. Such standing wave patterns are produced
within the medium when it is vibrated at certain frequencies. Each frequency is
associated with a different standing wave pattern. These frequencies and their
associated wave patterns are referred to as harmonics. A careful study of the standing wave patterns reveal a clear
mathematical relationship between the wavelength of the wave that produces the
pattern and the length of the medium in which the pattern is displayed.
Furthermore, there is a predictability about this mathematical relationship
that allows one to generalize and deduce a statement concerning this
relationship. To illustrate, consider the first harmonic standing wave pattern
for a vibrating rope as shown below.
Analyzing
the First Harmonic Pattern
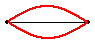
The pattern for the first harmonic reveals a single antinode
in the middle of the rope. This antinode position along the rope vibrates up
and down from a maximum upward displacement from rest to a maximum downward
displacement as shown. The vibration of the rope in this manner creates the
appearance of a loop within the string. A complete wave in a pattern could be described as
starting at the rest position, rising upward to a peak displacement, returning
back down to a rest position, then descending to a peak downward displacement
and finally returning back to the rest position. The animation below depicts
this familiar pattern. As shown in the animation, one complete wave in a
standing wave pattern consists of two loops. Thus, one loop
is equivalent to one-half of a wavelength.
In comparing the standing wave pattern for the first harmonic
with its single loop to the diagram of a complete wave, it is evident that
there is only one-half of a wave stretching across the length of the string.
That is, the length of the string is equal to one-half the length of a wave.
Put in the form of an equation:
Analyzing
the Second Harmonic Pattern
Now consider the string being vibrated with a
frequency that establishes the standing wave pattern for the second harmonic.
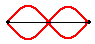
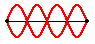
The second harmonic pattern consists of two anti-nodes. Thus,
there are two loops within the length of the string. Since each loop is
equivalent to one-half a wavelength, the length of the string is equal to
two-halves of a wavelength. Put in the form of an equation:
The same reasoning pattern can be applied to the case of the
string being vibrated with a frequency that establishes the standing wave
pattern for the third harmonic.
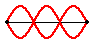
Analyzing
the Third Harmonic Pattern
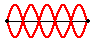
The third harmonic pattern consists of three
anti-nodes. Thus, there are three loops within the length of the string. Since
each loop is equivalent to one-half a wavelength, the length of the string is
equal to three-halves of a wavelength. Put in the form of an equation:
When inspecting the standing wave patterns and the
length-wavelength relationships for the first three harmonics, a clear pattern
emerges. The number of antinodes in the pattern is equal to the harmonic
number of that pattern. The first harmonic has one antinode; the second
harmonic has two antinodes; and the third harmonic has three antinodes. Thus,
it can be generalized that the nth harmonic has n antinodes
where n is an integer representing the harmonic number. Furthermore, one notices
that there are n halves wavelengths present within the length of the string. Put in the
form of an equation:
Summarizing
the Mathematical Relationships
This information is summarized in the table below.
|
Harmonic |
Pattern |
# of Loops |
Length-Wavelength Relationship |
|
1st |
|
1 |
L = 1 / 2 • λ |
|
2nd |
|
2 |
L = 2 / 2 • λ |
|
3rd |
|
3 |
L = 3 / 2 • λ |
|
4th |
|
4 |
L = 4 / 2 • λ |
|
5th |
|
5 |
L = 5 / 2 • λ |
|
6th |
|
6 |
L = 6 / 2 • λ |
|
nth |
-- |
n |
L = n / 2 • λ |
For standing wave patterns, there is a clear mathematical
relationship between the length of a string and the wavelength of the wave that
creates the pattern. The mathematical relationship simply emerges from the
inspection of the pattern and the understanding that each loop in the pattern
is equivalent to one-half of a wavelength. The general equation that describes
this length-wavelength relationship for any harmonic is:
Test your understanding of this relationship by answering the
questions below.
Check Your Understanding
1. Suppose that a string is 1.2 meters long and vibrates in
the first, second and third harmonic standing wave patterns. Determine the
wavelength of the waves for each of the three patterns.
|
First harmonic: 2.4 m |
Second harmonic: 1.2 m |
Third harmonic: 0.8 m |
For the first harmonic, the length of the string is equivalent
to one-half of a wavelength. If the string is 1.2 meters long, then one-half of
a wavelength is 1.2 meters long. The full wavelength is 2.4 meters long.
For the second harmonic, the length of the string is equivalent
to a full wavelength. If the string is 1.2 meters long, then the wavelength is
1.2 meters long.
For the third
harmonic, the length of the string is equivalent to three-halves of a
wavelength. If the string is 1.2 meters long, then 1.5 wavelengths is 1.2
meters long. A single wavelength is less than 1.2 meters; it can be found by
dividing 1.2 meters by 1.5. The wavelength of the third harmonic is 0.8 meters.
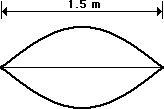2. The string at the right is 1.5 meters long and is vibrating as the
first harmonic. The string vibrates up and down with 33 complete vibrational
cycles in 10 seconds. Determine the frequency, period, wavelength and speed for
this wave.
Given: L = 1.5 m
33 cycles in 10 seconds
The frequency refers to how often a point on the medium
undergoes back-and-forth vibrations; it is measured as the number of cycles per
unit of time. In this case, it is
f = (33 cycles)
/ (10 seconds) = 3.3 Hz
The period is the reciprocal of the frequency.
T = 1 / (3.3 Hz)
= 0.303
seconds
The wavelength of the wave is related to the length of the rope.
For the first harmonic as pictured in this problem, the length of the rope is
equivalent to one-half of a wavelength. That is, L = 0.5 • W where W is the wavelength.
Rearranging the equation and substituting leads to the following results:
W = 2 • L = 2 •
(1.5 m) = 3.0
m
The speed of a wave can be calculated from its wavelength and
frequency using the wave equation:
v = f • W = (3.3
Hz) • (3. 0 m) = 9.9 m/s
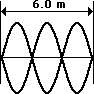3. The string at the right is 6.0 meters long and is vibrating as the
third harmonic. The string vibrates up and down with 45 complete vibrational
cycles in 10 seconds. Determine the frequency, period, wavelength and speed for
this wave.
Given: L = 6.0 m
45 cycles in 10 seconds
The frequency refers to how often a point on the medium
undergoes back-and-forth vibrations; it is measured as the number of cycles per
unit of time. In this case, it is
f = (45 cycles)
/ (10 seconds) = 4.5 Hz
The period is the reciprocal of the frequency.
T = 1 / (4.5 Hz)
= 0.222
seconds
The wavelength of the wave is related to the length of the rope.
For the third harmonic as pictured in this problem, the length of the rope is
equivalent to three-halves of a wavelength. That is, L = 1.5 • W where W is the
wavelength. Rearranging the equation and substituting leads to the following
results:
W = (2 / 3) • L
= (2 / 3) • (6.0 m) = 4.0 m
The speed of a wave can be calculated from its wavelength and
frequency using the wave equation:
v = f • W = (4.5
Hz) • (4. 0 m) = 18 m/s
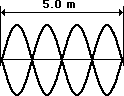4. The string at the right is 5.0 meters long and is vibrating as the
fourth harmonic. The string vibrates up and down with 48 complete vibrational
cycles in 20 seconds. Determine the frequency, period, wavelength and speed for
this wave.
Given: L = 5.0 m
48 cycles in 20 seconds
The frequency refers to how often a point on the medium
undergoes back-and-forth vibrations; it is measured as the number of cycles per
unit of time. In this case, it is
f = (48 cycles)
/ (20 seconds) = 2.4 Hz
The period is the reciprocal of the frequency.
T = 1 / (2.4 Hz)
= 0.417
seconds
The wavelength of the wave is related to the length of the rope.
For the fourth harmonic as pictured in this problem, the length of the rope is
equivalent to two full wavelengths. That is, L = 2 • W where W is the
wavelength. Rearranging the equation and substituting leads to the following
results:
W = 0.5 • L =
0.5 • (5.0 m) = 2.5 m
The speed of a wave can be calculated from its wavelength and
frequency using the wave equation:
v = f • W = (2.4 Hz) • (2.5 m) = 6.0 m/s
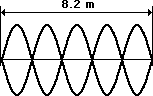5. The string at the right is 8.2 meters long and is vibrating as the
fifth harmonic. The string vibrates up and down with 21 complete vibrational
cycles in 5 seconds. Determine the frequency, period, wavelength and speed for
this wave.
Given:
L = 8.2 m
21
cycles in 5 seconds
The
frequency refers to how often a point on the medium undergoes back-and-forth
vibrations; it is measured as the number of cycles per unit of time. In this
case, it is
f = (21 cycles)
/ (5 seconds) = 4.2 Hz
The
period is the reciprocal of the frequency.
T = 1 / (4.2 Hz)
= 0.238 seconds
The
wavelength of the wave is related to the length of the rope. For the fifth
harmonic as pictured in this problem, the length of the rope is equivalent to
five-halves of a wavelength. That is, L = w.5 • W where W is the wavelength.
Rearranging the equation and substituting leads to the following results:
W = (2 / 5) • L
= (2 / 5) • (8.2 m) = 3.28 m
The
speed of a wave can be calculated from its wavelength and frequency using the
wave equation:
v = f • W = (4.2 Hz) • (3.28 m) = 13.8 m/s