Electric Current
If the two requirements of an electric circuit are met,
then charge will flow through the external circuit. It is said that there is a
current - a flow of charge. Using the word current in this
context is to simply use it to say that something is happening in the wires -
charge is moving. Yet current is a physical quantity that can be measured and
expressed numerically. As a physical quantity, current is the
rate at which charge flows past a point on a circuit. As depicted in the
diagram below, the current in a circuit can be determined if the quantity of
charge Q passing through a cross section of a wire in a time t can be
measured. The current is simply the ratio of the quantity of charge and time.
Current is a rate quantity. There are several
rate quantities in physics. For instance, velocity is a rate
quantity - the rate at which an object changes its position. Mathematically,
velocity is the position change per time ratio. Acceleration is a rate
quantity - the rate at which an object changes its velocity. Mathematically,
acceleration is the velocity change per time ratio. And power is a rate
quantity - the rate at which work is done on an object. Mathematically, power
is the work per time ratio. In every case of a rate quantity, the mathematical
equation involves some quantity over time. Thus, current as a rate quantity
would be expressed mathematically as
Note that the equation above uses the symbol I to
represent the quantity current.
As is the usual case, when a quantity is introduced in The
Physics Classroom, the standard metric unit used to express that quantity is
introduced as well. The standard metric unit for current is the ampere. Ampere is often shortened to Amp and is
abbreviated by the unit symbol A. A current of 1
ampere means that there is 1 coulomb of charge passing through a cross section
of a wire every 1 second.
1 ampere = 1 coulomb / 1 second
To test your understanding, determine the current for the
following two situations. Note that some extraneous information is given in
each situation. Click the Check Answer button to
see if you are correct.
|
A 2 mm long cross section of wire is
isolated and 20 C of charge is determined to pass through it in 40 s.
|
A 1 mm long cross section of wire is
isolated and 2 C of charge is determined to pass through it in 0.5 s.
|
|
I = _____ Ampere |
I = _____ Ampere |
Conventional Current Direction
The particles that carry charge through wires in a circuit
are mobile electrons. The electric field direction within a circuit is by
definition the direction that positive test charges are pushed. Thus, these
negatively charged electrons move in the direction opposite the electric field.
But while electrons are the charge carriers in metal wires, the charge carriers
in other circuits can be positive charges, negative charges or both. In fact,
the charge carriers in semiconductors, street lamps and fluorescent lamps are
simultaneously both positive and negative charges traveling in opposite
directions.
Ben Franklin, who conducted extensive scientific studies in both static
and current electricity, envisioned positive charges as the carriers of charge.
As such, an early convention for the direction of an electric current was
established to be in the direction that positive charges would move. The
convention has stuck and is still used today. The direction of
an electric current is by convention the direction in which a
positive charge would move. Thus, the current in the external circuit is
directed away from the positive terminal and toward the negative terminal of
the battery. Electrons would actually move through the wires in the opposite
direction. Knowing that the actual charge carriers in wires are negatively
charged electrons may make this convention seem a bit odd and outdated.
Nonetheless, it is the convention that is used worldwide and one that a student
of physics can easily become accustomed to.
Current
versus Drift Speed
Current has to do with the number of coulombs of charge that
pass a point in the circuit per unit of time. Because of its definition, it is
often confused with the quantity drift speed. Drift speed refers to
the average distance traveled by a charge
carrier per unit of time. Like the speed of any object, the drift speed of an
electron moving through a wire is the distance to time ratio. 
The path of a typical electron through a wire could be described as a
rather chaotic, zigzag path characterized by collisions with fixed atoms. Each
collision results in a change in direction of the electron. Yet because of
collisions with atoms in the solid network of the metal conductor, there are
two steps backwards for every three steps forward. With an electric potential
established across the two ends of the circuit, the electron continues to migrate
forward. Progress is always made towards the positive
terminal. Yet the overall effect of the countless collisions and the high
between-collision speeds is that the overall drift speed of an electron in a
circuit is abnormally low. A typical drift speed might be 1 meter per hour.
That is slow!
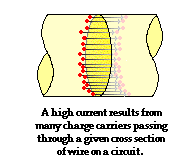One might then ask: How can there by a current on the order of 1 or 2
ampere in a circuit if the drift speed is only about 1 meter per hour? The
answer is: there are many, many charge carriers moving at once throughout the
whole length of the circuit. Current is the rate at which charge crosses a
point on a circuit. A high current is the result of several coulombs of charge
crossing over a cross section of a wire on a circuit. If the charge carriers
are densely packed into the wire, then there does not have to be a high speed
to have a high current. That is, the charge carriers do not have to travel a
long distance in a second, there just has to be a lot of them passing through
the cross section. Current does not have to do with how far charges move in a
second but rather with how many charges pass through a cross section of wire on
a circuit.
To illustrate how densely packed the charge carriers are, we
will consider a typical wire found in household lighting circuits - a 14-gauge
copper wire. In a 0.01 cm-long (very thin) cross-sectional slice of this wire,
there would be as many as 3.51 x 1020 copper
atoms. Each copper atom has 29 electrons; it would be unlikely that even the 11
valence electrons would be in motion as charge carriers at once. If we assume
that each copper atom contributes just a single electron, then there would be
as much as 56 coulombs of charge within a thin 0.01-cm length of the wire. With
that much mobile charge within such a small space, a small drift speed could
lead to a very large current.
To further illustrate this distinction between drift speed
and current, consider this racing analogy. Suppose that there was a very large
turtle race with millions and millions of turtles on a very wide race track.
Turtles do not move very fast - they have a very low drift speed.
Suppose that the race was rather short - say 1 meter in length - and that a
large percentage of the turtles reached the finish line at the same time - 30
minutes after the start of the race. In such a case, the current would be very
large - with millions of turtles passing a point in a short amount of time. In
this analogy, speed has to do with how far the turtles move in a certain amount
of time; and current has to do with how many turtles cross the finish line in a
certain amount of time.
The
Nature of Charge Flow
Once it has been established that the average drift speed of
an electron is very, very slow, the question soon arises: Why does the light in
a room or in a flashlight light immediately after the switched is turned on?
Wouldn't there be a noticeable time delay before a charge carrier moves from
the switch to the light bulb filament? The answer is NO! and the explanation of why reveals a significant amount
about the nature of charge flow in a circuit.
As mentioned above, charge carriers in the wires of electric circuits are electrons. These
electrons are simply supplied by the atoms of copper (or whatever material the
wire is made of) within the metal wire. Once the switch is turned to on, the circuit is closed and there is an electric potential difference is
established across the two ends of the external circuit. The electric field
signal travels at nearly the speed of light to all mobile 
electrons within the circuit, ordering them to beginmarching.
As the signal is received, the electrons begin moving along a zigzag path in
their usual direction. Thus, the flipping of the switch causes an immediate
response throughout every part of the circuit, setting charge carriers
everywhere in motion in the same net direction. While the actual motion of
charge carriers occurs with a slow speed, the signal that informs them to
start moving travels at a fraction of the speed of light.
The electrons that light the bulb in a
flashlight do not have to first travel from the switch through 10 cm of wire to
the filament. Rather, the electrons that light the bulb immediately after the
switch is turned to onare the
electrons that are present in the filament itself. As the switch is flipped,
all mobile electrons everywhere begin marching; and it is the mobile electrons
present in the filament whose motion are immediately responsible for the
lighting of its bulb. As those electrons leave the filament, new electrons
enter and become the ones that are responsible for lighting the bulb. The
electrons are moving together much like the water in the pipes of a home move.
When a faucet is turned on, it is the water
in the faucet that emerges from the spigot. One does not have to wait a
noticeable time for water from the entry point to your home to travel through
the pipes to the spigot. The pipes are already filled with water and water
everywhere within the water circuit is set in motion at the same time.
The picture of charge flow being developed here
is a picture in which charge carriers are like soldiers marching along
together, everywhere at the same rate. Their marching begins immediately in
response to the establishment of an electric potential across the two ends of
the circuit. There is no place in the electrical circuit 
where charge carriers become consumed or used up. While the energy
possessed by the charge may be used up (or a better way of putting this is to
say that the electric energy is transformed to other forms of energy), the
charge carriers themselves do not disintegrate, disappear or otherwise become
removed from the circuit. And there is no place in the circuit where charge
carriers begin to pile up or accumulate. The rate at which charge enters the
external circuit on one end is the same as the rate at which charge exits the
external circuit on the other end. Current - the rate of charge flow - is
everywhere the same. Charge flow is like the movement of soldiers marching in
step together, everywhere at the same rate.
Check Your Understanding
1. A current is said to exist whenever _____.
a. a wire is charged
b. a battery is
present
c. electric
charges are unbalanced
d. electric
charges move in a loop
Answer: D
Current is the
rate at which charge flows. Charge will not flow in a circuit unless there is
an energy source capable of creating an electric potential difference and
unless there is a closed conducting loop through which the charge can move.
2. Current has a direction. By convention, current is in the
direction that ___.
a.
+ charges move
b. - electrons move
c. + electrons move
Answer: A
By convention,
the electric current direction is the direction which positive charge would
move. In wires, the actual charge carriers are negatively charged electrons.
Nonetheless, the convention used for the direction of current is based on the
direction which positive charges would move.
3. The drift velocity of mobile charge carriers in electric
circuits is ____.
a. very fast; less than but very close to the
speed of light
b. fast; faster
than the fastest car but nowhere near the speed of light
c. slow; slower
than Michael Jackson runs the 220-meters
d. very slow;
slower than a snail
Answer: D
The
average speed of an electron within a circuit is very, very slow. This is due
primarily to the countless collisions with the fixed atoms in the wire. Actual
drift speeds depend upon numerous factors. A typical drift speed would be about
1 meter per hour.
4. If an electric circuit could be compared to a water
circuit at a water park, then the current would be analogous to the ____.
Choices:
|
A. water
pressure |
B.
gallons of water flowing down slide per minute |
|
C. water |
D. bottom
of the slide |
|
E. water
pump |
F. top of
the slide |
Answer: B
Current is the
rate at which something flows. Electric current is the rate at
which electric charge flows past a point on the electric circuit. Water current
is the rate at which water flows past a point on the water circuit. As such,
current is analogous to the number of gallons of water flowing into, along, and
out of a slide per unit of time.
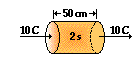5. The diagram at the right depicts a conducting wire. Two
cross-sectional areas are located 50 cm apart. Every 2.0 seconds, 10 C of
charge flow through each of these areas. The current in this wire is ____ A.
|
a. 0.10 |
b. 0.25 |
c. 0.50 |
d. 1.0 |
|
e. 5.0 |
f. 20 |
g. 10 |
h. 40 |
|
i. none of these |
|||
Answer: E
Current is the ratio of charge to time. The quantity of charge
passing through a cross section in 2 seconds is 10 C. The ratio of charge to
time is
I = Q / t
= ( 10 C) / ( 2 s) = 5 C/s = 5 Ampere
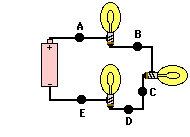6. Use the diagram at the right to complete the following statements:
a. A current of one ampere is a flow of charge at the rate of
_______ coulomb per second.
b. When a charge of 8 C flows past any point along a circuit
in 2 seconds, the current is ________ A.
c. If 5 C of
charge flow past point A (diagram at right) in 10 seconds, then the current is
_________ A.
d. If the current at point D is 2.0 A, then _______ C of
charge flow past point D in 10 seconds.
e. If 12 C of charge flow past point A in 3 seconds, then 8 C
of charge will flow past point E in ________ seconds.
f. True or False:
The
current at point E is considerably less than the current at point A since
charge is being used up in the light bulbs.
To answer all these questions, use the mathematical equation for
current: I
= Q / t
a. A current of one ampere is a flow of charge at the rate
of 1 coulomb per second.
b. When a charge of 8 coulombs flows past any point along a
circuit in 2 seconds, the current is 4 A.
c. If 5 coulombs of charge flow past point A (diagram at right)
in 10 seconds, then the current is 0.5 A.
d. If the current at point D is 2.0 A, then 20 coulombs of charge
flow past point D in 10 seconds.
e. If 12 coulombs of charge flow past point A in 3 seconds, then
8 coulombs of charge will flow past point E in 2 seconds. (The current
is 12 C / 3 s or 4 Amperes at point A. Since current is everywhere the same, it
is also 4 Amperes at point E. So the ratio of Q to t is 4 C / s.)
f. False. The current is everywhere
the same within an electric circuit.