Series Circuits
As mentioned in the previous section of Lesson 4, two or more electrical devices in a circuit can be connected by series
connections or by parallel connections. When all the devices are connected
using series connections, the circuit is referred to 
as a series circuit. In a series circuit, each device is connected
in a manner such that there is only one pathway by which charge can traverse
the external circuit. Each charge passing through the loop of the external
circuit will pass through each resistor in consecutive fashion.
A short comparison and contrast between series and parallel
circuits was made in the previous section of Lesson 4. In that section, it was emphasized that the act of adding more
resistors to a series circuit results in the rather expected result of having
more overall resistance. Since there is only one pathway through the circuit,
every charge encounters the resistance of every device; so adding more devices
results in more overall resistance. This increased resistance serves to reduce
the rate at which charge flows (also known as the current).
Equivalent
Resistance and Current
Charge flows together through the external circuit at a rate
that is everywhere the same. The current is no greater at one location as it is
at another location. The actual amount of current varies inversely with the
amount of overall resistance. There is a clear relationship between the
resistance of the individual resistors and the overall resistance of the
collection of resistors. As far as the battery that is pumping the charge is
concerned, the presence of two 6-Ω ;resistors in
series would be equivalent to having one 12-Ω resistor in the circuit. The
presence of three 6-Ω resistors in series would be equivalent to having
one 18-Ω resistor in the circuit. And the presence of four 6-Ω
resistors in series would be equivalent to having one 24-Ω resistor in the
circuit.
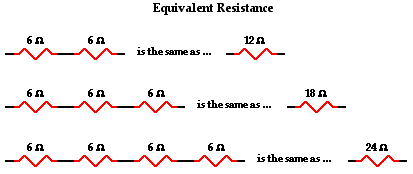
This is the concept of equivalent resistance. The equivalent
resistance of a circuit is the amount of resistance that a single resistor would
need in order to equal the overall effect of the collection of resistors that
are present in the circuit. For series circuits, the mathematical formula for
computing the equivalent resistance (Req) is
Req =
R1 + R2 + R3 + ...
where R1,
R2, and R3 are the resistance values of the individual
resistors that are connected in series.
The current in a series circuit is everywhere the same.
Charge does NOT pile up and begin to accumulate at any given location such that
the current at one location is more than at other locations. Charge does NOT
become used up by resistors such that there is less of it at one location
compared to another. The charges can be thought of as marching together through
the wires of an electric circuit, everywhere marching at the same rate. Current - the rate
at which charge flows - is everywhere the same. It is the same at the first
resistor as it is at the last resistor as it is in the battery. Mathematically,
one might write
Ibattery =
I1 = I2 = I3 = ...
where I1,
I2, and I3 are the current values at the individual
resistor locations.
These current values are easily calculated if the battery
voltage is known and the individual resistance values are known. Using the
individual resistor values and the equation above, the equivalent resistance
can be calculated. And using Ohm's law (ΔV =
I • R), the current in the battery and thus through every resistor can be
determined by finding the ratio of the battery voltage and the equivalent
resistance.
Ibattery =
I1 = I2 = I3 = ΔVbattery / Req
Electric
Potential Difference and Voltage Drops
As discussed in Lesson 1, the
electrochemical cell of a circuit supplies energy to the charge to move it
through the cell and to establish an electric potential difference across the
two ends of the external circuit. A 1.5-volt cell will establish an electric
potential difference across the external circuit of 1.5 volts. This is to say
that the electric potential at the positive terminal is 1.5 volts greater than
at the negative terminal. As charge moves through the external circuit, it
encounters a loss of 1.5 volts of electric potential. This loss in electric
potential is referred to as a voltage drop. It occurs as
the electrical energy of the charge is transformed to other forms of energy
(thermal, light, mechanical, etc.) within the resistors or loads. If an
electric circuit powered by a 1.5-volt cell is equipped with more than one
resistor, then the cumulative loss of electric potential is 1.5 volts. There is
a voltage drop for each resistor, but the sum of these voltage drops is 1.5
volts - the same as the voltage rating of the power supply. This concept can be
expressed mathematically by the following equation:
ΔVbattery =
ΔV1 + ΔV2 + ΔV3 + ...
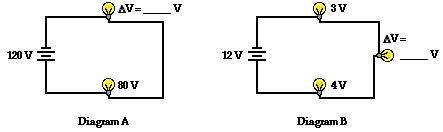
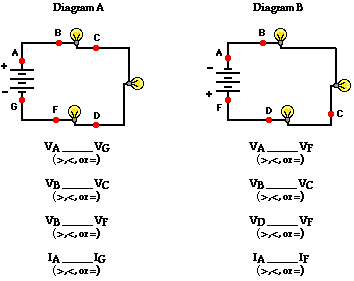
To illustrate this mathematical principle in action, consider the two
circuits shown below in Diagrams A and B. Suppose that you were to asked to determine the two unknown values of the
electric potential difference across the light bulbs in each circuit. To
determine their values, you would have to use the equation above. The battery
is depicted by its customary schematic symbol and its
voltage is listed next to it. Determine the voltage drop for the two light
bulbs and then click the Check Answers button to see if you are correct.
Earlier in Lesson 1, the use of an electric
potential diagram was discussed. An electric potential diagram is
a conceptual tool for representing the electric potential difference between
several points on an electric circuit. Consider the circuit diagram below and
its corresponding electric potential diagram.
The circuit shown in the diagram above is powered by a
12-volt energy source. There are three resistors in the circuit connected in
series, each having its own voltage drop. The negative sign for the electric
potential difference simply denotes that there is a loss in electric potential
when passing through the resistor. Conventional current is
directed through the external circuit from the positive terminal to the
negative terminal. Since the schematic symbol for a
voltage source uses a long bar to represent the positive terminal, location A
in the diagram is at the positive terminal or the high potential terminal.
Location A is at 12 volts of electric potential and location H (the negative
terminal) is at 0 volts. In passing through the battery, the charge gains 12
volts of electric potential. And in passing through the external circuit, the
charge loses 12 volts of electric potential as depicted by the electric
potential diagram shown to the right of the schematic diagram. This 12 volts of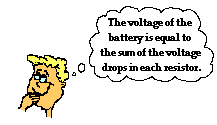 
electric potential is lost in three steps with each step corresponding
to the flow through a resistor. In passing through the connecting wires between
resistors, there is little loss in electric potential due to the fact that a
wire offers relatively little resistance to the flow of charge. Since locations
A and B are separated by a wire, they are at virtually the same electric
potential of 12 V. When a charge passes through its first resistor, it loses 3
V of electric potential and drops down to 9 V at location C. Since location D
is separated from location C by a mere wire, it is at virtually the same 9 V
electric potential as C. When a charge passes through its second resistor, it
loses 7 V of electric potential and drops down to 2 V at location E. Since
location F is separated from location E by a mere wire, it is at virtually the
same 2 V electric potential as E. Finally, as a charge passes through its last
resistor, it loses 2 V of electric potential and drops down to 0 V at G. At
locations G and H, the charge is out of energy and needs an energy boost in
order to traverse the external circuit again. The energy boost is provided by
the battery as the charge is moved from H to A.
In Lesson 3, Ohm's law (ΔV =
I • R) was introduced as an equation that relates the voltage drop across a
resistor to the resistance of the resistor and the current at the resistor. The
Ohm's law equation can be used for any individual resistor in a series circuit.
When combining Ohm's law with some of the principles already discussed on this
page, a big idea emerges.
In
series circuits, the resistor with the greatest resistance has the greatest
voltage drop.
Since the current is everywhere the same within a series
circuit, the I value of ΔV = I • R is
the same in each of the resistors of a series circuit. So the voltage drop
(ΔV) will vary with varying resistance. Wherever the resistance is
greatest, the voltage drop will be greatest about that resistor. The Ohm's law equation
can be used to not only predict that resistor in a series circuit will have the
greatest voltage drop, it can also be used to calculate the actual voltage drop
values.
|
ΔV1 = I • R1 |
ΔV2 = I • R2 |
ΔV3 = I • R3 |
Mathematical
Analysis of Series Circuits
The above principles and formulae can be used to analyze a
series circuit and determine the values of the current at and electric
potential difference across each of the resistors in a series circuit. Their
use will be demonstrated by the mathematical analysis of the circuit shown
below. The goal is to use the formulae to determine the equivalent resistance
of the circuit (Req), the current at the battery (Itot),
and the voltage drops and current for each of the three resistors.
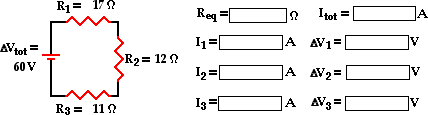
The analysis begins by using the resistance values for the
individual resistors in order to determine the equivalent resistance of the
circuit.
Req =
R1 + R2 + R3 = 17 Ω + 12 Ω + 11 Ω = 40 Ω
Now that the equivalent resistance is known, the current at
the battery can be determined using the Ohm's law equation. In using the Ohm's
law equation (ΔV = I • R) to determine the current in the circuit, it is important
to use the battery voltage for ΔV and the equivalent resistance for R. The
calculation is shown here:
Itot =
ΔVbattery / Req = (60 V) / (40 Ω) = 1.5 amp
The 1.5 amp value for current is the current at the battery
location. For a series circuit with no branching locations, the current is
everywhere the same. The current at the battery location is the same as the
current at each resistor location. Subsequently, the 1.5 amp is the value of I1,
I2, and I3.
Ibattery =
I1 = I2 = I3 = 1.5 amp
There are three values left to be determined - the voltage
drops across each of the individual resistors. Ohm's law is used once more to
determine the voltage drops for each resistor - it is simply the product of the
current at each resistor (calculated above as 1.5 amp) and the resistance of
each resistor (given in the problem statement). The calculations are shown
below.
|
ΔV1 = I1 • R1 ΔV1 = (1.5 A) • (17 Ω) ΔV1 = 25.5 V |
ΔV2 = I2 • R2 ΔV2 = (1.5 A) • (12 Ω) ΔV2 = 18 V |
ΔV3 = I3 • R3 ΔV3 = (1.5 A) • (11 Ω) ΔV3 = 16.5 V |
As a check of the accuracy of the mathematics performed, it
is wise to see if the calculated values satisfy the principle that the sum of
the voltage drops for each individual resistor is equal to the voltage rating
of the battery. In other words, is ΔVbattery = ΔV1 + ΔV2 + ΔV3 ?
Is ΔVbattery =
ΔV1 + ΔV2 + ΔV3 ?
Is 60 V = 25.5 V + 18 V + 16.5 V ?
Is 60 V = 60 V?
Yes!!
The mathematical analysis of this series circuit involved a
blend of concepts and equations. As is often the case in physics, the divorcing
of concepts from equations when embarking on the solution to a physics problem
is a dangerous act. Here, one must consider the concepts that the current is
everywhere the same and that the battery voltage is equivalent to the sum of
the voltage drops across each resistor in order to complete the mathematical
analysis. In the next part of Lesson 4, parallel
circuits will be analyzed using Ohm's law and parallel circuit concepts. We
will see that the approach of blending the concepts with the equations will be
equally important to that analysis.
Check Your Understanding
1. Use your understanding of equivalent resistance to
complete the following statements:
a. Two 3-Ω resistors placed in series would provide a
resistance that is equivalent to one _____-Ω resistor.
b. Three 3-Ω resistors placed in series would provide a
resistance that is equivalent to one _____-Ω resistor.
c. Three 5-Ω resistors placed in series would provide a
resistance that is equivalent to one _____-Ω resistor.
d. Three resistors with resistance values of 2-Ω , 4-Ω , and 6-Ω are placed in series.
These would provide a resistance that is equivalent to one _____-Ω
resistor.
e. Three resistors with resistance values of 5-Ω , 6-Ω , and 7-Ω are placed in series.
These would provide a resistance that is equivalent to one _____-Ω
resistor.
f. Three resistors with resistance values of 12-Ω,
3-Ω, and 21-Ω are placed in series. These would provide a resistance
that is equivalent to one _____-Ω resistor.
Use
the equation for the equivalent resistance of a series circuit:
Req =
R1 + R2 + R3 + ...
a.
Two 3-Ω resistors placed in series would provide a resistance which
is equivalent to one 6-Ω resistor.
b.
Three 3-Ω resistors placed in series would provide a resistance which
is equivalent to one 9-Ω resistor.
c.
Three 5-Ω resistors placed in series would provide a resistance which
is equivalent to one 15-Ω resistor.
d.
Three resistors with resistance values of 2-Ω , 4-Ω ,
and 6-Ω are placed in series. These would provide a resistance which
is equivalent to one 12-Ω resistor.
e.
Three resistors with resistance values of 5-Ω , 6-Ω ,
and 7-Ω are placed in series. These would provide a resistance which
is equivalent to one 18-Ω resistor.
f.
Three resistors with resistance values of 12-Ω , 3-Ω ,
and 21-Ω are placed in series. These would provide a resistance which
is equivalent to one 36-Ω resistor.
2. As the number of
resistors in a series circuit increases, the overall resistance __________
(increases, decreases, remains the same) and the current in the circuit
__________ (increases, decreases, remains the same).
As the number of
resistors in a series circuit increases, the overall resistance increases and the current in
the circuit decreases.
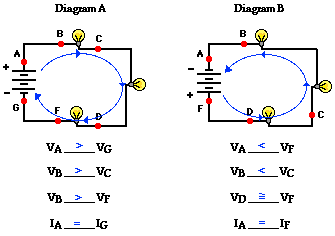
3. Consider the following two diagrams of series circuits.
For each diagram, use arrows to indicate the direction of the conventional
current. Then, make comparisons of the voltage and the current at the
designated points for each diagram.
The conventional current is directed through the external
circuit from the positive terminal to the negative terminal. The magnitude of
this current (I) is everywhere the same - thus the equal sign in the current
comparisons. As charge progresses in the direction of the conventional current,
there is a drop in electric potential every time it passes through a light
bulb. By the time the charge reaches the negative terminal, its electric
potential has dropped to zero volts. The closer a position is to the positive
terminal, the higher its potential; and conversely, the closer a position is to
the negative terminal, the lower its potential. If two locations are separated
by a mere wire (such as location D and F in Diagram B), then their potential is
approximately the same since there is relatively little resistance in a wire.
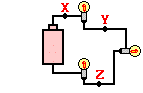4. Three identical light bulbs are connected to a D-cell as shown at the
right. Which one of the following statements is true?
a.
All three bulbs will have the same brightness.
b. The bulb between X and Y will be the brightest.
c. The bulb between Y and Z will be the brightest.
d. The bulb between Z and the battery will be the brightest.
Answer: A
The current in a
series circuit is the same at each resistor present in the circuit. Since each
light bulb has the same resistance ("identical bulbs") and the same
current, they will have the same power output (P = I2R as discussed
in the previous Lesson ). Thus, they will shine
with the same brightness.
5. Three identical light bulbs are connected to a battery as shown at
the right. Which adjustments could be made to the circuit that would increase
the current being measured at X? List all that apply.
a.
Increase the resistance of one of the bulbs.
b. Increase the resistance of two of the bulbs.
c. Decrease the resistance of two of the bulbs.
d. Increase the voltage of the battery.
e. Decrease the voltage of the battery.
f. Remove one of the bulbs.
Answer: C, D, and F
The current at
location X is the same as the current at the battery location. To increase the
value of the current at the battery, it would be necessary to increase the
battery voltage (choice D) or to decrease the equivalent resistance. Since the
equivalent resistance is the sum of the resistance of the individual resistors,
any decrease of resistance or removal of a resistor will lead to a decrease in
the equivalent resistance.
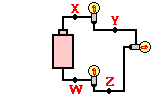6. Three identical light bulbs are connected to a battery as shown at
the right. W, X,Y and Z represent locations along
the circuit. Which one of the following statements is true?
a.
The potential difference between X and Y is greater than that between Y and Z.
b. The potential difference between X and Y is greater than
that between Y and W.
c. The potential difference between Y and Z is greater than
that between Y and W.
d. The potential difference between X and Z is greater than
that between Z and W.
e. The potential difference between X and W is greater than
that across the battery.
f. The potential difference between X and Y is greater than
that between Z and W.
Answer: D
In a series
circuit, the current is the same at each resistor. If the light bulbs are
identical, then the resistance is the same for each resistor. The voltage drop
(I•R) will be the same for each resistor since the current at and the
resistance of each resistor is the same. Thus the electric potential difference
across any one of the bulbs will be the same as that across any one of the
other bulbs. And the electric potential difference across two or three bulbs
will be greater than that across one bulb. The voltage boost in the battery
will be equal to the sum of the voltage drops across all three resistors. Only
choice D meets these criteria.
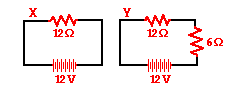7. Compare circuit X and Y below. Each is powered by a 12-volt battery.
The voltage drop across the 12-ohm resistor in circuit Y is ____ the voltage
drop across the single resistor in X.
a. smaller than
b. larger than
c. the same as
Answer: A
The voltage
gained at the battery is equal to the accumulative voltage drop when passing
through the external circuit. In circuit X, the voltage drop across the single
resistor must be 12 V. In circuit Y, the voltage drop across the 12 ohm
resistor must be less than 12 V since there will be an additional voltage drop
in the 6 ohm resistor. In fact, one might reason that the two voltage drops in
circuit Y will be 8 volts and 4 volts respectively, to add to a total voltage
drop of 12 volts.
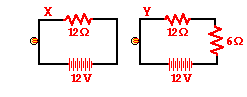8. A 12-V battery, a 12-ohm resistor and a light bulb are connected as
shown in circuit X below. A 6-ohm resistor is added to the 12-ohm resistor and
bulb to create circuit Y as shown. The bulb will appear ____.
a. dimmer in circuit X
b. dimmer in
circuit Y
c. the same
brightness in both circuits
Answer: B
The brightness of
a light bulb is related to the power output of the bulb, which can be computed
as the I • ΔV (see previous Lesson).
Since Circuit Y contains an additional resistor, its equivalent resistance is
greater than that of Circuit X. As such, Circuit X has a greater current than
that of Circuit Y. The voltage impressed across each circuit is the same - 12
volts (the battery voltage). This 12 volts of electric potential difference is
divided among the various circuit elements. There are two resistors and a light
bulb in Circuit Y and only one resistor and a light bulb in Circuit X. And so
the light bulb of Circuit X will have a greater ΔV than the light bulb of
Circuit Y. And so it is reasonable to conclude that the light bulb of Circuit X
has the greatest power - that is, the greatest I • ΔV product. And since
the bulb brightness will depend upon this power value, one must conclude that
the bulb will appear brighter in Circuit X and dimmer in Circuit Y.
close
9. Three resistors are connected in series. If placed in a
circuit with a 12-volt power supply. Determine the equivalent resistance, the
total circuit current, and the voltage drop across and current at each resistor.
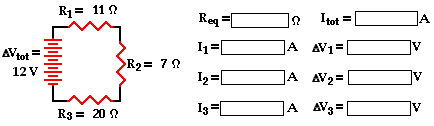
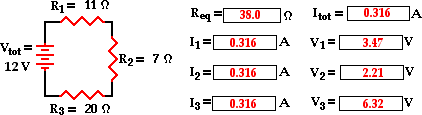
The analysis begins by using the resistance values for the
individual resistors in order to determine the equivalent resistance of the
circuit.
Req = R1 + R2 +
R3 = 11 Ω + 7 Ω +
20 Ω = 38 Ω
Now that the equivalent resistance is known, the current through
the battery can be determined using Ohm's law equation. In using the Ohm's law
equation (ΔV = I • R) to determine the current in the circuit, it is important
to use the battery voltage for ΔV and the equivalent resistance for
R. The calculation is shown here:
Itot = ΔVbattery / Req =
(12 V) / (38 Ω) = 0.31579 Amp
The 0.316 Amp value for current is the current at the battery
location. For a series circuit with no branching locations, the current is
everywhere the same. The current at the battery location is the same as the
current at each resistor location. Subsequently, the 0.316 Amp (rounded) is the
value of I1, I2, and I3.
Ibattery = I1 = I2 =
I3 = 0.316 Amp (rounded)
There are three values left to be determined - the voltage drops
across each of the individual resistors. Ohm's law is used once more to
determine the voltage drops for each resistor - it is simply the product of the
current at each resistor (calculated above as 0.31579 Amp) and the resistance
of each resistor (given in the problem statement). The calculations are shown
below.
|
ΔV1 = I1 •
R1 ΔV1 =
(0.31579 A) • (11 Ω) ΔV1 = 3.47 V |
ΔV2 =
(0.31579 A) • (7 Ω) ΔV2 = 2.21 V |
ΔV3 = I3 •
R3 ΔV3 =
(0.31579 A) • (20 Ω) ΔV3 = 6.32 V |
As a check of the accuracy of the mathematics performed, it is
wise to see if the calculated values satisfy the principle that the sum of the
voltage drops for each individual resistor is equal to the voltage rating of
the battery. In other words, is ΔVbattery = ΔV1 + ΔV2 + ΔV3 ?
Is ΔVbattery = ΔV1 + ΔV2 + ΔV3 ?
Is 12 V = 3.47 V + 2.21 V + 6.32 V ?
Is 12 V = 12 V?
Yes!!