Mathematics of Circular Motion
There are three mathematical quantities that
will be of primary interest to us as we analyze the motion of objects in circles. These three
quantities are speed, acceleration and force. The speed of an object moving in
a circle is given by the following equation.

The acceleration of an object moving in a
circle can be determined by either two of the following equations.

The equation on the right (above) is derived from the
equation on the left by the substitution of the expression for speed.
The net force (Fnet) acting upon an object moving in circular motion is directed inwards.
While there may by more than one force acting upon the object, the vector sum
of all of them should add up to the net force. In general, the inward force is
larger than the outward force (if any) such that the outward force cancels and
the unbalanced force is in the direction of the center of the circle. The net force is related
to the acceleration of the object (as is always the case) and is thus given by
the following three equations:
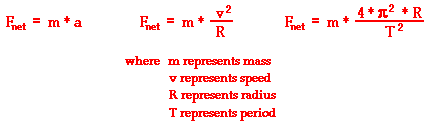
The equations in the middle (above) and on the right (above)
are derived from the equation on the left by the substitution of the
expressions for acceleration.
This set of circular motion equations can be used in two ways:
· as a "recipe"
for algebraic problem-solving in order
to solve for an unknown quantity.
· as a guide
to thinking about how an
alteration in one quantity would affect a second quantity.
These two ways are illustrated below.
Equations
as a Guide to Thinking
An equation expresses a mathematical relationship between the
quantities present in that equation. For instance, the equation for Newton's
second law identifies how acceleration is related to the net force and the mass
of an object.
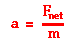
The relationship expressed by the equation is that the
acceleration of an object is directly proportional to the net force acting upon
it. In other words, the bigger the net force value is, the bigger that the
acceleration value will be. As net force increases, the acceleration increases.
In fact, if the net force were increased by a factor of 2, the equation would
predict that the acceleration would increase by a factor of 2. Similarly, if
the net force were decreased by a factor of 2, the equation would predict that
the acceleration would decrease by a factor of 2.
Newton's second law equation also reveals the relationship
between acceleration and mass. According to the equation, the acceleration of
an object is inversely proportional to mass of the object. In other words, the
bigger the mass value is, the smaller that the acceleration value will be. As
mass increases, the acceleration decreases. In fact, if the mass were increased
by a factor of 2, the equation would predict that the acceleration would
decrease by a factor of 2. Similarly, if the mass were decreased by a factor of
2, the equation would predict that the acceleration would increase by a factor
of 2.
As mentioned previously, equations allow for predictions to
be made about the affect of an alteration of one quantity on a second quantity. Since the
Newton's second law equation shows three quantities, each raised to the first
power, the predictive ability of the equation is rather straightforward. The
predictive ability of an equation becomes more complicated when one of the
quantities included in the equation is raised to a power. For instance,
consider the following equation relating the net force (Fnet) to the speed (v) of an object moving in uniform circular motion.
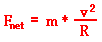
This equation shows that the net force required for an object
to move in a circle is directly proportional to the square of the speed of the
object. For a constant mass and radius, the Fnet is proportional to thespeed2.
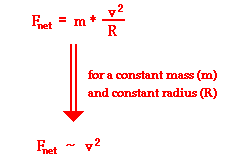
The factor by which the net force is altered is the square of
the factor by which the speed is altered. Subsequently, if the speed of the
object is doubled, the net force required for that object's circular motion is
quadrupled. And if the speed of the object is halved (decreased by a factor of
2), the net force required is decreased by a factor of 4.
Equations as a Recipe for Problem-Solving
The mathematical equations presented
above for the motion of objects in circles can be used to solve circular
motion problems in which an unknown quantity must be determined. The process of
solving a circular motion problem is much like any other problem in physics
class. The process involves a careful reading of the problem, the
identification of the known and required information in variable form, the
selection of the relevant equation(s), substitution of known values into the
equation, and finally algebraic manipulation of the equation to determine the
answer. Consider the application of this process to the following two circular
motion problems.
|
Sample Problem #1 A 900-kg
car moving at 10 m/s takes a turn around a circle with a radius of 25.0 m.
Determine the acceleration and the net force acting upon the car. |
The solution of this problem begins with the identification
of the known and requested information.
|
Known Information: m
= 900 kg v = 10.0
m/s R = 25.0 m |
Requested Information: a = ???? Fnet = ???? |
To determine the acceleration of the car, use the equation a
= v2 / R. The solution is as
follows:
a = v2 /
R
a = (10.0 m/s)2 / (25.0 m)
a = (100 m2/s2) / (25.0 m)
a = 4 m/s2
To determine the net force acting upon the car, use the
equation Fnet = m•a. The solution is
as follows.
Fnet = m • a
Fnet = (900 kg)
• (4 m/s2)
Fnet = 3600 N
|
Sample Problem #2 A 95-kg
halfback makes a turn on the football field. The halfback sweeps out a path
that is a portion of a circle with a radius of 12-meters. The halfback makes
a quarter of a turn around the circle in 2.1 seconds. Determine the speed,
acceleration and net force acting upon the halfback. |
The solution of this problem begins with the identification
of the known and requested information.
|
Known Information: m
= 95.0 kg R = 12.0 m Traveled 1/4-th of the circumference in
2.1 s |
Requested Information: v = ???? a = ???? Fnet = ???? |
To determine the speed of the halfback, use the equation v =
d / t where the d is one-fourth of the circumference and the time is 2.1 s. The
solution is as follows:
v = d / t
v = (0.25 • 2 • pi • R) / t
v = (0.25 • 2 • 3.14 • 12.0 m) / (2.1 s)
v = 8.97 m/s
To determine the acceleration of the halfback, use the
equation a = v2 / R. The solution is as follows:
a = v2 /
R
a = (8.97 m/s)2 / (12.0 m)
a = (80.5 m2/s2) / (12.0 m)
a = 6.71 m/s2
To determine the net force acting upon the halfback, use the
equation Fnet = m•a. The solution is
as follows.
Fnet = m*a
Fnet = (95.0
kg)*(6.71 m/s2)
Fnet = 637 N
In Lesson 2 of this unit, circular motion principles and the
above mathematical equations will be combined to explain and analyze a
variety of real-world motion scenarios including amusement
park rides and circular-type motions in athletics.
Check Your Understanding
1. Anna Litical is practicing a centripetal force
demonstration at home. She fills a bucket with water, ties it to a strong rope,
and spins it in a circle. Anna spins the bucket when it is half-full of water
and when it is quarter-full of water. In which case is more force required to
spin the bucket in a circle? Explain using an equation as a "guide to
thinking."

It will
require more
force to
accelerate a full bucket of water compared to a half-full bucket. According to
the equation Fnet =
m•v2 / R, force and mass are directly proportional. So the
greater the mass, the greater the force.
2. A Lincoln Continental and a Yugo are making a turn. The
Lincoln is four times more massive than the Yugo. If they make the turn at the
same speed, then how do the centripetal forces acting upon the two cars
compare. Explain.

The centripetal
force on the Continental is four times greater than that of a Yugo. According
to the equation Fnet=(m•v2) / R, force and mass are directly
proportional. So 4 times the mass means 4 times the force.
3. The Cajun Cliff-hanger at Great America is a ride in which
occupants line the perimeter of a cylinder and spin in a circle at a high rate
of turning. When the cylinder begins spinning very rapidly, the floor is
removed from under the riders' feet. What affect does a doubling in speed have
upon the centripetal force? Explain.

Doubling the
speed of the ride will cause the force to be four times greater than the original
force. According to the equation Fnet=(m•v2) / R, force and speed2 are
directly proportional. So 2X the speed means 4X the force (that's from 22).
4. Determine the centripetal force acting upon a 40-kg child
who makes 10 revolutions around the Cliffhanger in 29.3 seconds. The radius of the barrel
is 2.90 meters.

Answer: Fnet = 533 N
Given: m = 40 kg; R = 2.90 m; T = 2.93 s (since 10 cycles takes
29.3 s).
First, find speed using
speed=(2 • pi • R) / T = 6.22
m/s.
Then find the acceleration using
a = v2 /
R = = (6.22 m/s)2 / (2.90 m) = 13.3
m/s/s
Now use Fnet = m • a to find
that Fnet = 533 N.