The Forbidden F-Word

When the subject of circular motion is discussed, it is not uncommon to hear mention of the word centrifugal. Centrifugal, not to be confused with centripetal, means away from the center or outward. The use of or at least the familiarity with this word centrifugal, combined with the common sensation of an outward lean when experiencing circular motion, often creates or reinforces a common student misconception. The common misconception, believed by many physics students, is the notion that objects in circular motion are experiencing an outward force. "After all," a well-meaning student may think, "I can recall vividly the sensation of being thrown outward away from the center of the circle on that roller coaster ride. Therefore, circular motion must be characterized by an outward force." This misconception is often fervently adhered to despite the clear presentation by a textbook or teacher of an inward force requirement. As discussed previously in Lesson 1, the motion of an object in a circle requires that there be an inward net force - the centripetal force requirement. There is an inward-directed acceleration that demands an inward force. Without this inward force, an object would maintain a straight-line motion tangent to the perimeter of the circle. Without this inward or centripetal force, circular motion would be impossible.

Evaluate Your Own Experiences

So why then is this student misconception of an outward or centrifugal force so prevalent and so stubbornly adhered to? Perhaps like all misconceptions, the notion of a centrifugal force as lodged in a person's head has a particularly lengthy history. Part of that history is certainly attributable to the experience of a circular motion - either as a passenger or driver in an automobile or perhaps on an amusement park ride. Even learned physics types would admit that circular motion leaves the moving person with the sensation of being thrown outward from the center of the circle. But before drawing hasty conclusions, ask yourself three probing questions:

· Does the sensation of being thrown outward from the center of a circle mean that there was definitely an outward force?

· If there is such an outward force on my body as I make a left-hand turn in an automobile, then what physical object is supplying the outward push or pull?

· And finally, could that sensation be explained in other ways that are more consistent with our growing understanding of Newton's laws?

If you can answer the first of these questions with "No" then you have a chance. But if you quickly conclude that the outward feeling means there is an outward force, then you at least must admit that your conclusion is contrary to all that has been discussed in Lesson 1 and that you don't believe that Newton's laws accurately describe circular motion. The sensation of being thrown outward is attributable to the idea of inertia, rather than the idea of force. When making that left-hand turn in the car, your tendency to be thrown rightward across the seat (that would be outward or away from the center of the circle) was not due to a force. It was due to your tendency to travel in a straight line while the car seat was making its turn. In fact, you were not thrown rightward at all; you moved in a perfectly straight line. If an airborne camera had collected the motion on film from above and we could watch the instant replay, then it would be a no-brainer - the car turned left and your body kept going straight. Finally, your body hits the door on the right side of the car and the door provides an inward push on your body to cause your body to begin moving in circular motion. But until hitting the door, your body's tendency was to follow its inertial path.

The Law of Inertia Explains the Feeling of an Outward Force

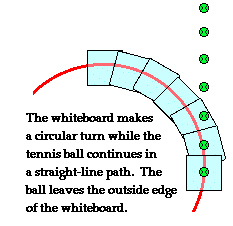
A common physics demonstration involves using a flat whiteboard with a tennis ball on top of it. The whiteboard is carried along in a straight-line path; the ball rest on top of the whiteboard and follows the same straight-line path. Then suddenly, the board is turned leftward to begin a circular motion; yet the ball keeps moving straight. Ultimately, the ball rolls off the right-edge of the board and continues in its straight-line inertial path. Without an unbalanced force on the ball, the ball continues in its original motion. The whiteboard merely moved out from under the ball as it makes its turn. If you could watch carefully, then you could view the ball's path from the perspective of an airborne camera. It's a no brainer - the ball moves straight while the whiteboard turns. And finally, the ball travels off the "outside edge" of the whiteboard. Relative to the circular motion of the whiteboard, the ball moves away from the center of the circle. But explaining the motion of the ball does not require that we imagine or dream up the existence of an outward or centrifugal force. The motion of the ball is explained by the tendency of an object in motion to continue in motion in the same direction. INERTIA!

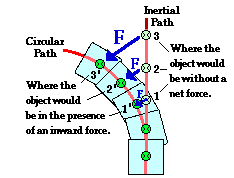Now suppose that a block is attached to the top of the whiteboard on the "outside" of the ball with such an orientation that it would apply an inward force upon the ball. When the whiteboard is turned, the block would turn as well and supply the centripetal force required to move the ball in a circle. Without the block, the ball would have moved along the straight-line path, moving to position 1 after say 0.1 seconds, then to position 2 after 0.2 seconds, then to position 3 after 0.3 seconds, and so on. But with the block supplying an inward force, the ball moves inward towards the center of the circle relative to its straight-line path. Instead of being at position 1, the ball is closer to the center at position 1'. And instead of being at position 2 after 0.2 seconds, the ball is forced inwards towards position 2'. And instead of being at position 3 after 0.3 seconds, the ball is forced inwards towards position 3'. The inward net force accelerates the ball inward, causing it to deviate from its straight-line path that is directed tangent to the circle.

If you were the tennis ball in the first example above, then you might feel like you were being pushed outwards. After all, you would travel through the outside door of the whiteboard. Yet it is clear from the diagram and the discussion that you are not deviating from any straight-line path. It is merely that the whiteboard is moving inward relative to your path and you are moving outward relative to the whiteboard's path. But this sensation of relative motion does not give reason for supposing that an outward force exists. This notion of an outward force is merely fictitious. Newton's law of inertia - "an object in motion continues in motion with the same speed and in the same direction unless acted upon an unbalanced force" - provides a more reasonable explanation for the sensations experienced by those who are in circular motion. A centrifugal or outward net force simply does not exist. No physical object could ever be identified that was pushing you outwards. And if there was a physical object pushing or pulling you outwards (e.g., in the rightwards direction when taking a left-hand turn), then you certainly would not turn in the circle that you are turning in.

An object moving n circular motion is at all times moving tangent to the circle; the velocity vector for the object is directed tangentially. To make the circular motion, there must be a net or unbalanced force directed towards the center of the circle in order to deviate the object from its otherwise tangential path. This path is an inward force - a centripetal force. That is spelled c-e-n-t-r-i-p-e-t-a-l, with a "p." The other word - centrifugal, with an "f" - will be considered our forbidden F-word. Simply don't use it and please don't believe in it.

Pilots at an air show perform a loop-the-loop. The pilots are oriented upside down at the top of the loop. They do not fall out due to inertia and centripetal force. Their natural tendency is to continue tangent to the curved path, but the gravitational force (and perhaps some normal force) pushes them center-ward. This centripetal force allows for circular motion.