The Logic behind Momentum Conservation
Consider a collision between two objects - object 1 and
object 2. For such a collision, the forces acting between the two objects are
equal in magnitude and opposite in direction (Newton's
third law). This statement can be expressed in equation
form as follows.
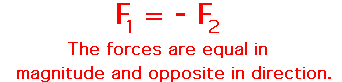
The forces act between the two objects for a given amount of
time. In some cases, the time is long; in other cases the time is short.
Regardless of how long the time is, it can be said that the time that the force
acts upon object 1 is equal to the time that the force acts upon object 2. This
is merely logical. Forces result from interactions (or contact) between two
objects. If object 1 contacts object 2 for 0.050 seconds, then object 2 must be
contacting object 1 for the same amount of time (0.050 seconds). As an
equation, this can be stated as

Since the forces between the two objects are equal in
magnitude and opposite in direction, and since the times for which these forces
act are equal in magnitude, it follows that the impulses experienced
by the two objects are also equal in magnitude and opposite in direction. As an
equation, this can be stated as
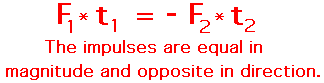
But the impulse experienced by an object is equal to
the change in momentum of that object (the
impulse-momentum change theorem). Thus, since each object
experiences equal and opposite impulses, it follows logically that they must
also experience equal and opposite momentum changes. As an equation, this can
be stated as
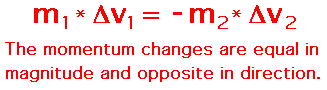
 The Law of
Momentum Conservation
The Law of
Momentum Conservation
The above equation is one statement of the law of momentum
conservation. In a collision, the momentum change of object 1 is equal to and
opposite of the momentum change of object 2. That is, the momentum lost by
object 1 is equal to the momentum gained by object 2. In most collisions
between two objects, one object slows down and loses momentum while the other
object speeds up and gains momentum. If object 1 loses 75 units of momentum,
then object 2 gains 75 units of momentum. Yet, the total momentum of the two
objects (object 1 plus object 2) is the same before the collision as it is
after the collision. The total momentum of the system (the
collection of two objects) is conserved.
A useful analogy for understanding momentum
conservation involves a money transaction between two people. Let's refer to
the two people as Jack and Jill. Suppose that we were to check the pockets of
Jack and Jill before and after the money transaction in order to determine the
amount of money that each possesses. Prior to the transaction, Jack possesses
$100 and Jill possesses $100. The total amount of money of the two people
before the transaction is $200. During the transaction, Jack pays Jill $50 for
the given item being bought. There is a transfer of $50 from Jack's pocket to Jill's
pocket. Jack has lost $50 and Jill has gained $50. The money lost by Jack is
equal to the money gained by Jill. After the transaction, Jack now has $50 in
his pocket and Jill has $150 in her pocket. Yet, the total amount of money of
the two people after the transaction is $200. The total amount of money (Jack's
money plus Jill's money) before the transaction is equal to the total amount of
money after the transaction. It could be said that the total amount of money of the system (the
collection of two people) is conserved. It is the same before as it is after
the transaction.
A useful means of depicting the transfer and the conservation
of money between Jack and Jill is by means of a table.
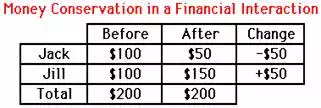
The table shows the amount of money possessed by the two
individuals before and after the interaction. It also shows the total amount of
money before and after the interaction. Note that the total amount of money
($200) is the same before and after the interaction - it is conserved. Finally,
the table shows the change in the amount of money possessed by the two
individuals. Note that the change in Jack's money account (-$50) is equal to
and opposite of the change in Jill's money account (+$50).
For any collision occurring in an isolated
system, momentum is conserved. The total amount of
momentum of the collection of objects in the system is the same before the
collision as after the collision. A common physics lab involves the dropping of
a brick upon a cart in motion.
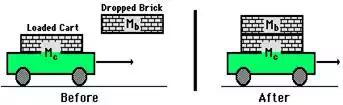
The dropped brick is at rest and begins with zero momentum.
The loaded cart (a cart with a brick on it) is in motion with considerable
momentum. The actual momentum of the loaded cart can be determined using the
velocity (often determined by a ticker tape analysis) and the mass. The total
amount of momentum is the sum of the dropped brick's momentum (0 units) and the
loaded cart's momentum. After the collision, the momenta of the two separate
objects (dropped brick and loaded cart) can be determined from their measured
mass and their velocity (often found from a ticker tape analysis). If momentum
is conserved during the collision, then the sum of the dropped brick's and
loaded cart's momentum after the collision should be the same as before the
collision. The momentum lost by the loaded cart should equal (or approximately
equal) the momentum gained by the dropped brick. Momentum data for the interaction
between the dropped brick and the loaded cart could be depicted in a table
similar to the money table above.
|
|
Before Collision |
After Collision Momentum |
Change in Momentum |
|
Dropped Brick |
0 units |
14 units |
+14 units |
|
Loaded Cart |
45 units |
31 units |
-14 units |
|
Total |
45 units |
45 units |
|
Note that the loaded cart lost 14 units of momentum and the
dropped brick gained 14 units of momentum. Note also that the total momentum of
the system (45 units) was the same before the collision as it was after the
collision.
Collisions commonly occur in contact sports (such as
football) and racket and bat sports (such as baseball, golf, tennis, etc.).
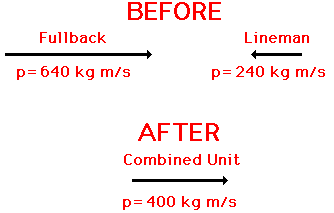
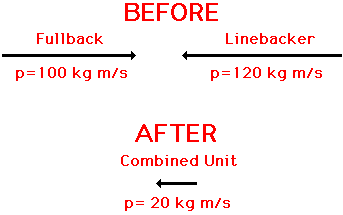
Consider a collision in football between a fullback and a linebacker during a goal-line
stand. The fullback plunges across the goal line and
collides inmidair with the linebacker. The linebacker and
fullback hold each other and travel together after the collision. The fullback
possesses a momentum of 100 kg*m/s, East before the collision and the linebacker possesses a momentum of 120 kg*m/s, West
before the collision. The total momentum of the system before the collision is
20 kg*m/s, West (review the section on adding vectors if
necessary). Therefore, the total momentum of the system after the collision
must also be 20 kg*m/s, West. The fullback and the linebacker move
together as a single unit after the collision with a combined momentum of 20
kg*m/s. Momentum is conserved in the collision. A vector
diagram can be used to represent this principle of momentum conservation; such a
diagram uses an arrow to represent the magnitude and direction of the momentum
vector for the individual objects before the collision and the combined
momentum after the collision.
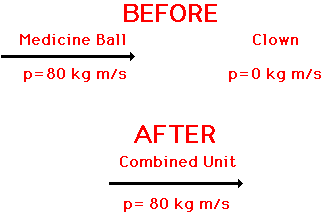
Now suppose that a medicine ball is thrown to a clown who is
at rest upon the ice; the clown catches the medicine ball and glides together
with the ball across the ice. The momentum of the medicine ball is 80 kg*m/s
before the collision. The momentum of the clown is 0 m/s before the collision.
The total momentum of the system before the collision is 80 kg*m/s. Therefore,
the total momentum of the system after the collision must also be 80 kg*m/s.
The clown and the medicine ball move together as a single unit after the
collision with a combined momentum of 80 kg*m/s. Momentum is conserved in the
collision.
Momentum is conserved for any interaction between two objects
occurring in an isolated system. This conservation of momentum can be observed
by a total system momentum analysis or by a momentum change analysis. Useful
means of representing such analyses include a momentum table and a vector
diagram. Later in Lesson 2, we will use the momentum conservation principle to
solve problems in which the after-collision velocity of objects is predicted.
Check Your Understanding
Express your understanding of the concept and mathematics of
momentum by answering the following questions. Click on the button to view the
answers.
1. When fighting fires, a firefighter must use great caution
to hold a hose that emits large amounts of water at high speeds. Why would such
a task be difficult?

The hose is
pushing lots of water (large mass) forward at a high speed. This means the
water has a large forward momentum. In turn, the hose must have an equally
large backwards momentum, making it difficult for the firefighters to manage.
close
2. A large truck and a Volkswagen have a head-on collision.
a. Which vehicle experiences the greatest force of impact?
b. Which vehicle experiences the greatest impulse?
c. Which vehicle experiences the greatest momentum change?
d. Which vehicle experiences the greatest acceleration?

a, b, c: the same for each.
Both the Volkswagon and the
large truck encounter the same force, the same impulse, and the same momentum
change (for reasons discussed in this lesson).
d: Acceleration is greatest for the Volkswagon. While the two vehicles
experience the same force, the acceleration is greatest for the Volkswagon due to its smaller mass. If you find this
hard to believe, then be sure to read the next question and its accompanying
explanation.
close
3. Miles Tugo and
Ben Travlun are riding in a bus at highway
speed on a nice summer day when an unlucky bug splatters onto the windshield.
Miles and Ben begin discussing the physics of the situation. Miles suggests
that the momentum change of the bug is much greater than that of the bus. After
all, argues Miles, there was no noticeable change in the speed of the bus
compared to the obvious change in the speed of the bug. Ben disagrees entirely,
arguing that that both bug and bus encounter the same force, momentum change,
and impulse. Who do you agree with? Support your answer.

Ben Travlun is
correct.
The bug and bus
experience the same force, the same impulse, and the same momentum change (as
discussed in this lesson). This is contrary to the popular (though false)
belief which resembles Miles' statement. The bug has less mass and therefore
more acceleration; occupants of the very massive bus do not feel the extremely
small acceleration. Furthermore, the bug is composed of a less hardy material
and thus splatters all over the windshield. Yet the greater "splatterability" of the bug and the greater
acceleration do not mean the bug has a greater force, impulse, or momentum
change.
4. If a ball is projected upward from the ground with ten
units of momentum, what is the momentum of recoil of the Earth? ____________ Do
we feel this? Explain.

The earth
recoils with 10 units of momentum. This is not felt by Earth's occupants. Since the mass of the
Earth is extremely large, the recoil velocity of the Earth is extremely small
and therefore not felt.
5. If a 5-kg bowling ball is projected upward with a velocity
of 2.0 m/s, then what is the recoil velocity of the Earth (mass = 6.0 x 1024 kg).

Since the ball has an upward momentum of 10 kg*m/s, the Earth must have a downward
momentum of 10 kg*m/s. To find the velocity of the Earth, use the momentum
equation, p = m*v. This equation rearranges to v=p/m. By substituting into this
equation,
v = (10 kg*m/s)/(6*1024 kg)
v = 1.67*10-24 m/s (downward)
Another way to write the velocity of the earth is to write it as
0.00000000000000000000000167
m/s
6. A 120 kg lineman moving west at 2 m/s
tackles an 80 kg football fullback moving east at 8 m/s. After the collision,
both players move east at 2 m/s. Draw a vector diagram in which the before- and
after-collision momenta of each player is represented by a momentum vector.
Label the magnitude of each momentum vector.
See answer below.
7. In an effort to exact the most severe capital punishment
upon a rather unpopular prisoner, the execution team at the Dark Ages
Penitentiary search for a bullet that is ten times as massive as the rifle
itself. What type of individual would want to fire a rifle that holds a bullet
that is ten times more massive than the rifle? Explain.

Someone
who doesn't know much physics. In such a
situation as this, the target would be a safer place to stand than the rifle.
The rifle would have a recoil velocity that is ten times larger than the
bullet's velocity. This would produce the effect of "the rifle actually
being the bullet."
8. A baseball player holds a bat loosely and bunts a ball.
Express your understanding of momentum conservation by filling in the tables
below.
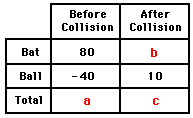

a: +40 (add the momentum of the
bat and the ball)
c: +40 (the total momentum is the
same after as it is before the collision)
b: 30 (the bat must have 30 units of momentum in order for the total
to be +40)
9. A Tomahawk cruise missile is launched from the barrel of a
mobile missile launcher. Neglect friction. Express your understanding of
momentum conservation by filling in the tables below.
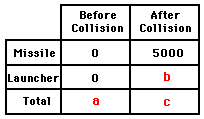

a: 0 (add the momentum of the
missile and the launcher)
c: 0 (the total momentum is the
same after as it is before the collision)
b: -5000 (the launcher must have -5000 units of momentum in order for the
total to be +0)