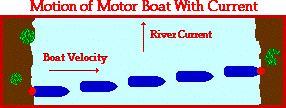
Analysis of a Riverboat's Motion
The effect of the wind upon the plane is similar to the
effect of the river current upon the motorboat. If a motorboat were to head
straight across a river (that is, if the boat were to point its bow straight
towards the other side), it would not reach the shore directly across from its
starting point. The river current influences the motion of the boat and carries
it downstream. The motorboat may be moving with a velocity of 4 m/s directly
across the river, yet the resultant velocity of the boat will be greater than 4
m/s and at an angle in the downstream direction. While the speedometer of the
boat may read 4 m/s, its speed with respect to an observer on the shore will be
greater than 4 m/s.
The resultant velocity of the motorboat can be
determined in the same manner as was done for the plane. The resultant velocity
of the boat is the vector sum of the boat velocity and the river velocity.
Since the boat heads straight across the river and since the current is always
directed straight downstream, the two vectors are at right angles to each
other. Thus, the Pythagorean theorem can be used to determine the resultant velocity.
Suppose that the river was moving with a velocity of 3 m/s, North and the
motorboat was moving with a velocity of 4 m/s, East.
What would be the resultant velocity of the motorboat (i.e., the velocity
relative to an observer on the shore)? The magnitude of the resultant can be
found as follows:
(4.0 m/s)2 + (3.0 m/s)2 = R2
16 m2/s2 + 9 m2/s2 = R2
25 m2/s2 = R2
SQRT (25 m2/s2) = R
5.0 m/s = R
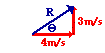The direction of the resultant is the counterclockwise angle
of rotation that the resultant vector makes with due East. This angle can be
determined using a trigonometric function as shown below.
tan (theta) = (opposite/adjacent)
tan (theta) = (3/4)
theta = invtan (3/4)
theta = 36.9
degrees
Given a boat velocity of 4 m/s, East and a river velocity of
3 m/s, North, the resultant velocity of the boat
will be 5 m/s at 36.9 degrees.
Motorboat problems such as these are typically accompanied by
three separate questions:
a. What is the
resultant velocity (both magnitude and direction) of the boat?
b. If the width
of the river is X meters wide, then how much time does
it take the boat to travel shore to shore?
c. What
distance downstream does the boat reach the opposite shore?
The first of these three questions was answered above; the
resultant velocity of the boat can be determined using the Pythagorean theorem (magnitude) and a trigonometric function
(direction). The second and third of these questions can be answered using the average speed
equation (and a lot of logic).
ave. speed =
distance/time
Consider the following example.
The solution to the first question has already been shown in
the above discussion. The resultant velocity of the boat is 5 m/s
at 36.9 degrees. We will start in on the second question.
The river is 80-meters wide. That is, the distance from shore
to shore as measured straight across the river is 80 meters. The time to cross
this 80-meter wide river can be determined by rearranging and substituting into
the average speed equation.
time =
distance /(ave. speed)
The distance of 80 m can be substituted into the numerator.
But what about the denominator? What value should be used for average speed?
Should 3 m/s (the current velocity), 4 m/s (the boat velocity), or 5 m/s (the
resultant velocity) be used as the average speed value for covering the 80
meters? With what average speed is the boat traversing the 80 meter wide river?
Most students want to use the resultant velocity in the equation since that is
the actual velocity of the boat with respect to the shore. Yet the value of 5
m/s is the speed at which the boat covers the diagonal dimension of the river.
And the diagonal distance across the river is not known in this case. If one
knew the distance C in the diagram below, then the average speed
C could be used to calculate the time to reach the opposite shore.
Similarly, if one knew the distance B in the diagram
below, then the average speed B could be used to calculate the time to reach the
opposite shore. And finally, if one knew the distance A in the diagram below,
then the average speed A could be usedto calculate the time to reach the opposite shore.
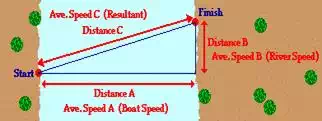
In our problem, the 80 m corresponds to the distance A, and
so the average speed of 4 m/s (average speed in the direction straight across
the river) should be substituted into the equation to determine the time.
time = (80 m)/(4 m/s) = 20 s
It requires 20 s for the boat to travel across the river.
During this 20 s of crossing the river, the boat also drifts downstream. Part c of the
problem asks "What distance downstream does the boat reach the opposite
shore?" The same equation must be used to calculate this downstream
distance. And once more, the question arises, which one
of the three average speed values must be used in the equation to calculate the
distance downstream? The distance downstream corresponds to Distance B on the above
diagram. The speed at which the boat covers this distance corresponds to Average Speed
B on the diagram above (i.e., the speed at which the current moves - 3
m/s). And so the average speed of 3 m/s (average speed in the downstream
direction) should be substituted into the equation to determine the distance.
distance = ave. speed * time = (3 m/s) * (20 s)
distance = 60 m
The boat is carried 60 meters downstream during the 20
seconds it takes to cross the river.
The mathematics of the above problem is no more difficult
than dividing or multiplying two numerical quantities by each other. The
mathematics is easy! The difficulty of the problem is conceptual in nature; the
difficulty lies in deciding which numbers to use in the equations. That
decision emerges from one's conceptual understanding (or unfortunately, one's
misunderstanding) of the complex motion that is occurring. The motion of the
riverboat can be divided into two simultaneous parts - a motion in the direction
straight across the river and a motion in the downstream direction. These two
parts (or components) of the motion occur simultaneously for the same time
duration (which was 20 seconds in the above problem). The decision as to which
velocity value or distance value to use in the equation must be consistent with
the diagram above. The boat's motor is what carries the boat across
the river the Distance A; and so any calculation involving the Distance A must involve the
speed value labeled as Speed A (the boat speed
relative to the water). Similarly, it is the current of the river that carries
the boat downstream for the Distance B; and so any
calculation involving the Distance B must involve the
speed value labeled asSpeed B (the river speed).
Together, these two parts (or components) add up to give the resulting motion
of the boat. That is, the across-the-river component of displacement adds to
the downstream displacement to equal the resulting displacement. And likewise,
the boat velocity (across the river) adds to the river velocity (down the
river) to equal the resulting velocity. And so any calculation of the Distance
C or the Average Speed C ("Resultant Velocity") can be performed
using the Pythagorean theorem.