Addition of Non-Perpendicular Vectors
Now we will consider situations in which the
two (or more) vectors that are being added are not at right angles to each
other. The Pythagorean theorem is not applicable to such situations since it applies
only to right triangles. Two non-perpendicular vectors will not form a right
triangle. Yet it is possible to force two (or more) non-perpendicular vectors
to be transformed into other vectors that do form a right triangle. The trick
involves the concept of a
vector component and the process of
vector resolution.
A vector component describes the effect of a vector in
a given direction. Any angled vector has two components; one is directed horizontally and
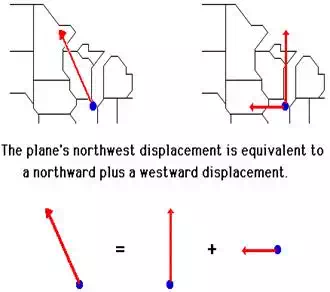
the other is directed vertically. For instance, a northwest vector has a
northward component and a westward component. Together, the effect these two
components are equal to the overall effect of the angled vector. As an
example, consider a plane that flies northwest from Chicago O'Hare airport
towards the Canada border. The northwest displacement vector of the plane has
two components - a northward component and a westward component. When added
together, these two components are equal to the overall northwest displacement.
This is shown in the diagram below.
The northwest vector has north and west components that
are represented as Ax and Ay. It can be said that
A = Ax + Ay
So whenever we think of a northwest vector, we
can think instead of two vectors - a north and a west vector. The two
components Ax + Ay can be
substituted in for the single vector A in the
problem.
Now suppose that your task involves
adding two non-perpendicular vectors together. We will call the vectors A and B.
Vector A is a nasty
angled vector that is neither horizontal nor vertical. And vector B is a nice,
polite vector directed horizontally. The situation is shown below.
Of course nasty vector A has two
components - Ax and Ay. These two components together are equal to vector A. That is, A = Ax + Ay .
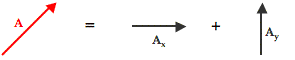
And since this is true, it makes since to say
that A + B
= Ax + Ay + B.
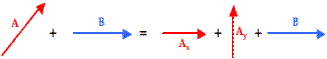
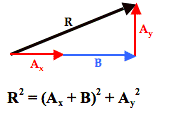
And so the problem of A + B has been
transformed into a problem in which all vectors are at right angles to each
other. Nasty has been replaced by nice and that
should make any physics student happy. With all vectors being at right angles
to one another, their addition leads to a resultant that is at the hypotenuse
of a right triangle. The Pythagorean theorem can then be used to determine the magnitude of the
resultant.
To see how this process works with an actual vector
addition problem, consider Example 3 shown below.
Example 3:
Max
plays middle linebacker for South's
football team. During one play in last Friday night's game against New Greer
Academy, he made the following movements after the ball was snapped on third
down. First, he back-pedaled in the
southern direction for 2.6 meters. He then shuffled to his left (west) for a
distance of 2.2 meters. Finally, he made a half-turn and ran downfield a
distance of 4.8 meters in a direction of 240° counter-clockwise from east (30°
W of S) before finally knocking the wind out of New Greer's wide receiver.
Determine the magnitude and direction of Max's overall displacement.
As is the usual case, the solution begins with a diagram
of the vectors being added.
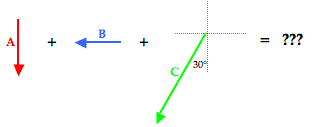
To assist in the discussion, the three vectors have
been labeled as
vectors A, B, and C. The resultant is the vector sum of these three vectors; a
head-to-tail vector addition diagram reveals that the resultant is directed
southwest. Of the three vectors being added, vector C is clearly the nasty vector.
Its direction is neither due south nor due west. The solution involves
resolving this vector into its components.
The process of vector resolution was discussed earlier in this lesson. The process involves using the magnitude and the sine and
cosine functions to determine the x- and y-components of the vector. Vector C
makes a 30° angle with the southern direction. By sketching a right triangle
with horizontal and vertical legs and C as the hypotenuse, it becomes possible
to determine the components of vector C. This is shown in the diagram below.
The side adjacent this 30° angle in the triangle is the vertical side; the
vertical side represents the vertical (southward) component of C - Cy. So to
determine Cy, the cosine function is used. The side opposite the 30°
angle is the horizontal side; the horizontal side represents the horizontal
(westward) component of C - Cx. The values of Cx and Cy can be determined by using SOH CAH TOA. The cosine function
is used to determine the southward component since the southward component is
adjacent to the 30° angle. The sine function is used to determine the westward
component since the westward component is the side opposite to the 30° angle.
The work is shown below.
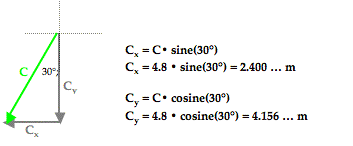
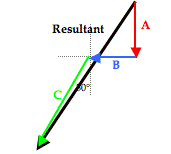
Now our vector addition problem has been transformed
from the addition of two nice vectors and one nasty vector into the addition of
four nice vectors.
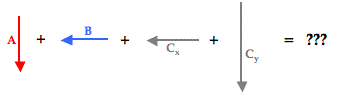
With all vectors oriented along are customary
north-south and east-west axes, they can be added head-to-tail in any order to
produce a right triangle whose the hypotenuse is the resultant. Such a diagram
is shown below.
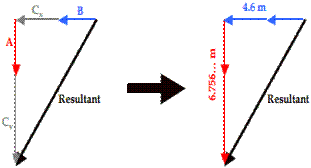
The triangle's perpendicular sides have
lengths of 4.6 meters and 6.756 meters. The length of the horizontal side (4.6
m) was determined by adding the values of B (2.2 m) and Cx (2.4 m). The length of the vertical side (6.756
m) was
determined by adding the values of A (2.6 m) and Cy (4.156
m).
The resultant's magnitude (R) can now be determined using the Pythagorean theorem.
R2 = (6.756
m)2 + (4.6 m)2
R2 = 45.655
m2 + 21.16 m2
R2 = 66.815
m2
R = SQRT(66.815
m2 )
R = 8.174
m
R = ~8.2 m
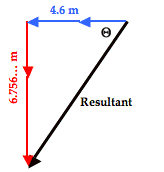The direction of the resultant can be determined by finding
the angle that the resultant makes with either the north-south or the east-west
vector. The diagram at the right shows the angle theta (Θ) marked inside
the vector addition triangle. This angle theta is the angle that the resultant
makes with west. Its value can be determined using the tangent function. The
tangent function (as in TOA) relates the angle value to the ratio of the
lengths of the opposite side to the adjacent side. That is,
Tangent (Θ) = (6.756
m)/(4.6
m) = 1.46889
Using the inverse tangent function, the angle theta
(Θ) can be determined. On most calculators, this involves using the
2nd-Tangent buttons.
Θ = tan-1 (1.46889
)
= 55.7536
°
Θ = ~56°
This 56° angle is the angle between the
resultant vector (drawn in black above) and the westward direction. This makes
the direction 56° south of west. The direction of the resultant based on the
counter-clockwise from east convention (CCW) can be determined by adding 180°
to the 56°. So the CCW direction is 236°.
Example 4 provides one final example of how to combine
vector resolution with vector addition in order to add three or more
non-perpendicular vectors. Because this example includes three particularly nasty
vectors, a table will be used to organize the information about hemagnitude and direction of the
components. The use of a table is a wise idea when problems get complicated.
Example 4:
Cameron
Per (his friends call him Cam) and Baxter Nature are on a hike. Starting from
home base, they make the following movements.
A: 2.65 km, 140° CCW
B: 4.77 km, 252° CCW
C: 3.18 km, 332° CCW
Determine
the magnitude and direction of their overall displacement.
The visual representation of the situation is shown
below.
To determine the resultant, the three individual
vectors are resolved into horizontal and vertical components. The angle
information about each vector is used to form a right triangle in which the
vector is the hypotenuse and the perpendicular sides are oriented along the
east-west and north-south axes. This is shown in the diagram below.
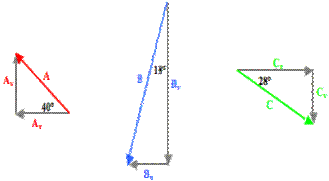
Trigonometric functions - sine, cosine and tangent -
are then used to determine the magnitude of the horizontal and vertical
component of each vector. The work is shown and organized in the table below.
|
Vector |
East-West
Component |
North-South Component |
|
|
(2.65 km)cos(40°) |
(2.65
km)sin(40°) |
|
|
(4.77 km)sin(18°) |
(4.77
km)cos(18°) |
|
|
(3.18 km)cos(28°) |
(3.18
km)sin(28°) |
|
|
0.696 km, West |
4.326
km, South |
The last row of the above table represents the sum of
all the East-West components and the sum of all the North-South components. The
resultant consists of these two components. The resultant is determined by
adding together these two the components to form a right triangle that has a
hypotenuse that is equal to the resultant. This typically involves adding all
the horizontal components to determine the total length of the horizontal side
of the right triangle
and adding all the vertical components to determine the
total length of the vertical side of the right triangle. This is done in the
table above by simple adding another row to the table for the vector sum of all
the components. In adding the east-west components of all the individual
vectors, one must consider that an eastward component and a westward component
would add together as a positive and a negative. Some students prefer to think of this as
subtraction as opposed to addition. In actuality, it really is addition - the
addition of vectors with opposite direction. Similarly, a northward and a
southward component would also add together as a positive and a negative.
Once the bottom row is accurately determined, the magnitude of the resultant
can be determined using Pythagorean theorem.
R2 = (0.696 km)2 + (4.326
km)2
R2 = 0.484 km2 + 18.714 km2
R2 = 19.199 km2
R = SQRT(19.199 km2)
R = ~4.38 km
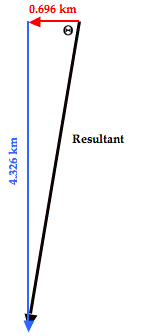The direction of the resulting displacement can be determined
by constructing the final triangle from the components of the resultant. The
components of the resultant are simply the sum the east-west and north-south
components. Once done, SOH CAH TOA is used to determine the angle that the
resultant makes with a nearby axis. The diagram is shown at the right. The
angle labeled as
theta (Θ) is the angle between the resultant vector and the west axis.
This angle can be calculated as follows:
Tangent(Θ) = opposite/adjacent
Tangent(Θ) = (4.326 km)/(0.696 km)
Tangent(Θ) = 6.216
Θ = tan-1(6.216)
Θ = 80.9°
This angle measure represents the angle of rotation of
the vector south of due west. It would be worded as 80.9° south of west. Since
west is 180° counterclockwise from east, the direction could also be expressed in
the counterclockwise (CCW) from east convention as 260.9°.
So the result of our analysis is that the overall
displacement is 4.38 km with a direction of 260.9° (CCW).