Force is a Vector
Quantity
A force is a vector quantity. As learned
in an earlier unit, a vector quantity is a quantity that has both magnitude and
direction. To fully describe the force acting upon an object, you must describe
both the magnitude (size or numerical value) and the direction. Thus, 10 Newton
is not a full description of the force acting upon an object. In contrast, 10
Newton, downward is a complete description of the force acting upon an object;
both the magnitude (10 Newton) and the direction (downward) are given.
 Because a force is a vector that has a direction, it is common to
represent forces using diagrams in which a force is represented by an arrow.
Such vector diagrams were introduced in an earlier unit and are
used throughout the study of physics. The size of the arrow is reflective of
the magnitude of the force and the direction of the arrow reveals the direction
that the force is acting. (Such diagrams are known as free-body diagrams and
are discussed later in this lesson.) Furthermore, because forces are vectors, the
effect of an individual force upon an object is often canceled by
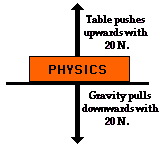
the effect of another force. For example, the effect of a 20-Newton upward
force acting upon a book is canceled by the
effect of a 20-Newton downward force acting upon the book. In such instances,
it is said that the two individual forces balance
each other; there would be no unbalanced
force acting upon the book.
Because a force is a vector that has a direction, it is common to
represent forces using diagrams in which a force is represented by an arrow.
Such vector diagrams were introduced in an earlier unit and are
used throughout the study of physics. The size of the arrow is reflective of
the magnitude of the force and the direction of the arrow reveals the direction
that the force is acting. (Such diagrams are known as free-body diagrams and
are discussed later in this lesson.) Furthermore, because forces are vectors, the
effect of an individual force upon an object is often canceled by
the effect of another force. For example, the effect of a 20-Newton upward
force acting upon a book is canceled by the
effect of a 20-Newton downward force acting upon the book. In such instances,
it is said that the two individual forces balance
each other; there would be no unbalanced
force acting upon the book.
 Other situations could be imagined in which two of the individual vector
forces cancel each other ("balance"), yet a third individual force
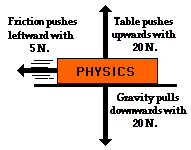
exists that is not balanced by another force. For example, imagine a book
sliding across the rough surface of a table from left to right. The downward
force of gravity and the upward force of the table supporting the book act in
opposite directions and thus balance each other. However, the force of friction
acts leftwards, and there is no rightward force to balance it. In this case, an unbalanced
force acts upon the book to change its state of
motion.
Other situations could be imagined in which two of the individual vector
forces cancel each other ("balance"), yet a third individual force
exists that is not balanced by another force. For example, imagine a book
sliding across the rough surface of a table from left to right. The downward
force of gravity and the upward force of the table supporting the book act in
opposite directions and thus balance each other. However, the force of friction
acts leftwards, and there is no rightward force to balance it. In this case, an unbalanced
force acts upon the book to change its state of
motion.
The exact details of drawing free-body diagrams are discussed
later. For now, the emphasis is upon the fact that a force is a vector quantity
that has a direction. The importance of this fact will become clear as we analyze the individual forces acting upon an object later in
this lesson.