How Fast? And How Far?
Free-falling objects are in a state of acceleration.
Specifically, they are accelerating at a rate of 9.8 m/s/s. This is to say that
the velocity of a free-falling object is changing by 9.8 m/s every second. If
dropped from a position of rest, the object will be traveling 9.8 m/s
(approximately 10 m/s) at the end of the first second, 19.6 m/s (approximately
20 m/s) at the end of the second second, 29.4 m/s (approximately 30 m/s) at the end of the third
second, etc. Thus, the velocity of a free-falling object that has been dropped
from a position of rest is dependent upon the time that it has fallen. The
formula for determining the velocity of a falling object after a time of t seconds is
vf = g * t
(dropped from rest)
where g is the acceleration of gravity. The value for g
on Earth is 9.8 m/s/s. The above equation can be used to calculate the velocity
of the object after any given amount of time when dropped from rest. Example
calculations for the velocity of a free-falling object after six and eight
seconds are shown below.
Example Calculations:
At t = 6 s
vf = (9.8 m/s2) * (6 s) = 58.8 m/s
At t = 8 s
vf = (9.8 m/s2) * (8 s) = 78.4 m/s
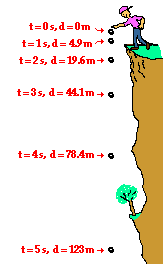
The distance that a free-falling object has fallen from a
position of rest is also dependent upon the time of fall. This distance can be
computed by use of a formula; the distance fallen after a time of t seconds is given by the formula.
d = 0.5 * g * t2
(dropped from rest)
where g is the acceleration of gravity (9.8 m/s/s on
Earth). Example calculations for the distance fallen by a free-falling object
after one and two seconds are shown below.
Example Calculations:
At t = 1 s
d = (0.5) * (9.8 m/s2) * (1 s)2 = 4.9 m
At t = 2 s
d = (0.5) * (9.8 m/s2) * (2 s)2 = 19.6 m
At t = 5 s
d = (0.5) * (9.8 m/s2) * (5 s)2 = 123 m
(rounded from 122.5 m)
The diagram below (not drawn to scale) shows the results of
several distance calculations for a free-falling object dropped from a position
of rest.