The Critical Angle

In the previous part of Lesson 3, the phenomenon of total internal reflection was introduced. Total internal reflection (TIR) is the phenomenon that involves the reflection of all the incident light off the boundary. TIR only takes place when both of the following two conditions are met:

· a light ray is in the more dense medium and approaching the less dense medium.

· the angle of incidence for the light ray is greater than the so-called critical angle.

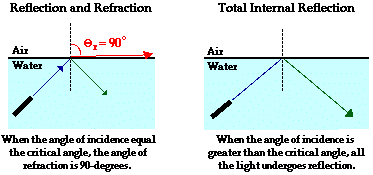
In our introduction to TIR, we used the example of light traveling through water towards the boundary with a less dense material such as air. When the angle of incidence in water reaches a certain critical value, the refracted ray lies along the boundary, having an angle of refraction of 90-degrees. This angle of incidence is known as the critical angle; it is the largest angle of incidence for which refraction can still occur. For any angle of incidence greater than the critical angle, light will undergo total internal reflection.

The Critical Angle Derivation

So the critical angle is defined as the angle of incidence that provides an angle of refraction of 90-degrees. Make particular note that the critical angle is an angle of incidence value. For the water-air boundary, the critical angle is 48.6-degrees. For the crown glass-water boundary, the critical angle is 61.0-degrees. The actual value of the critical angle is dependent upon the combination of materials present on each side of the boundary.

Let's consider two different media - creatively named medium i (incident medium) and medium r (refractive medium). The critical angle is the Θ_i that gives a Θ_r value of 90-degrees. If this information is substituted into Snell's Law equation, a generic equation for predicting the critical angle can be derived. The derivation is shown below.

n_i *• sine(Θ_i) = n_r • sine (Θ_r)

n_i • sine(Θ_crit) = n_r • sine(90 degrees)

n_i • sine(Θ_crit) = n_r

sine(Θ_crit) = n_r/n_i

Θ_crit= sine^-1 (n_r/n_i) = invsine (n_r/n_i)

The critical angle can be calculated by taking the inverse-sine of the ratio of the indices of refraction. The ratio of n_r/n_i is a value less than 1.0. In fact, for the equation to even give a correct answer, the ratio of n_r/n_imust be less than 1.0. Since TIR only occurs if the refractive medium is less dense than the incident medium, the value of n_i must be greater than the value of n_r. If at any time the values for the numerator and denominator become accidentally switched, the critical angle value cannot be calculated. Mathematically, this would involve finding the inverse-sine of a number greater than 1.00 - which is not possible. Physically, this would involve finding the critical angle for a situation in which the light is traveling from the less dense medium into the more dense medium - which again, is not possible.

This equation for the critical angle can be used to predict the critical angle for any boundary, provided that the indices of refraction of the two materials on each side of the boundary are known. Examples of its use are shown below:

Example A

Calculate the critical angle for the crown glass-air boundary. Refer to the table of indices of refraction if necessary.

The solution to the problem involves the use of the above equation for the critical angle.

Θcrit = sin^-1 (n_r/n_i) = invsine (n_r/n_i)

Θcrit = sin^-1 (1.000/1.52) = 41.1 degrees

Example B

Calculate the critical angle for the diamond-air boundary. Refer to the table of indices of refraction if necessary.

The solution to the problem involves the use of the above equation for the critical angle.

Θcrit = sin^-1 (n_r/n_i) = invsine (n_r/n_i)

Θcrit = sin^-1 (1.000/2.42) = 24.4 degrees

TIR and the Sparkle of Diamonds

Relatively speaking, the critical angle for the diamond-air boundary is an extremely small number. Of all the possible combinations of materials that could interface to form a boundary, the combination of diamond and air provides one of the largest differences in the index of refraction values. This means that there will be a very small n_r/n_i ratio and subsequently a small critical angle. This peculiarity about the diamond-air boundary plays an important role in the brilliance of a diamond gemstone. Having a small critical angle, light has the tendency to become "trapped" inside of a diamond once it enters. A light ray will typically undergo TIR several times before finally refracting out of the diamond. Because the diamond-air boundary has such a small critical angle (due to diamond's large index of refraction), most rays approach the diamond at angles of incidence greater than the critical angle. 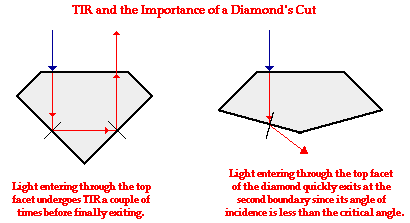This gives diamond a tendency to sparkle. The effect can be enhanced by the cutting of a diamond gemstone with a strategically planned shape. The diagram below depicts the total internal reflection within a diamond gemstone with a strategic and a non-strategic cut.

Check Your Understanding

1. Suppose that the angle of incidence of a laser beam in water and heading towards air is adjusted to 50-degrees. Use Snell's law to calculate the angle of refraction? Explain your result (or lack of result).

See Answer

Good luck! This problem has no solution. The angle of incidence is greater than the critical angle, so TIR occurs. There is no angle of refraction.

2. Aaron Agin is trying to determine the critical angle of the diamond-glass surface. He looks up the index of refraction values of diamond (2.42) and crown glass (1.52) and then tries to compute the critical angle by taking the

invsine(2.42/1.52).

Unfortunately, Aaron's calculator keeps telling him he has an ERROR! Aaron hits the calculator and throws it own the ground a few times; he then repeats the calculation with the same result. He then utters something strange about the pizza he had slopped on it the evening before and runs out of the library with a disappointed disposition. What is Aaron's problem? (That is, what is the problem with his method of calculating the critical angle?)

See Answer

Poor Aaron! It's not your pizza that's causing the problem; its your inappropriate use of the equation. You will need to take the inverse sine of the ratio (1.52 / 2.42). You have switched your numerator and denominator.

3. Calculate the critical angle for an ethanol-air boundary. Refer to the table of indices of refraction if necessary.

See Answer

Θcrit = sine^-1 (n_i / n_r)

Θcrit= sine^-1 (1.0 / 1.36)

Θcrit = 47.3 degrees

4. Calculate the critical angle for a flint glass-air boundary. Refer to the table of indices of refraction if necessary.

See Answer

Θcrit = sine^-1 (n_i / n_r)

Θcrit = sine^-1 (1.0 / 1.58)

Θcrit = 39.3 degrees

5. Calculate the critical angle for a diamond-crown glass boundary. Refer to the table of indices of refraction if necessary.

See Answer

Θcrit = sine^-1 (n_i / n_r)

Θcrit = sine^-1 (1.52 / 2.42)

Θcrit = 38.9 degrees

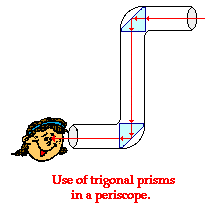6. Some optical instruments, such as periscopes and binoculars use trigonal prisms instead of mirrors to reflect light around corners. Light typically enters perpendicular to the face of the prism, undergoes TIR off the opposite face and then exits out the third face. Why do you suppose the manufacturer prefers the use of prisms instead of mirrors?

See Answer

A prism will allow light to undergo total internal reflection whereas a mirror allows light to both reflect and refract. So for a prism, 100 percent of the light is reflected. But for a mirror, only about 95 percent of the light is reflected. For these reasons, a prism will produce a brighter image due to the greater percent of light being reflected.