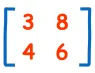
A Matrix
(This one has 2 Rows and 2 Columns)
Determinant of a Matrix
The determinant of a matrix is a special number that can be calculated from a square matrix.
A Matrix is an array of numbers:

A Matrix
(This one has 2 Rows and 2 Columns)
The determinant of that matrix is (calculations are explained later):
3×6 − 8×4 = 18 − 32 = −14
What is it for?
The determinant helps us find the inverse of a matrix, tells us things about the matrix that are useful in systems of linear equations, calculus and more.
Symbol
The symbol for determinant is two vertical lines either side.
Example:
|A| means the determinant of the matrix A
(Exactly the same symbol as absolute value.)
Calculating the Determinant
First of all the matrix must be square (i.e. have the same number of rows as columns). Then it is just basic arithmetic. Here is how:
For a 2×2 Matrix
For a 2×2 matrix (2 rows and 2 columns):
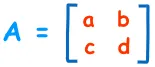
The determinant is:
|A| = ad − bc
"The determinant of A equals a times d minus b times c"
It is easy to remember when you think of a cross: Blue is positive (+ad), Red is negative (−bc) |
|
|
Example:
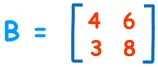
|B|= 4×8 − 6×3
= 32 − 18
= 14
For a 3×3 Matrix
For a 3×3 matrix (3 rows and 3 columns):
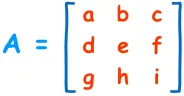
The determinant is:
|A| = a(ei − fh) − b(di − fg) + c(dh − eg)
"The determinant of A equals ... etc"
It may look complicated, but there is a pattern:
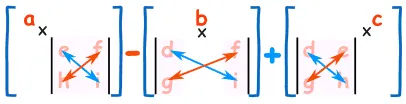
To work out the determinant of a 3×3 matrix:
Multiply a by the determinant of the 2×2 matrix that is not in a's row or column.
Likewise for b, and for c
Sum them up, but remember the minus in front of the b
As a formula (remember the vertical bars || mean "determinant of"):
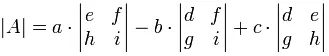
"The determinant of A equals a times the determinant of ... etc"
Example:
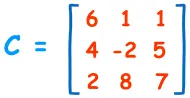
|C|= 6×(−2×7 − 5×8) − 1×(4×7 − 5×2) + 1×(4×8 − (−2×2))
= 6×(−54) − 1×(18) + 1×(36)
= −306
For 4×4 Matrices and Higher
The pattern continues for 4×4 matrices:
plus a times the determinant of the matrix that is not in a's row or column,
minus b times the determinant of the matrix that is not in b's row or column,
plus c times the determinant of the matrix that is not in c's row or column,
minus d times the determinant of the matrix that is not in d's row or column,
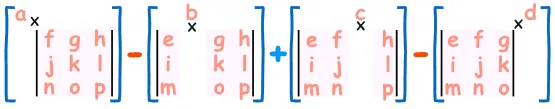
As a formula:
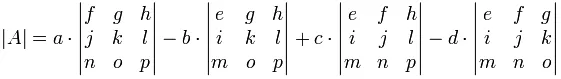
Notice the +−+− pattern (+a... −b... +c... −d...). This is important to remember.