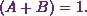Partial Fractions Of Higher Degree Numerators With Examples

EXAMPLE 1

Write the following expression into the partial fraction form:

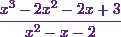$\cfrac{x^3-2x^2-2x+3}{x^2-x-2}$ .

SOLUTION

Here the given expression is: 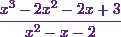$\cfrac{x^3-2x^2-2x+3}{x^2-x-2}$ .

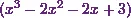That means the denominator is (x^2-x-2) . Also the numerator is (x^3-2x^2-2x+3) .

So, I can say that the degree of the numerator is 3. The denominator’s degree is 2.

This means the numerator’s degree is higher than that of the denominator. Therefore this expression has a higher degree numerator.

So, my first job is to reduce the degree of the numerator.

STEP 1

First of all, I’ll divide the numerator by the denominator.

So I can rewrite the expression as

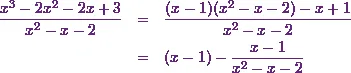
$\begin{eqnarray*} \frac{x^3-2x^2-2x+3}{x^2-x-2}&=&\frac{(x-1)(x^2-x-2)-x+1}{x^2-x-2}\\ &=&(x-1)-\frac{x-1}{x^2-x-2} \end{eqnarray*}$

Now 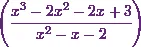$\left(\cfrac{x^3-2x^2-2x+3}{x^2-x-2}\right)$ is reduced to two parts:

one is (x-1) and
the other is 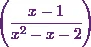$\left(\cfrac{x-1}{x^2-x-2}\right)$ .

The first part cannot be reduced any more.

But I can still reduce the second part. So let’s start then.

STEP 2

Here I start with the factorisation. Then I use the assumptions like

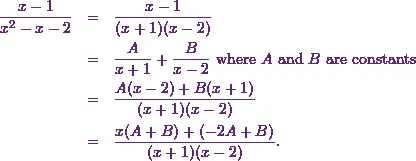
$\begin{eqnarray*} \frac{x-1}{x^2-x-2}&=&\frac{x-1}{(x+1)(x-2)}\\ &=&\frac{A}{x+1}+\frac{B}{x-2} ~\text{where $A$ and $B$ are constants}\\ &=&\frac{A(x-2)+B(x+1)}{(x+1)(x-2)}\\ &=&\frac{x(A+B)+(-2A+B)}{(x+1)(x-2)}. \end{eqnarray*}$

Now I have the same denominator on both left hand and right hand sides.

Therefore I can say,

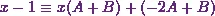$x-1 \equiv x(A+B)+(-2A+B)$ .

I can compare the coefficients of on both sides.

Thus I get

(1) $\begin{equation*} (A+B)=1. \end{equation*}$

I can also compare constants on both sides. From that I have

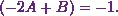
(2) $\begin{equation*} (-2A+B)=-1. \end{equation*}$

From equation (1), I have 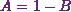A=1-B .

Next substitute this value of 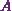in equation (2).

Then I get

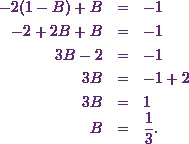
$\begin{eqnarray*} -2(1-B)+B&=&-1\\ -2+2B+B&=&-1\\ 3B-2&=&-1\\ 3B&=&-1+2\\ 3B&=&1\\ B&=&\frac{1}{3}. \end{eqnarray*}$

Now I substitute this value of in equation (1).

Therefore I have

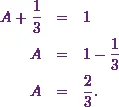
$\begin{eqnarray*} A+\frac{1}{3}&=&1\\ A&=&1-\frac{1}{3}\\ A&=&\frac{2}{3}. \end{eqnarray*}$

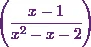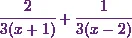So the partial fraction of $\left(\cfrac{x-1}{x^2-x-2}\right)$ is $\cfrac{2}{3(x+1)}+\cfrac{1}{3(x-2)}$ .

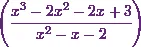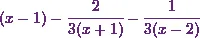Hence I can conclude that the partial fraction of $\left(\cfrac{x^3-2x^2-2x+3}{x^2-x-2}\right)$ is $(x-1)-\cfrac{2}{3(x+1)} - \cfrac{1}{3(x-2)}$ .

This is the solution to the given example.