Economics of power factor improvement:

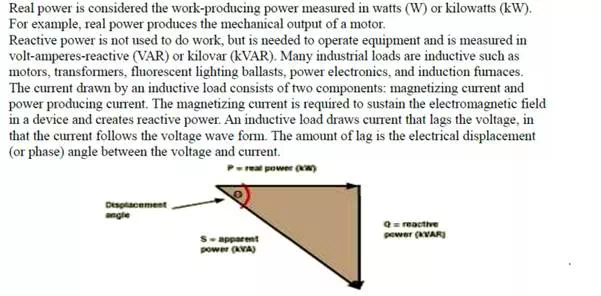
For example, real power produces the mechanical output of a motor. Reactive power is not used to do work, but is needed to operate equipment and is measured in volt-amperes-reactive (VAR) or kilovar (kVAR). Many industrial loads are inductive such as motors, transformers, fluorescent lighting ballasts, power electronics, and induction furnaces. The current drawn by an inductive load consists of two components: magnetizing current and power producing current.

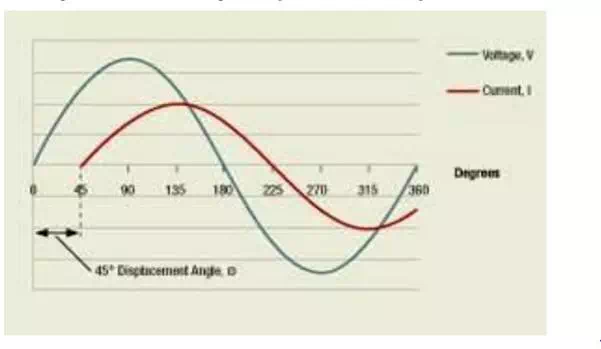
 The magnetizing current is required to sustain the electromagnetic field in a device and creates reactive power. An inductive load draws current that lags the voltage, in that the current follows the voltage wave form.

The amount of lag is the electrical displacement (or phase) angle between the voltage and current. n the absence of harmonics, apparent power (also known as demand power) is comprised of (vectorial sum) both real and reactive power and is measured in units of volt-amps (VA) or kilovolt-amps (kVA). Power factor (PF) is the ratio of the real power to apparent power and represents how much real power electrical equipment uses. It is a measure of how effectively electrical power is being used.

 Power factor is also equal to the cosine of the phase angle between the voltage and current

Electrical loads demand more power than they consume. Induction motors convert at most 80% to 90% of the delivered power into useful work or electrical losses. The remaining power is used to establish an electromagnetic field in the motor. The field is alternately expanding and collapsing (once each cycle), so the power drawn into the field in one instant is returned to the electric supply system in the next instant. Therefore, the average power drawn by the field is zero, and reactive power does not register on a kilowatt-hour meter. The magnetizing current creates reactive power. Although it does no useful work, it circulates between the generator and the load and places a heavier drain on the power source as well as the transmission and distribution system.

As a means of compensation for the burden of supplying extra current, many utilities establish a power factor penalty in their rate schedule. A minimum power factor, usually 0.85 to 0.95, is established. When a customer’s power factor drops below the minimum value, the utility collects a low power factor revenue premium on the customer’s bill. Another way some utilities collect a low power factor premium is to charge for kVA (apparent power) rather than kW (real power). With a diverse range of billing rate structures imposed by electrical utilities especially for large users, it is imperative to fully understand the billing method employed.

Improving power factor

Adding capacitors is generally the most economical way to improve a facility’s power factor. While the current through an inductive load lags the voltage, current to a capacitor leads the voltage. Thus, capacitors serve as a leading reactive current generator to counter the lagging reactive current in a system.

The expression “release of capacity” means that as power factor of the system is improved, the total current flow will be reduced. This permits additional loads to be added and served by the existing system. In the event that equipment, such as transformers, cables, and generators, may be thermally overloaded, improving power factor may be the most economical way to reduce current and eliminate the overload condition.

Primarily, the cost-effectiveness of power factor correction depends on a utility’s power factor penalties. It is crucial to understand the utility’s rate structure to determine the return on investment to improve power factor.

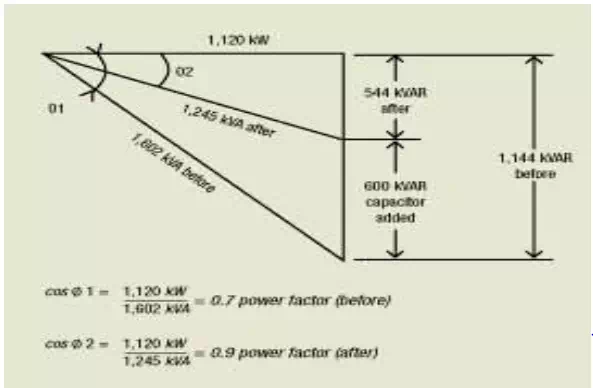
Maintaining a high power factor in a facility will yield direct savings. In addition to reducing power factor penalties imposed by some utilities, there may be other economic factors that, when considered in whole, may lead to the addition of power factor correction capacitors that provide a justifiable return on investment. Other savings, such as decreased distribution losses, improved voltage reduction, and increased facility current carrying capacity, are less obvious. Though real, often these reductions yield little in cost savings and are relatively small in comparison to the savings to be gained from reducing power factor penalties.

Harmonic current considerations:

This article intentionally assumes that a facility does not have significant harmonic currents present. However, some caution must be taken when applying capacitors in a circuit where harmonics are present (true power factor).

Although capacitors themselves do not generate harmonics, problems arise when capacitors for power factor correction improvement are applied to circuits with nonlinear loads that interject harmonic currents. Those capacitors may lower the resonant frequency of that circuit enough to create a resonant condition. Resonance is a special condition in which the inductive reactance is equal to the capacitive reactance. As resonance is approached, the magnitude of harmonic current in the system and capacitor becomes much larger than the harmonic current generated by the nonlinear load. The current may be high enough to blow capacitor fuses, create other “nuisance” problems, or develop into a catastrophic event. A solution to this problem is to detune the circuit by changing the point where the capacitors are connected to the circuit, changing the amount of applied capacitance, or installing passive filter reactors to a capacitor bank, which obviously increases its cost. Use of an active harmonic filter may be another solution.