Examples of Electric Circuit Based on Node Voltage Method

Example;1

Find the current through 'ab-branch' ( I ) and voltage (V ) across the current source using Node-voltage method.

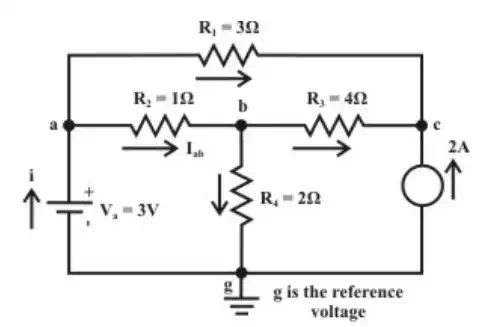
Solution:

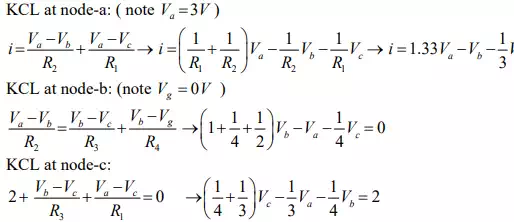
Using the value of  Va = 3V in equations we get the following equations:

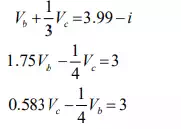
Example;2

Determine the current, i shown in fig. using node-voltage method --- (a) applying voltage to current source conversion (b) without any source conversion.

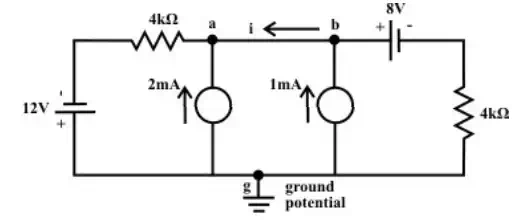
Solution:

Part(a):

In node voltage analysis, sometimes the solution turns out to be very simple while we change all series branches containing voltage sources to their equivalent current sources. On the other hand, we observed in the loop analysis method that the conversion of current source to an equivalent voltage makes the circuit analysis very easy  and simple. For this example, both the practical voltage sources (one is left of ‘node-a’ and other is right of ‘node-b’) are converted into practical current sources. After transformation, the circuit is redrawn and shown in fig.(a)

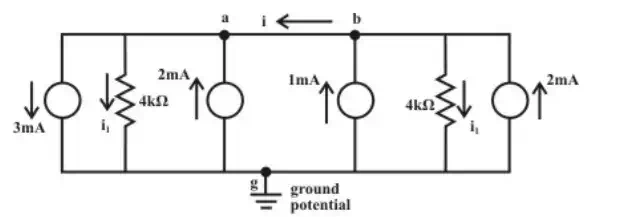
                                                                  (a)

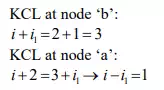
From Above equations , one can get (current flows from ‘b’ to ‘a’) and

(B)

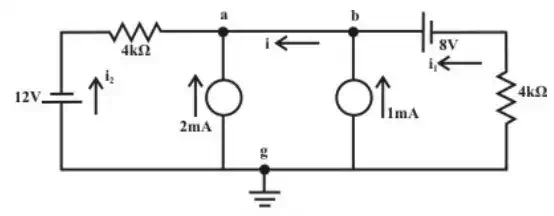
Part(b):

Let us assume is the current flowing through the battery source from ‘right to left’ and is the current flowing through the 12-battery source from ‘bottom to top’(see Fig(b)).

KCL at node ‘b’: It is assumed that the current flowing in 4 kΩ resistor from bottom to top terminal. This implies that the bottom terminal of 4 kΩ resistor is higher potential than the top terminal.

KCL at node ‘a’: (currents are in m A)

From Above equations , we get Va = 4V and i  = 2 m A(current flows from ‘b’ to ‘a’).