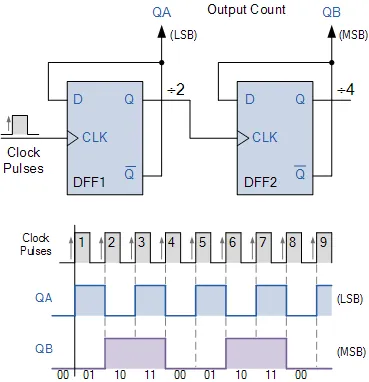
MOD-4 Counter

Technically as well as being a 1-bit storage device, a single flip-flop on its own could be thought of as a MOD-2 counter, as it has a single output resulting in a count of two, either a 0 or 1, on the application of the clock signal. But a single flip-flop on its own produces a limited counting sequence, so by connecting together more flip-flops to form a chain, we can increase the counting capacity and construct a MOD counter of any value.

If a single flip-flop can be considered as a modulo-2 or MOD-2 counter, then adding a second flip-flop would give us a MOD-4 counter allowing it to count in four discrete steps. The overall effect would be to divide the original clock input signal by four. Then the binary sequence for this 2-bit MOD-4 counter would be: 00, 01, 10, and 11 as shown.

MOD-4 Counter and Timing Diagram

Note that for simplicity, the switching transitions of QA, QB and CLK in the above timing diagram are shown to be simultaneous even though this connection represents an asynchronous counter. In reality there would be a very small switching delay between the application of the positive going clock (CLK) signal, and the outputs at QA and QB.

We can show visually the operation of this 2-bit asynchronous counter using a truth table and state diagram.

MOD-4 Counter State Diagram

We can see from the truth table of the counter, and by reading the values of QA and QB, when QA = 0 and QB = 0, the count is 00. After the application of the clock pulse, the values become QA = 1, QB = 0, giving a count of 01 and after the next clock pulse, the values become QA = 0, QB = 1, giving a count of 10. Finally the values become QA = 1, QB = 1, giving a count of 11. The application of the next clock pulse causes the count to return back to 00, and thereafter it counts continuously up in a binary sequence of: 00, 01, 10, 11, 00, 01 …etc.

Then we have seen that a MOD-2 counter consists of a single flip-flop and a MOD-4 counter requires two flip-flops,allowing it to count in four discrete steps. We could easily add another flip-flop onto the end of a MOD-4 counter to produce a MOD-8 counter giving us a 2³ binary sequence of counting from 000 up to 111, before resetting back to 000. A fourth flip-flop would make a MOD-16 counter and so on, in fact we could go on adding extra flip-flops for as long as we wanted.

MOD-8 Counter and State Diagram

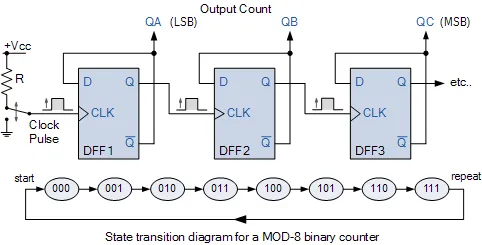
We can therefore construct mod counters to have a natural count of 2ⁿ states giving counters with mod counts of 2, 4, 8, 16, and so on, before repeating itself. But sometimes it is necessary to have a modulus counter that resets its count back to zero during the normal counting process and does not have a modulo that is a power of 2. For example, a counter having a modulus of 3, 5, 6, or 10.

Counters of Modulo “m”

Counters, either synchronous or asynchronous progress one count at a time in a set binary progression and as a result an “n”-bit counter functions naturally as a modulo 2ⁿ counter. But we can construct mod counters to count to any value we want by using one or more external logic gates causing it to skip a few output states and terminate at any count resetting the counter back to zero, that is all flip-flops have Q = 0.

In the case of modulo “m” counters, they do not count to all their possible states, but instead count to the “m” value and then return to zero. Obviously, “m” is a number smaller than 2ⁿ, (m < 2ⁿ). So how do we get a binary counter to return to zero part way through its count.

Fortunately, as well as counting, up or down, counters can also have additional inputs called CLEAR and PRESET which makes it possible to clear the count to zero, (all Q = 0) or to preset the counter to some initial value. The TTL 74LS74 has active-low Preset and Clear inputs.

Let’s assume for simplicity that the CLEAR inputs are all connected together and are active-high inputs allowing the flip-flops to operate normally when the Clear input is equal to 0 (LOW). But if the Clear input is at logic level “1” (HIGH), then the next positive edge of the clock signal will reset all the flip-flops into the state Q = 0, regardless of the value of the next clock signal.

Note also that as all the Clear inputs are connected together, a single pulse can also be used to clear the outputs (Q) of all the flip-flops to zero before counting starts to ensure that the count actually starts from zero. Also some larger bit counters have an additional ENABLE or INHIBIT input pin which allows the counter to stop the count at any point in the counting cycle and hold its present state, before being allowed to continue counting again. This means the counter can be stopped and started at will without resetting the outputs to zero.

A Modulo-5 Counter

Suppose we want to design a MOD-5 counter, how could we do that. First we know that “m = 5”, so 2ⁿ must be greater than 5. As 2¹ = 2, 2² = 4, 2³ = 8, and 8 is greater than 5, then we need a counter with three flip-flops (N = 3) giving us a natural count of 000 to 111 in binary (0 to 7 decimal).

Taking the MOD-8 counter above, the truth table for the natural count is given as:

MOD-8 Counter and Truth Table

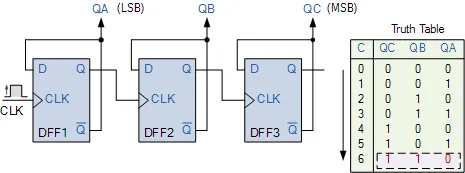
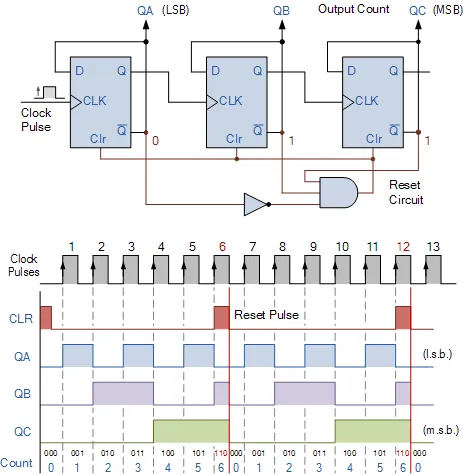
As we are constructing a MOD-5 counter, we want the counter to reset back to zero after a count of 5. However, we can see from the attached truth table that the count of six gives us the output condition of: QA = 0, QB = 1, and QC = 1.

We can decode this output state of 110 (6) to give us a signal to clear (Clr) the counter back to zero with the help of a 3-input AND gate (TTL 74LS11) and an inverter or NOT gate, (TTL 74LS04).

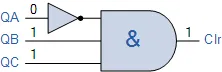
The inputs of the combinational logic circuit of the inverter and AND gate are connected to QA, QB, and QC respectively with the output of the AND gate at logic level “0” (LOW) for any combinations of the input other than the one we want.

In binary code, the output sequence count will look like this: 000, 001, 010, 011, 100, 101. But when it reaches the state of 110 (6), the combinational logic circuit will detect this 110 state and produce an output at logic level “1” (HIGH).

We can then use the resulting HIGH output from the AND gate to reset the counter back to zero after its output of 5 (decimal) count giving us the required MOD-5 counter. When the output from the combinational circuit is LOW it has no effect on the counting sequence.

MOD-5 Counter and Truth Table

Then we can use combinational logic decoding circuits around a basic counter, either synchronous or asynchronous to produce any type of MOD Counter we require as each of the counters unique output states can be decoded to reset the counter at the desired count.

In our simple example above, we have used a 3-input AND gate to decode the 110 state, but the first time that QB and QC are both at logic 1 is is when the count reaches six, so a 2-input AND gate connected to QB and QC could be used without the complication of the third input and the inverter.

However, one of the disadvantages of using asynchronous counters for producing a MOD counter of a desired count is that undesired effects called “glitches” can occur when the counter reaches its reset condition. During this brief time the outputs of the counter may take on an incorrect value, so it is sometimes better to use synchronous counters as modulo-m counters as all the flip-flops are clocked by the same clock signal so change state at the same time.

Modulus 10 Counter

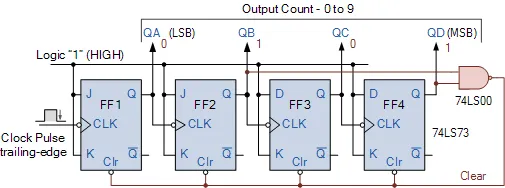
A good example of a modulo-m counter circuit which uses external combinational circuits to produce a counter with a modulus of 10 is the Decade Counter. Decade (divide-by-10) counters such as the TTL 74LS90, have 10 states in its counting sequence making it suitable for human interfacing where a digital display is required.

The decade counter has four outputs producing a 4-bit binary number and by using external AND and OR gates we can detect the occurrence of the 9th counting state to reset the counter back to zero. As with other mod counters, it receives an input clock pulse, one by one, and counts up from 0 to 9 repeatedly.

Once it reaches the count 9 (1001 in binary), the counter goes back to 0000 instead of continuing on to 1010. The basic circuit of a decade counter can be made from JK flip-flops (TTL 74LS73) that switch state on the negative trailing-edge of the clock signal as shown.

MOD-10 Decade Counter

MOD Counter Summary

We have seen in this tutorial about MOD Counters that binary counters are sequential circuits that generate binary sequences of bits as a result of a clock signal and the state of a binary counter is determined by the specific combination formed by all the counters outputs together.

The number of different output states a counter can produce is called the modulo or modulus of the counter. The Modulus (or MOD-number) of a counter is the total number of unique states it passes through in one complete counting cycle with a mod-n counter being described also as a divide-by-n counter.

The modulus of a counter is given as: 2ⁿ where n = number of flip-flops. So a 3 flip-flop counter will have a maximum count of 2³ = 8 counting states and would be called a MOD-8 counter. The maximum binary number that can be counted by the counter is 2ⁿ–1 giving a maximum count of (111)₂ = 2³–1 = 7₁₀. Then the counter counts from 0 to 7.

Common MOD counters include those with MOD numbers of 2, 4, 8 and 16 and with the use of external combinational circuits can be configured to count to any predetermined value other than one with a maximum 2ⁿ modulus. In general, any arrangement of a “m” number of flip-flops can be used to construct any MOD counter.

A common modulus for counters with truncated sequences is ten (1010), called MOD-10. A counter with ten states in its sequence is known as a decade counter. Decade counters are useful for interfacing to digital displays. Other MOD counters include the MOD-6 or MOD-12 counter which have applications in digital clocks to display the time of day.