POSITIONAL NUMBER SYSTEM

In a positional number system, there are only a few symbols called digits. These symbols represent different values, depending on the position they occupy in a number. The value of each digit in such a number is determined by three considerations.

1. The digit itself,

2. The position of the digit in the number, and

3. The base of the number system (where base is defined as the total number of digits available in the number system). In our day-to-day life, we use decimal number system. In this system, base is equal to 10 because there are altogether ten symbols or digit (0,1,2,3,4,5,6,7,8, and 9).

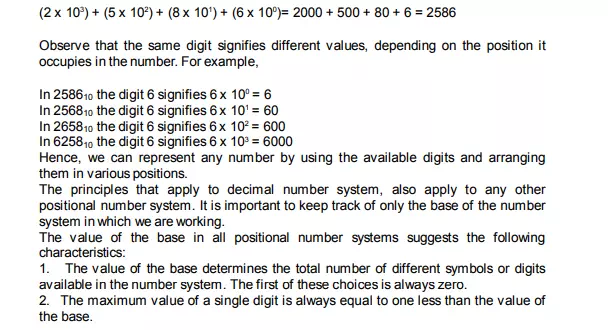
You know that in decimal number system. Successive positions to the left of the decimal point represent units, tens, hundreds, thousands, etc. However, notice that each position represents a specific power of the base (10). For example, decimal number 2586 (written as 2586) consists of digit 6 in units position, 8 in tens position, 5 in hundreds position, and 2 in thousands position, and its value can be written as: