Deoiling Hydrocyclones And Their Performance Prediction

The static hydrocyclone has rapidly been accepted as a compact and efficient means of removing dispersed hydrocarbons from water. The basic design of the static hydrocyclone is illustrated schematically in the figure below:

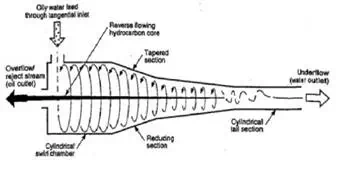
The water containing the dispersed hydrocarbons enters the hydrocyclone through a tangential inlet atthe top of the swirl chamber. As the liquids swirl along the hydrocyclone, the centrifugal forces genarated promote the separation of the hydrocarbon and water phases, with the hydrocarbon phase forming a thin core at the centre of the hydrocyclone.

By maintaining a suitable pressure ratio between the clean water outlet stream and the reject oil outlet stream, the geometry of the hydrocyclone will result in the thin hydrocarbon core flowing in a reverse direction, exiting from the top of the swirl chamber. The clean water exits from the tail section of the hydrocyclone.

The following definitions are commonly used for hydrocyclones:

Feed: The oil water stream entering the hydrocyclone

Underflow: The clean water stream exiting from the tail of the hydrocyclone

Reject Stream or Overflow: The concentrated hydrocarbon stream exiting from the head of the hydrocyclone through the reject port

Reject Ratio ®: The ratio of the reject and feed stream volumetric flow rates (R = Q_reject / Q_feed)

Performance Prediction:

Cut Size Diameter:

The cut size diameter is a droplet diameter which can be used to characterize the separation performance of a hydrocyclone.

A common cut size diameter definition is the 50% cut size d₅₀.This is defined as the hydrocarbon droplet diameter which has a 50% probability of leaving the hydrocyclone in the reject stream and a 50% probability of leaving the hydrocyclone in the water outlet stream i.e.if

n_{d, reject} / n_d,out = 1 then d = d₅₀

Another commonly used cut size is d75, representing the hydrocarbon droplet size with a 75% chance of being removed from the water stream.

Cut Size Diameter Correlations:

Several correlations are available for the prediction of the droplet cut size. The following correlations are presented:

Bradley Equation:

d₅₀ = 0.053*D_cycl*(ρ_c / (Re_i*Δρ))^0.5

where:

d₅₀ = droplet size diameter as defined above, m

D_cycl = Diameter of the cylindrical hydrocyclone chamber, m

ρ_c = Density of the continuous phase (water), kg/m³

Δρ = Density diff. between the continuous phase (water) (ρ_c) and the dispersed phase (oil) (ρ_oil), kg/m³

Note: The min. recommended density difference for a hydrocyclone is 50 kg/m³ @operating temperature

Re_i = D_i*V_i*ρ_c / μ_c

where:

Re_i = Reynolds number at the hydrocyclone inlet, dimensionless

D_i = inlet diameter at hydrocyclone inlet, m

V_i = Fluid velocity at inlet, m/s

μ_c = viscosity of the continuous phase (water), kg/m-s

Rietema Equation:

d₅₀ = 0.51*D_cycl*[ρ_c / ((Re_i)^1.375*Δρ)]^0.5

where:

d₅₀ = droplet size diameter as defined above, m

D_cycl = Diameter of the cylindrical hydrocyclone chamber, m

ρ_c = Density of the continuous phase (water), kg/m³

Δρ = Density diff. between the continuous phase (water) (ρ_c) and the dispersed phase (oil) (ρ_oil), kg/m³

Note: The min. recommended density difference for a hydrocyclone is 50 kg/m³ @operating temperature

Re_i = D_i*V_i*ρ_c / μ_c

where:

Re_i = Reynolds number at the hydrocyclone inlet, dimensionless

D_i = inlet diameter at hydrocyclone inlet, m

V_i = Fluid velocity at inlet, m/s

μ_c = viscosity of the continuous phase (water), kg/m-s

Coleman-Thew Empirical Model:

d₇₅ = (Hy₇₅*D_cycl ^3*μ_c / (Q*Δρ))^0.5

where:

d₇₅ = droplet size diameter as defined above, m

D_cycl = Diameter of the cylindrical hydrocyclone chamber, m

ρ_c = Density of the continuous phase (water), kg/m³

μ_c = viscosity of the continuous phase (water), kg/m-s

Q = Volumetric flow rate at inlet of hydrocyclone, m³/h

Δρ = Density diff. between the continuous phase (water) (ρ_c) and the dispersed phase (oil) (ρ_oil), kg/m³

Note: The min. recommended density difference for a hydrocyclone is 50 kg/m³ @operating temperature

Hy₇₅ = c₁*(Re_D)^c₂

where:

Re_D = 4*Q*ρ_c / (π*D_cycl*μ_c)

Hy₇₅ = hydrocyclone number, dimensionless

Re_D = Hydrocyclone reynolds number, dimensionless

ρ_c = Density of the continuous phase (water), kg/m³

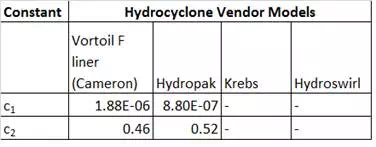
c₁ & c₂ = Empirically derived constants based on the hydrocyclone model

Some constants are tabulated below for some vendor models