External Transport Effects

For a solid-catalyzed reaction to take place, a reactant in the fluid phase must first diffuse through the stagnant boundary layer surrounding the catalyst particle. This mode of transport is described (in one spatial dimension) by the Stefan-Maxwell equations (see Appendix C for details):

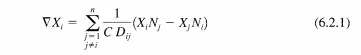
where Xi is the mole fraction of component i, C is the total concentration, Ni is the flux of component i, and Dij is the diffusivity of component i in j. The following relationship for diffusion of A in a two component mixture at constant pressure (constant total concentration) can be obtained from simplifying the Stefan-Maxwell equations:

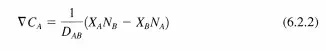
Since there are only two components in the mixture, Xs = 1 - XA and the above expression reduces to:

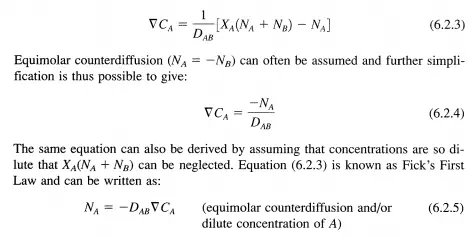
The diffusivities of gases and liquids typically have magnitudes that are 10- 1 and 10- 5 cm2 s-1, respectively. The diffusivity of gases is proportional to TI.5 and inversely proportional to P, whereas, the diffusivity of liquids is proportional to T and inversely proportional to viscosity Ii (may strongly depend on T).

To obtain the flux of reactant A through the stagnant boundary layer surrounding a catalyst particle, one solves Equation (6.2.~ with the appropriate boundary conditions. If the thickness of the boundary layer 8 is small compared to the radius of curvature of the catalyst particle, then the problem can be solved in one dimension as depicted in Figure 6.2.1. In this case, Fick's Law reduces to:

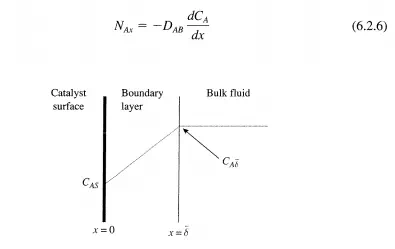
Since the flux of A must be constant through the stagnant film (conservation of mass), the derivative of the flux with respect to distance in the film must vanish:

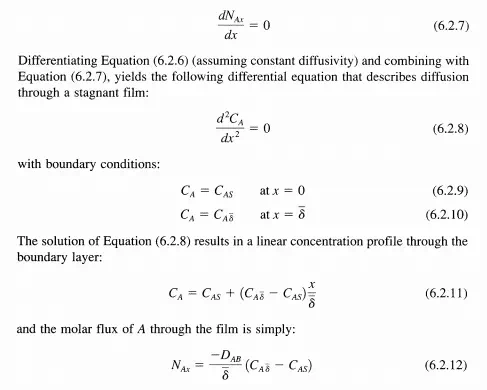
Although diffusion of reacting species can be wr:!tten in terms of the diffusivity and boundary layer thickness, the magnitude of 8 is unknown. Therefore, the mass-transfer coefficient is normally used. That is, the average molar flux from the bulk fluid to the solid surface is

where kc is the mass transfer coefficient over the surface area of the particle.