Tensile Stress and Strain 

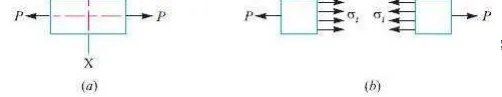
When a body is subjected to two equal and opposite axial pulls P (also called tensile load) as shown in Fig. (a), then the stress induced at any section of the body is known as tensile stress as shown in Fig. (b). A little consideration will show that due to the tensile load, there will be a decrease in cross-sectional area and an increase in length of the body. The ratio of the increase in length to the original length is known as tensile strain.

Let    P = Axial tensile force acting on the body,

A = Cross-sectional area of the body,

l = Original length, and

δl = Increase in length.

 Tensile stress, σt = P/A Tensile strain, εt = δl / l

Compressive Stress and Strain

 When a body is subjected to two equal and opposite axial pushes P (also called compressive load) as shown in Fig.(a), then the stress induced at any section of the body is known as compressive stress as shown in Fig.(b). A little consideration will show that due to the compressive load, there will be an increase in cross-sectional area and a decrease in length of the body. The ratio of the decrease in length to the original length is known as compressive strain

Let    P = Axial compressive force acting on the body,

A = Cross-sectional area of the body,

l = Original length, and

δl = Decrease in length.

Compressive stress, σc = P/A Compressive strain, εc = δl /l

 Young's Modulus or Modulus of Elasticity

 Hooke's law states that when a material is loaded within elastic limit, the stress is directly proportional to strain, i.e.

       ∝ ε

       = E.ε

 E = σ / ε

 = P l / (A×δ l)

 Where E is a constant of proportionality known as Young's modulus or modulus of elasticity. In S.I. units, it is usually expressed in GPa i.e. GN/m² or kN/mm². It may be noted that Hooke's law holds good for tension as well as compression.