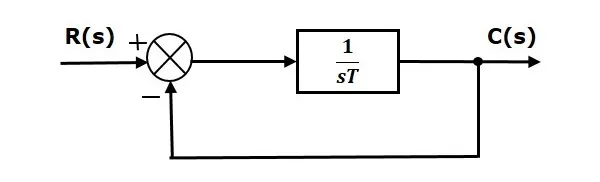
Response of the First Order System
let us discuss the time response of the first order system. Consider the following block diagram of the closed loop control system. Here, an open loop transfer function, 1sT1sT is connected with a unity negative feedback.

We know that the transfer function of the closed loop control system has unity negative feedback as,

The power of s is one in the denominator term. Hence, the above transfer function is of the first order and the system is said to be the first order system.
We can re-write the above equation as
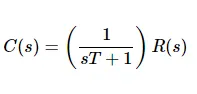
Where,
· C(s) is the Laplace transform of the output signal c(t),
· R(s) is the Laplace transform of the input signal r(t), and
· T is the time constant.
Follow these steps to get the response (output) of the first order system in the time domain.
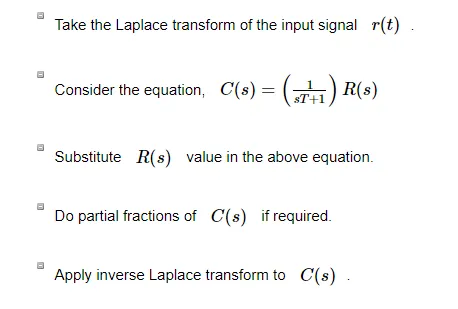
In the previous chapter, we have seen the standard test signals like impulse, step, ramp and parabolic. Let us now find out the responses of the first order system for each input, one by one. The name of the response is given as per the name of the input signal. For example, the response of the system for an impulse input is called as impulse response.